_S01_024_Q01.png)
Water and liquid is filled up behind a square wall of side l
Find out (a). Pressure at A, B and C
(b). Forces in part AB and BC
(c). Total force and point of application of force. (Neglect atmosphere pressure in every calculation.)
_S01_024_S01.png)
_S01_024_S02.png)
(a). As there is no liquid above 'A'
So pressure at A,pA=0
pressure at B,pB=ρgh1
Pressure at C,pC=ρgh1+2ρgh2
(b). Force at A=0
Take strip of width dx at a depth x in part AB
Pressure is equal to ρgx
force on strip=pressure×area
dF=ρgxldx
Total force upto B:Fh1∫0ρgxldx=ρgxlh212=1000×10×10×5×52=1.25×106N
In part BC for force take a elementary strip of width dx in portion BC.
pressure is equal to =ρgh1+2ρg(x−h1)
force on elementary strip=pressure×area dF=[ρgh1+2ρg(x−h1)]ldx
Total force on part BC F=∫lh1[ρgh1+2ρg(x−h1)]ldx
=[ρgh1x+2ρg[x22−h1x]]lh1l
=ρgh1h2l+2ρl[l2−h212−h1l+h21]
=ρgh1h2l+2ρgl2[l2+h21−2h1l]=ρgh1h2l+ρgl(l−h1)2
=ρgh2l[h1+h2]=ρgh2l2=1000×10×5×10×10=5×106N
(c). Total force
Taking torque about A
Total torque of force in AB=∫dF⋅x=∫h10ρgxldx.x
=[ρghlx33]h10=ρglh313=1000×10×10×1253=1.25×1073N−m
Total torque of force in BC =∫dF⋅x
On solving we get =ρgh1h2l[h1+h22]+ρgh22l[h1+2h23]
=1000×10×5×5×10[5+2.5]+1000×10×25×10[5+103]
=2.5×7.5×106+62.53×106=118.753×106
total torque =11.875×1073+1.25×1073=13.125×1073
total torque =total × distance of point of application of force from top =F.xp
6.25×106xp=13.125×1073
xp=7m
Topper's Solved these Questions
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBook:ALLENChapter:ELASTICITY, SURFACE TENSION AND FLUID MECHANICSExercise:Exercise 1 (Elasticity)
Explore 16 Videos
- ELASTICITY, SURFACE TENSION AND FLUID MECHANICSBook:ALLENChapter:ELASTICITY, SURFACE TENSION AND FLUID MECHANICSExercise:Exercise 1 (Surface Tension)
Explore 21 Videos
- CENTRE OF MASSBook:ALLENChapter:CENTRE OF MASSExercise:EXERCISE-V B
Explore 19 Videos
- ERROR AND MEASUREMENTBook:ALLENChapter:ERROR AND MEASUREMENTExercise:Part-2(Exercise-2)(B)
Explore 22 Videos
Similar Questions
Water and liquid are filled up behind a square wall of side. Find out
a. pressures at A,B and C
b. forces in part AB and BC
c. total force and point of application of force (neglect atmospheric pressure in every calculation)
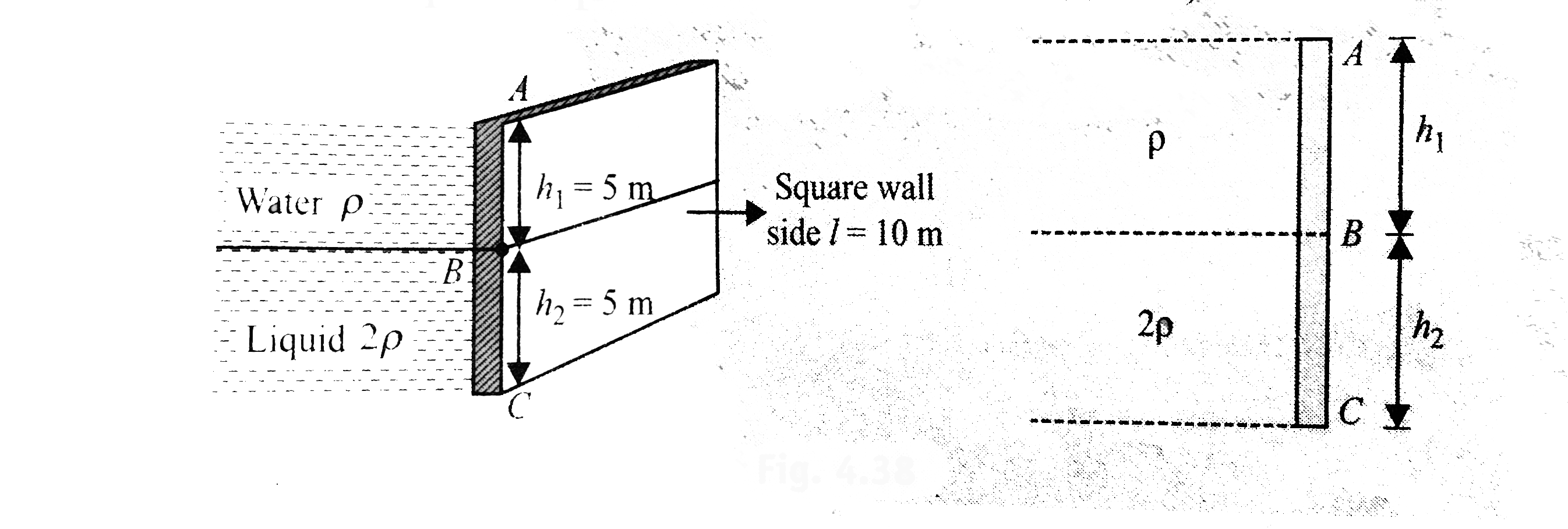
View SolutionHydrostatics: Pressure due to a Liquid Column | Equating pressure in U-tube Problems | Force and Torque on container wall (point of application of force)
View Solution
Knowledge Check
Mercury is completely filled in a rectangular take of height 72 cm. The atmospheric pressure at the place is 72 cm. of Hg. Find the distance in cm of point of application from bottom of tank of the net force , on the inner surface of the side vertical wall of tank.
A32 cmB16 cmC74 cmD42 cm
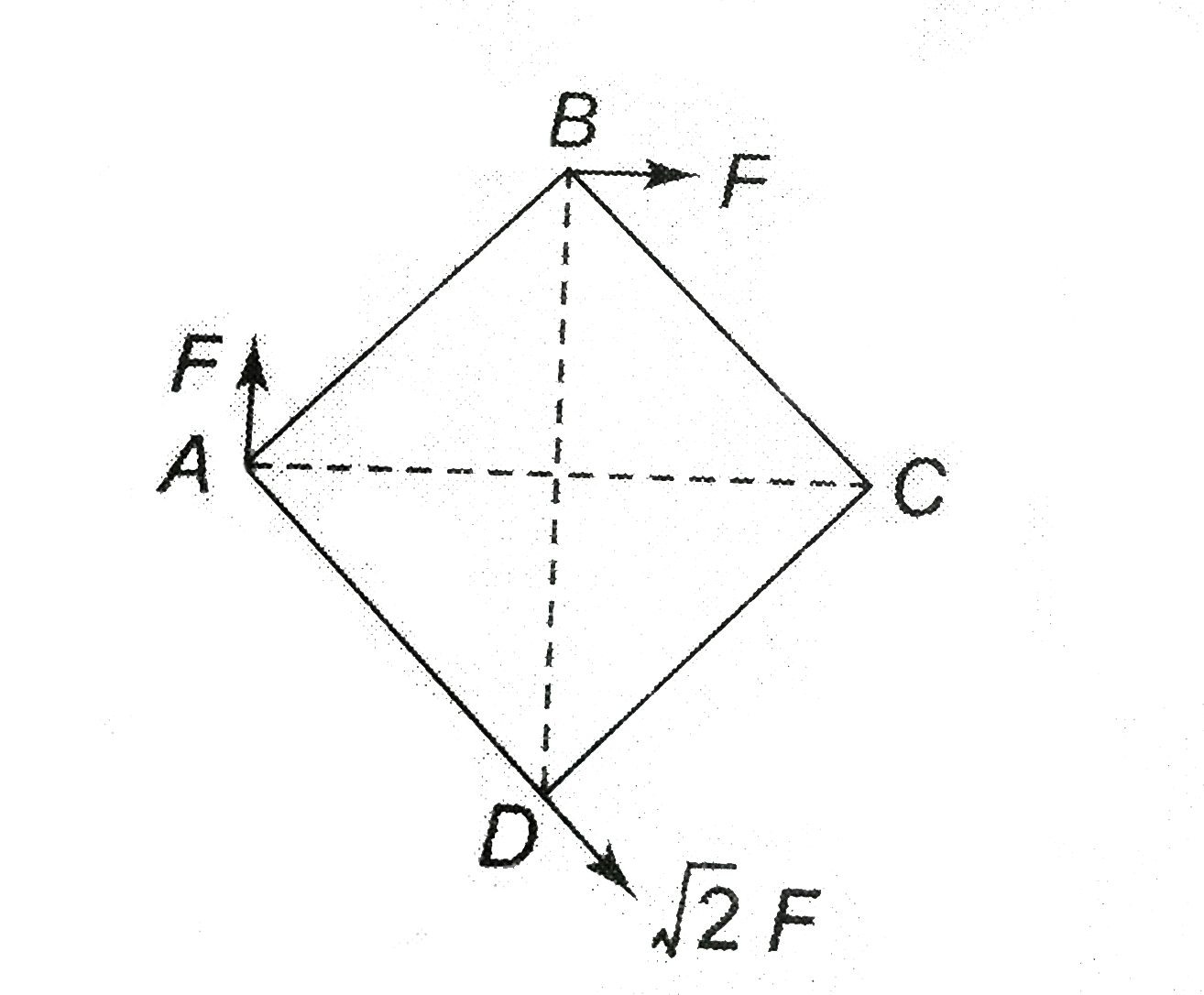
Three force are applied to a square plate as shown in the figure. Find the magnitude, direction and point of application of the resultant force if this point is taken on the side BC.
View Solution_S01_031_Q01.png)
In a given arrangement (a) find out velocity of water coming out of C
(b). Find out pressure A, B and C
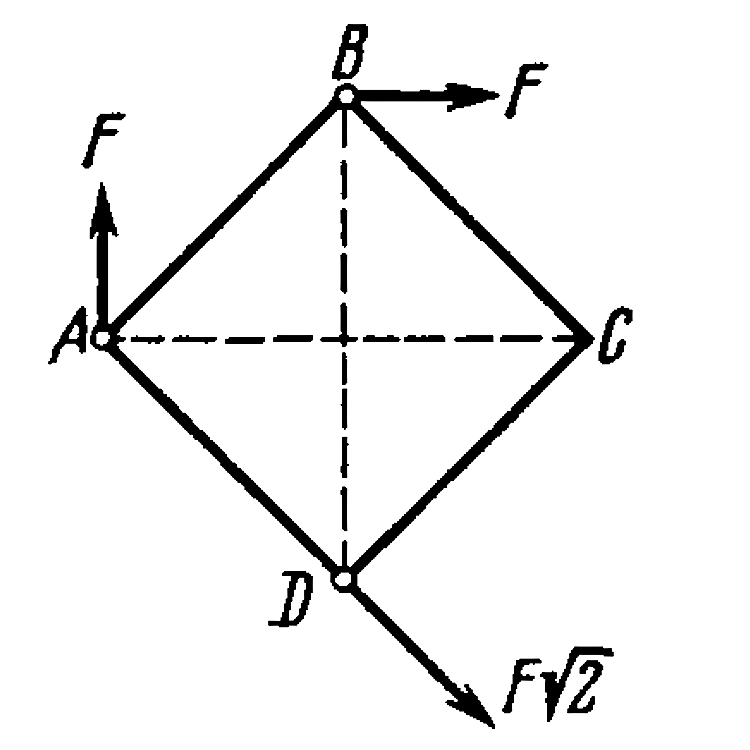
View SolutionThree forces are applied to a square plate as shown in Figure. Find the modulus, direction, and the point of application of the resultant force, if this point is taken on the side BC.
View SolutionTwo parallel unlike forces of magnitudes 15 N and 10 N are acting at point A and B respectively. If C is the point of action of resultant, then AB/BC is
View SolutionTwo parallel unlike forces of magnitudes 15 N and 10 N are acting at point A and B respectively. If C is the point of action of resultant, then AB/BC is
View SolutionTwo liquids of densities ρ1 and ρ2(ρ2=3ρ1) are filled up behind a square wall of side 6 m as shown in figure. Each liquid has a height of 3 m. The ratio of the forces due to these liquids exerted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing)2
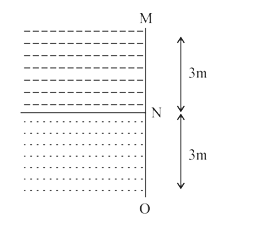
View Solution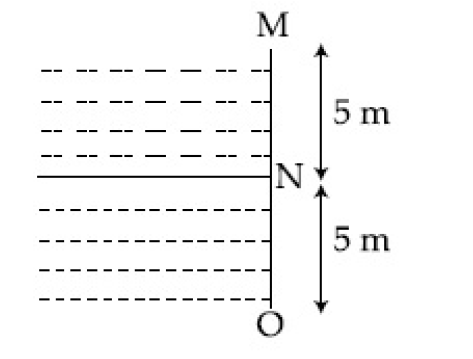 Tow liquids of desities ρ1 and ρ2(ρ2=2ρ1) are filled up behind a square wall of side 10 m as shown in the figure. Each liquids has a height of 5m. The ratio of the forces due to these liqyuids exeted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing):
Tow liquids of desities ρ1 and ρ2(ρ2=2ρ1) are filled up behind a square wall of side 10 m as shown in the figure. Each liquids has a height of 5m. The ratio of the forces due to these liqyuids exeted on upper part MN to that at the lower part NO is (Assume that the liquids are not mixing):
View Solution
