A light source of power 16 watts emits light of wavelength 310nm. If all emitted photons are made to strike a metal plate of work function 1.5eV then find out the magnetic of photo-current if 50% of incident photons eject photoelectrons.
To solve the problem step by step, we will follow these calculations:
Step 1: Calculate the energy of a single photon.
The energy of a photon can be calculated using the formula:
E=hcλ
where:
- h (Planck's constant) = 6.626×10−34J s
- c (speed of light) = 3×108m/s
- λ (wavelength) = 310nm=310×10−9m
Substituting the values:
E=(6.626×10−34J s)×(3×108m/s)310×10−9m
Calculating this gives:
E≈6.39×10−19J
Step 2: Convert the energy of a photon from joules to electron volts.
To convert joules to electron volts, we use the conversion factor 1eV=1.6×10−19J:
E≈6.39×10−19J1.6×10−19J/eV≈3.99eV
Step 3: Calculate the number of photons emitted per second.
The number of photons emitted per second can be calculated using the formula:
Number of photons=PowerEnergy of one photon
Given the power of the light source is 16W:
Number of photons=16W6.39×10−19J≈2.5×1019photons/s
Step 4: Calculate the number of photoelectrons emitted.
Since only 50% of the incident photons eject photoelectrons:
Number of photoelectrons=0.5×2.5×1019≈1.25×1019photoelectrons/s
Step 5: Calculate the photo current.
The photo current can be calculated using the formula:
I=Number of photoelectrons×e
where e (the charge of an electron) is approximately 1.6×10−19C:
I=1.25×1019photoelectrons/s×1.6×10−19C≈2A
Final Answer:
The magnitude of the photo current is approximately 2A.
---
The energy of a photon can be calculated using the formula:
E=hcλ
where:
- h (Planck's constant) = 6.626×10−34J s
- c (speed of light) = 3×108m/s
- λ (wavelength) = 310nm=310×10−9m
E=(6.626×10−34J s)×(3×108m/s)310×10−9m
Calculating this gives:
E≈6.39×10−19J
To convert joules to electron volts, we use the conversion factor 1eV=1.6×10−19J:
E≈6.39×10−19J1.6×10−19J/eV≈3.99eV
The number of photons emitted per second can be calculated using the formula:
Number of photons=PowerEnergy of one photon
Given the power of the light source is 16W:
Number of photons=16W6.39×10−19J≈2.5×1019photons/s
Since only 50% of the incident photons eject photoelectrons:
Number of photoelectrons=0.5×2.5×1019≈1.25×1019photoelectrons/s
The photo current can be calculated using the formula:
I=Number of photoelectrons×e
where e (the charge of an electron) is approximately 1.6×10−19C:
I=1.25×1019photoelectrons/s×1.6×10−19C≈2A
The magnitude of the photo current is approximately 2A.
Topper's Solved these Questions
Similar Questions
A cylindrical source of light which emits radiation radially (from curved surface) only , placed at the centre of hollow, metallic cylindrical surface, as shown in diagram.
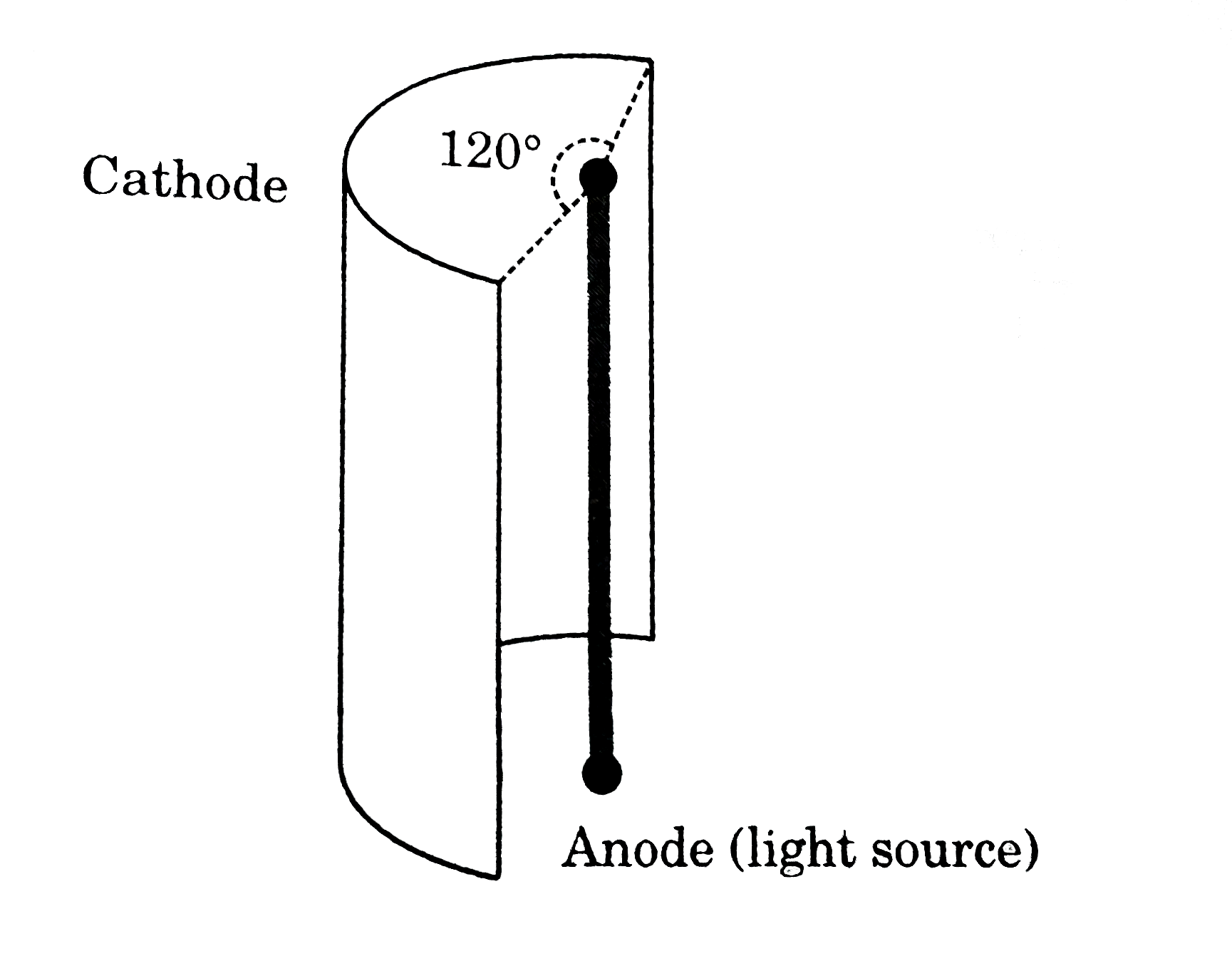
The power of source is 90 watt and it emits light of wavelength 4000Å only . The emitted photons strike the metallic cylindrical surface which results in ejection of photoelectrons. All ejected photoelectrons reaches to anode (light source). The magnitude of photocurrent (in amp) is :
[Given:h=6.4×10−34J/sec]
View SolutionThe energy of emitted photoelectrons from a metal is 0.9ev, The work function of the metal is 2.2eV. Then the energy of the incident photon is
View Solution
Knowledge Check
If Photon having wavelength 6.2nmwas allowed to strike a metal plate having work function 50eV then calculate wavelength associated with emitted electron :
A1×10−10mB2×10−10mC3×10−18mD4×10−34mLight of wavelength 5000Å falls on a sensitive plate with photoelectric work function of 1.9eV. The kinetic energy of the photoelectron emitted will be
A0.58eVB2.48eVC1.24eVD1.16eVLight of wavelength 5000Å falls on a sensitive plate with photoelectric work function of 1.9eV. The kinetic energy of the photoelectron emitted will be
A0.58 eVB2.48 eVC1.24 eVD1.16 eV
Ligth of wavelength 4000Å is incident on a metal plate whose work function is 2eV. What is maximum kinetic enegy of emitted photoelectron ?
View SolutionThe wavelength of the electron emitted by a metal sheet of work function 5eV when photons from EMR of wavelength 62 nm strike the metal plate
View SolutionLight of wavelength 3000Å is incident on a metal surface whose work function is 1 eV. The maximum velocity of emitted photoelectron will be
View SolutionLight of wavelength 500nm is incident on a metal with work function 2.28eV. The de Broglie wavelength of the emitted electron is
View SolutionRadiation of wavelength 310 nm is incidenton a metal surface of work function 1eV. Find maximum speed of photoelectrons emitted . ( Closed value )
View Solution
