The coefficient of mutual inductance
μ0a2π1n(1+a2b)
μ0aπ1n(1+b2a)
μ0a2π1n(1+ab)
μ0a2π1n(1+ba)
The correct Answer is:C
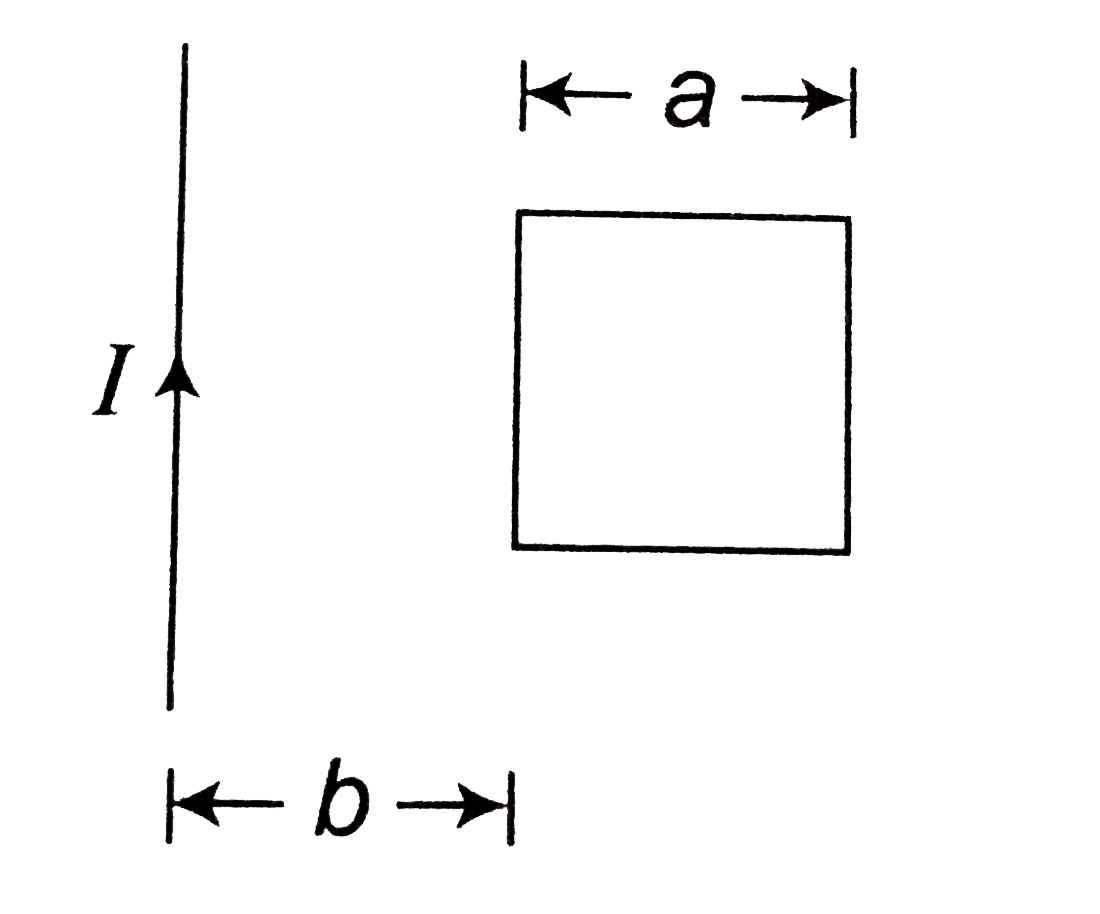
Magnetic field due to wire
B=μ0i2πx,dA=adx
dϕ=BdA
ϕ=μ0ia2π∫b+abdxx=μ0ia2π1n(1+ab)=Mi
M=μ0a2π1n(1+ab)
Topper's Solved these Questions
Similar Questions
Two circular coils are kept in vicinity of each other. The current in primary coil changes from 10 A to 6 A in 0.3 sec. Calculate the coefficient of mutual inductance of the pair of emf of 1V induced in secondary coil.
View SolutionA current of 0.5 A in the primary coil of a transfomer is reduced to zero at a uniform rate in 0.1 second. If the coefficient of mutual inductance is 3H, what is the emf induced in the secondary coil and charge in the magnetic flux per turn in the secondary coil if it has 50 turns?
View Solution
Knowledge Check
Alternating current of peak value (2π) ampere flows through the primary coil of the transformer. The coefficient of mutual inductance between primary and secondary coil is 1 henry. The peak e.m.f. induced in secondary coil is (Frequency of a.c.=50Hz)
A100VB200VC300VD400VAlternating current of peak value (2π) ampere flows through the primary coil of the transformer. The coefficient of mutual inductance between primary and secondary coil is 1 henry. The peak e.m.f. induced in secondary coil is (Frequency of AC= 50 Hz)
A100 VB200 VC300 VD400 VA current of 10A in the primary coil of a circuit is reduced to zero. If the coefficient of mutual inductance is 3 H and emf induced in the secondary coil is 30 kV, time taken for the change of current is
A103sB102sC10−3sD10−2s
A small square loop of side 'a' and one turn is placed inside a larger square loop of side b and one turn (b>>a). The two loops are coplanar with their centres coinciding. If a current I is passed in the square loop of side 'b', then the coefficient of mutual inductance between the two loops is :
View SolutionA 100 cm long solenoid having diameter 2 cm and 1000 turns has a primary solenoid closely wound near its mid point. If the coefficient of mutual inductance between the coils is 0.002 H, then find the number of turns in secondary coil.
View SolutionWhat are the factors on which coefficient of mutual inductance of two coils depends?
View SolutionIn a transformer , the coefficient of mutual inductance between the primary and the secondary coil is 0.2 henry. When the current changes by 5 ampere//second in the primary, the induced e.m.f. in the secondary will be
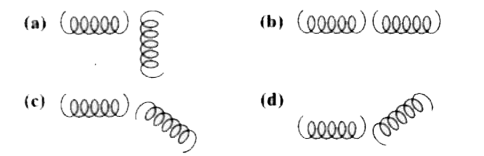
View SolutionFor which arrangement of two coils, shown in the below figure, coefficient of mutual inductance is maximum and for which it is minimum?
View Solution
