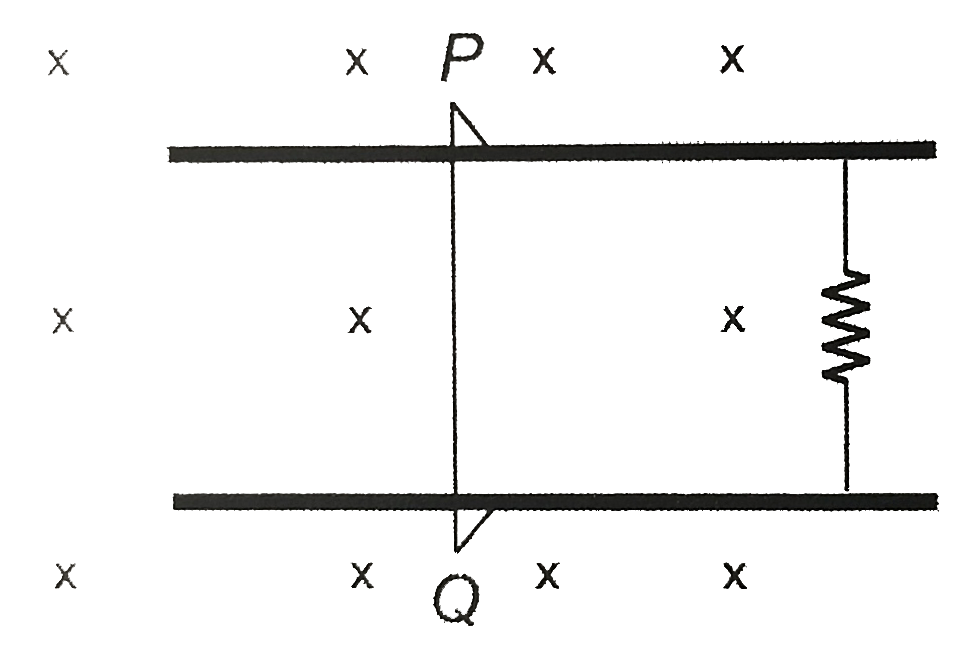
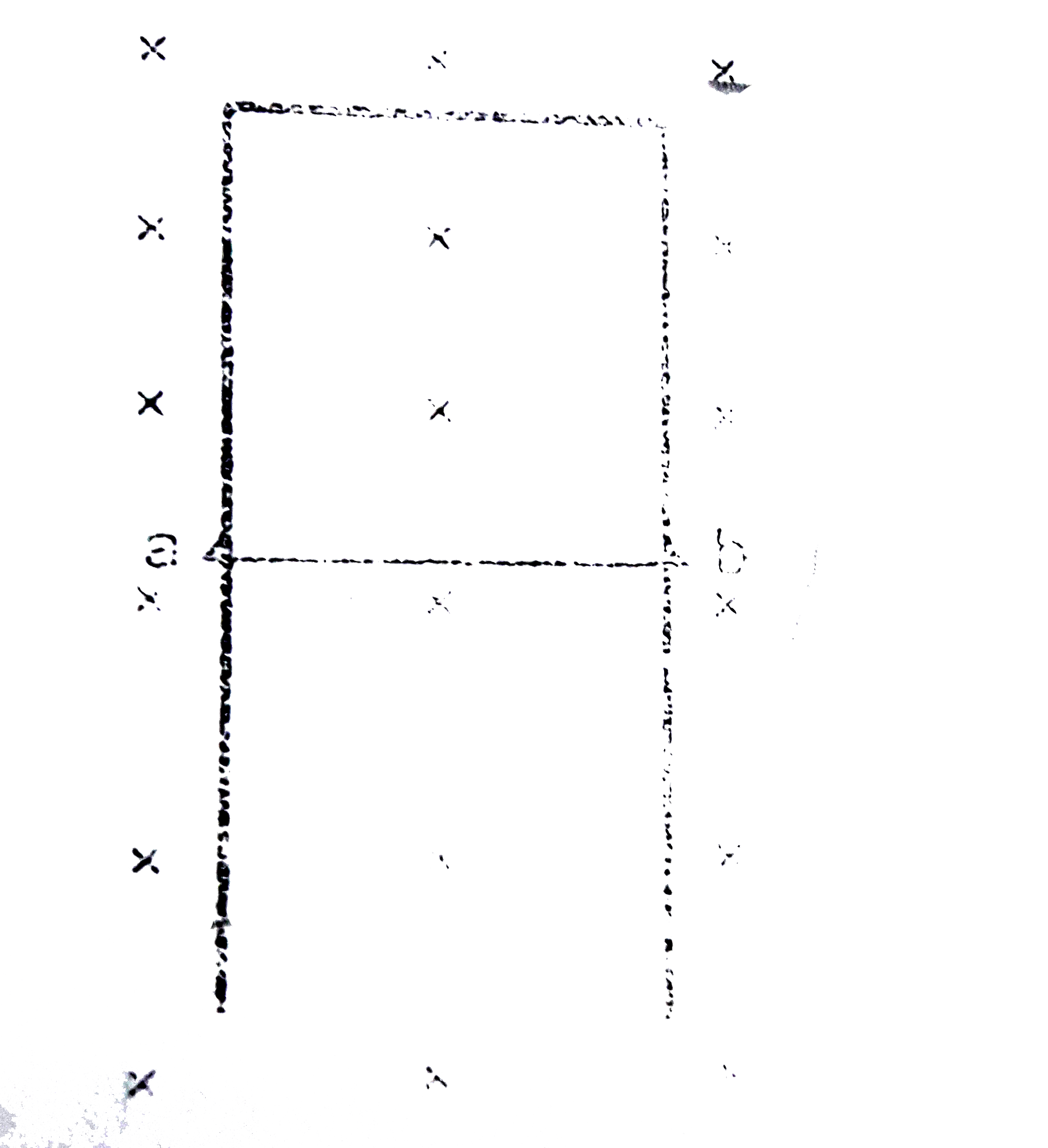
Consider the situation shown in the figure. The wire PQ has mass m , resistance r and can slide 0n the smooth, horizontal parallel rails separated by a distance l . The resistance of the rais is negiligible. A uniform magnetic field B exists in the reactangular region and a resistance R connects the rail outside the field region. At t=0 , the wire PQ is pushed toward right with a speed v0 . Find
(a) the current in the loop at an instant when the speed of the wire PQ is v
(b) the acceleration of the wire as this instant
(c) the velocity v as a function of x
(d) the maximum distance the wire will move
(e) the velocity as a function of time
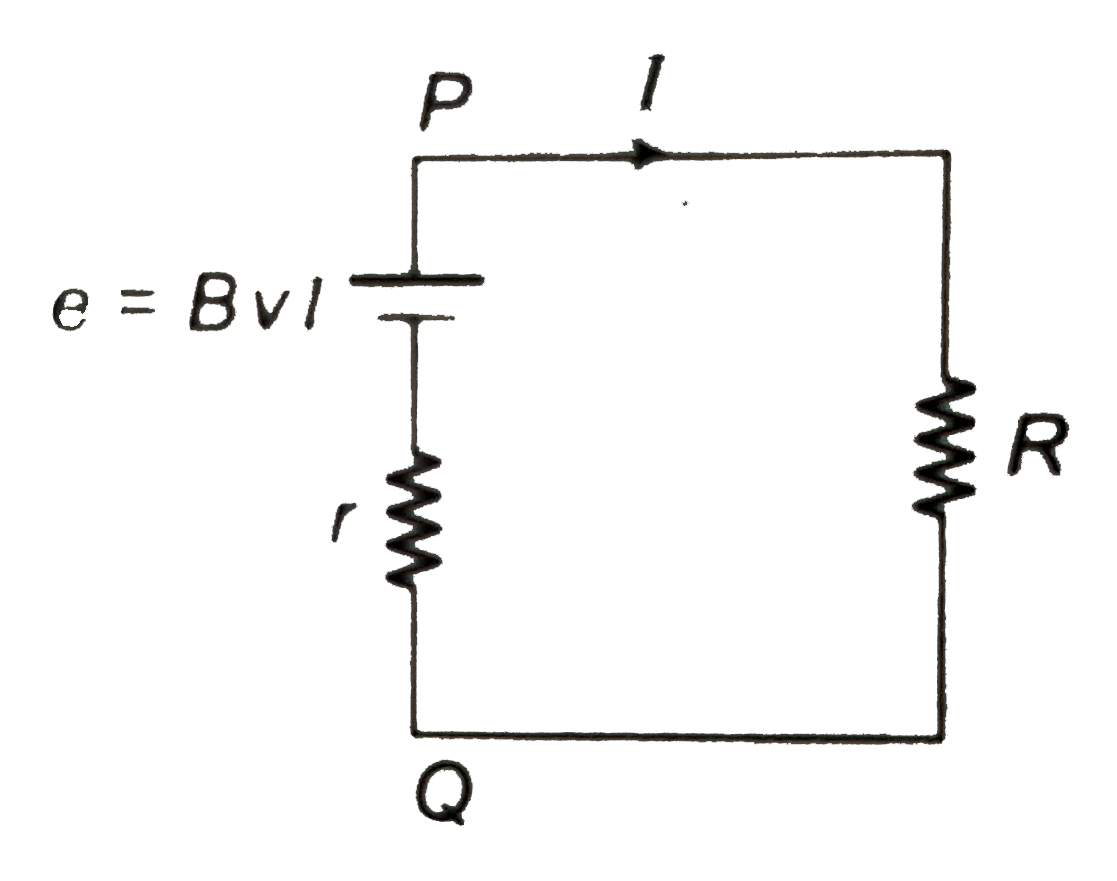
When speed of wire PQ is v , it can be replaced by a battery of emf e=Bvl
(a)I=eR+r=BvlR+r
Force on wire PQ ,
F=Bil=B2vl2R+r , towards left
(b) Retardation of wire a=B2l2m(R+r)
(c) a=B2l2m(R+r)v
vdvdx=−B2l2m(R+r)v
∫vv0dv=−B2l2m(R+r)∫x0dx
|v|vv0=−B2l2m(R+r)|x|x0
v−v0=−B2l2m(R+r)x
v−v0=−B2l2m(R+r)
(d) The wire will stop, when v=0
x0=mv0(R+r)B2l2
(e) a=B2l2m(R+r)v=λv
where λ=B2l2m(R+r)
dvdt=−λv
∫vv0dvv=−λ∫t0dt
|1nv|vvo=−λt
1nv−1nv0=−λt
1n(v/v0)=−λt
vv0=e−λt
v=v0e−λt


Topper's Solved these Questions
Similar Questions
A rod of mass m, length l and resistance R is sliding down on a smooth inclined parallel rails with a cinstant velocity v. If a uniform horizontal magnetic field B exists, then find thevalue of B.
View SolutionConsider the situation shown in figure.The wire CD has a negligible resistance and is made to slide on the three rails with a constant speed of 50cm/s.Find the current in the 10Ω resistor when the switch S is thrown to (a) the middle rail (b)bottom rails.(Neglect resistance of rails)

View Solution
Knowledge Check
A wire ab of length l, mass m and resistance R slides on a smooth thick pair of metallic rails joined at the bottom as shown in fig. The plane of the rails makes an angle θ with the horizontal. A vertical magnetic field B exist in the region. If the wire slides on the rails at a constant speed v, then the value of B is -
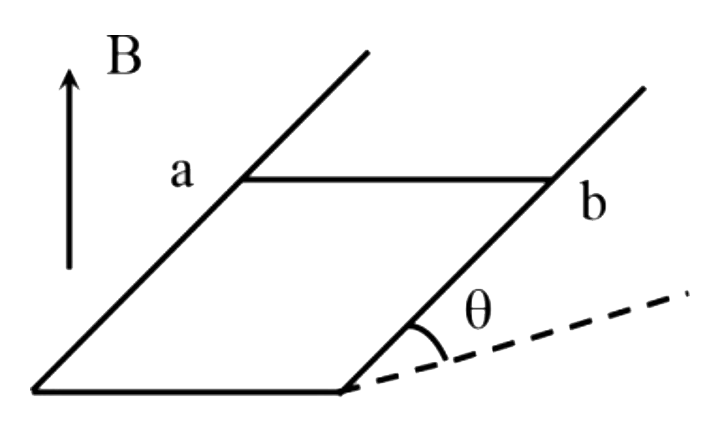 A√mgRvl2cos2θB√mgRcosθvl2sin2θC√mgRv2l2sin2θD√mgRsinθvl2cos2θ
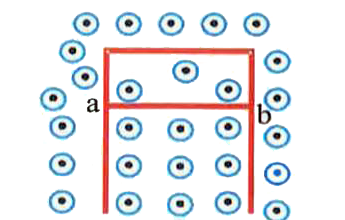
A√mgRvl2cos2θB√mgRcosθvl2sin2θC√mgRv2l2sin2θD√mgRsinθvl2cos2θA copper wire ab of length l, resistance r and mass m starts sliding at t=0 down a smooth, vertical, thick pair of connected condcuting rails as shown in figure.A uniform magnetic field B exists in the space in a direction perpendicular to the plane of the rails which options are correct.
 AThe magnitude and direction of the induced current in the wire when speed of the wire v is vBlr, a to bBThe downward acceleration of the wire at this instant g−B2l2mrv.CThe velocity of the wire as a function of time vm(1−e−gt/vm)(where vm=mgrB2l2)DThe displacement of the wire as a function of time vmt−v2mg(1−e−gt/vm)(where vm=mgrB2l2)
AThe magnitude and direction of the induced current in the wire when speed of the wire v is vBlr, a to bBThe downward acceleration of the wire at this instant g−B2l2mrv.CThe velocity of the wire as a function of time vm(1−e−gt/vm)(where vm=mgrB2l2)DThe displacement of the wire as a function of time vmt−v2mg(1−e−gt/vm)(where vm=mgrB2l2)A copper wire ab of length l, resistance r and mass m start sliding at t=0 down a smooth, vertical, thick pair of connected conducting rails as shown in figure. A uniform magnetic field B exists in the space in a direction perpendicular to the plane of the rails which options are correct .
 AThe magnitude and direction of the induced current in the wire when speed of the wire v is vBlr, a to bBThe downward acceleration of the wire at this instant g−B2l2mrvCThe velocity of the wire as a function of time vm(1−e−gt/vm),(wherevm=mgrB2l2)DThe displacement of the wire as a function of time vmt−v2mg(1−e−gt/vm),(wherevm=mgrB2l2)
AThe magnitude and direction of the induced current in the wire when speed of the wire v is vBlr, a to bBThe downward acceleration of the wire at this instant g−B2l2mrvCThe velocity of the wire as a function of time vm(1−e−gt/vm),(wherevm=mgrB2l2)DThe displacement of the wire as a function of time vmt−v2mg(1−e−gt/vm),(wherevm=mgrB2l2)
In a metal wire of mass m=24.1 mg can slide with negligible friction on two horizontal parallel rails separated by distance d=2.56 cm . The track lies in a vertical uniform magnetic field of magnitude 56.3 m. At time t=0 , device G is connected to the rails . producing a constant current i=9.13 mA in the wire and rails (even as the wire moves). At t=61.1 ms , what are the wire's (a) speed and (b) direction of motion (left or right)?

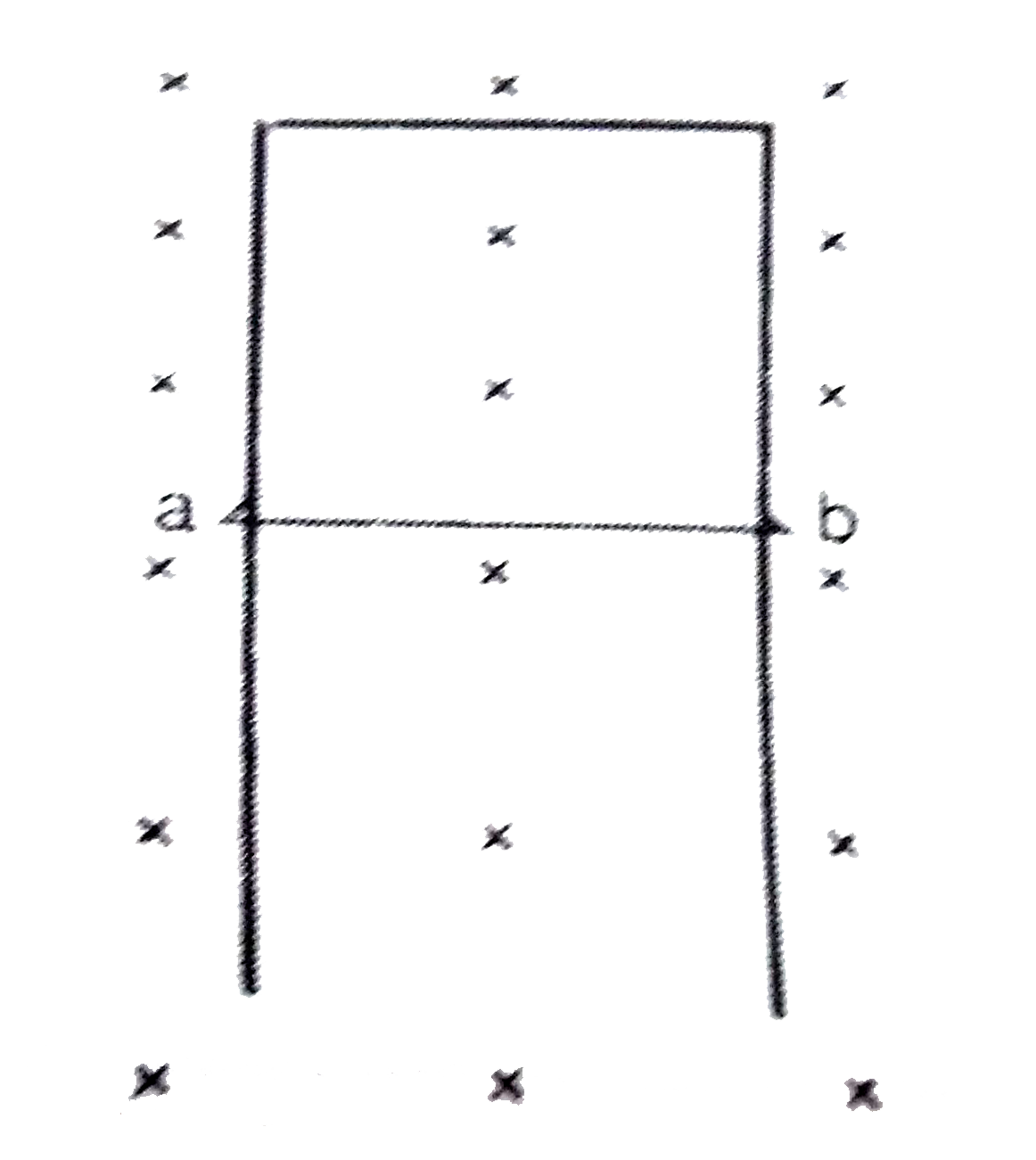
View SolutionA constant force is being applied on a road of length 'l' kept at rest on two parallel conducting rails connected at ends by resistance R in uniform magnitic field B shown.
View SolutionA conducting wire ab of length l resistance r and mass m starts sliding down at t=0 on a smooth, vertical thick pair of connected rails as shown. The terminal speed of the wire is
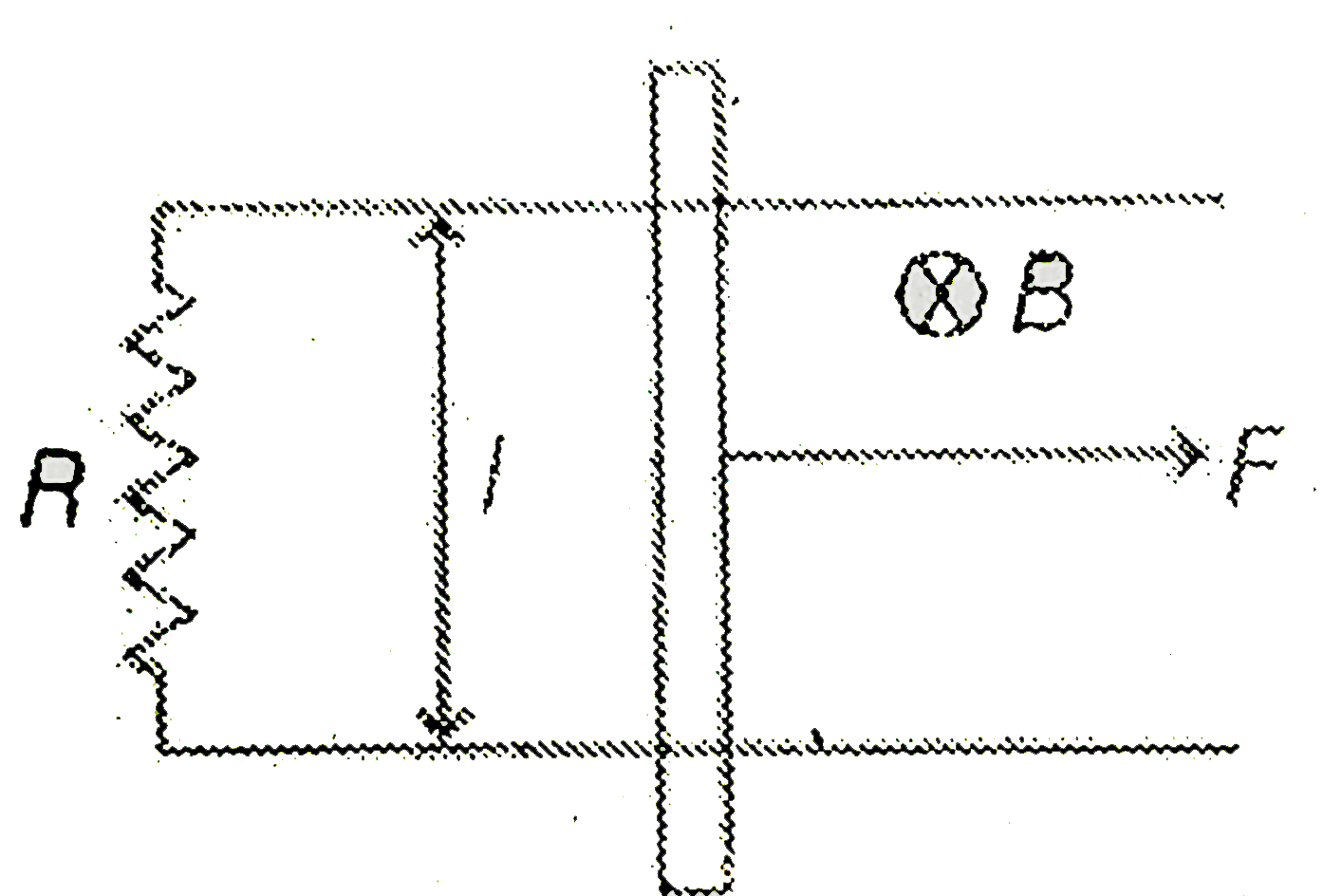
View SolutionA horizontal wire is free to slide on the vertical rails of a conducting frame as shown in figure. The wire has a mass m and length l and the resistance of the circuit is R. If a uniform magnetic field B is directed perpendicular to the frame, the terminal speed of the wire as it falls under the force of gravity is

View SolutionA conducting wire of length l and mass m can slide without friction on two parallel rails and is connected to capacitance C. The whole system lies in a magnetic field B and a constant force F is applied to the rod. Then
View Solution
