A long solenoid of radius 3cm , length I00cm carries a current of 4A . The total number of truns is 300 . Assuming ideal solenoid, find the flux passing through a circular surface having centre on axis of solenoid,
(a) of radius 2cm and is perprndicular of the axis of solenoid (i) inside and (ii) at the end of solenoid
(b) of radius 4cm and is perprndicular of the axis of solenoid
(c) of radius greater than 3cm and angle between normal to area and axis of solenoid is 60∘C
n=Nl=300I=300turns/m
(a) B=μ0ni , A=πr2I , θ=0∘
ϕ=Bacosθ=μ0ni.πr2Icos0
=4π×I0−7×300×4×π×(2×I0−2)2
=I.92×I0−6 where =I.92μWb
At the end : B:I2μ0ni
π=I.922=0.92μWb
(b) The magnetic field exists only in radius 3cm
Aπr2
ϕ=I.92×π(3)2π(2)2=4.32μWb
(c) θ=60∘ , ϕ=4.32cos60∘=2.I6Wb
θ=90∘ , ϕ=0
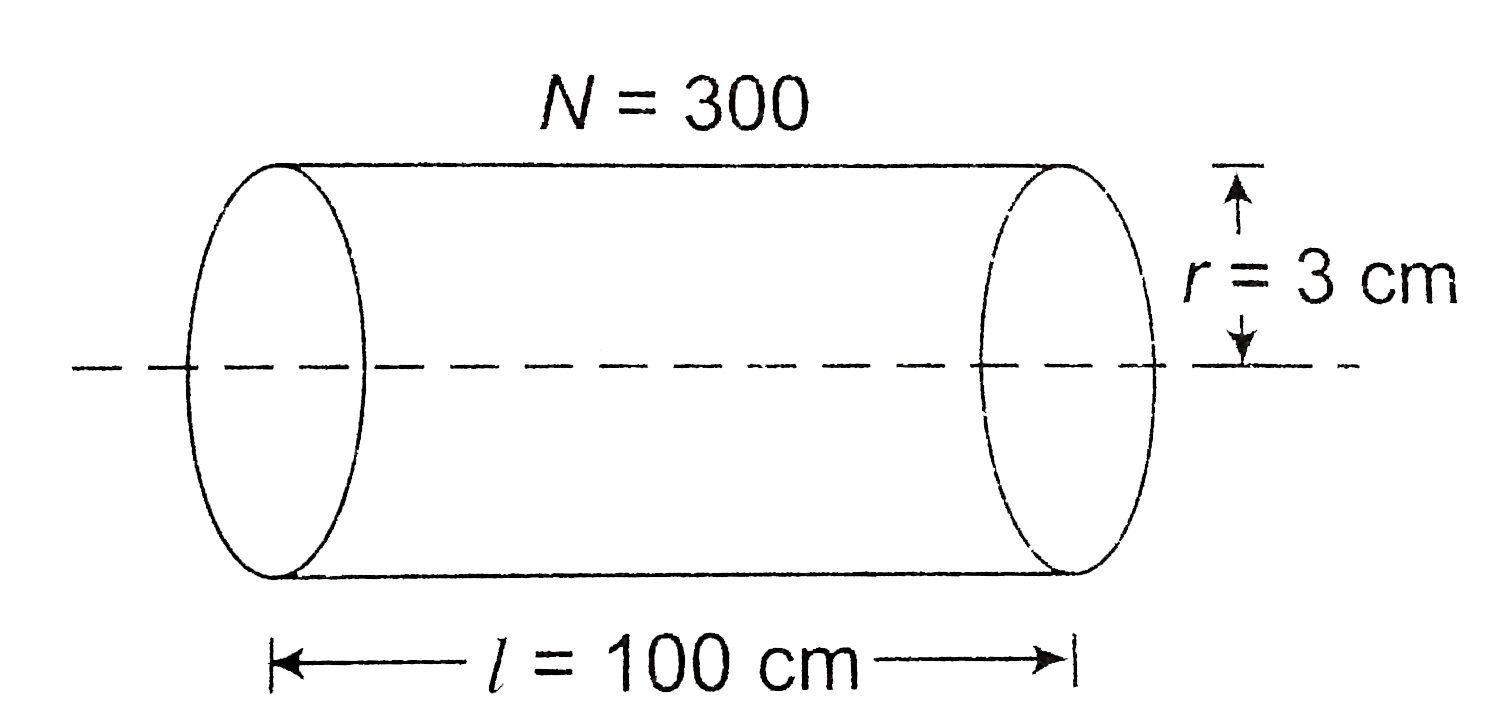
Topper's Solved these Questions
Similar Questions
A long solenoid of radius 4 cm, length 400 cmcarries a current of 3 A. The total number of turns is 100. Assuming ideal solenoid, find the flux passing through a circular surface having centre on axis of solenoid of radius 3 cm and is perpendicular to the axis of solenoid (i) inside and (ii) at the end of solenoid.
View SolutionA long solenoid with radius 2cm carries a current of 2A. The solenoid is 70cm long and is composed of 300 turns of wire. Assuming ideal solenoid model, calculate the flux linked with a circular surface if
(a) it has a radius of 1cm and is perpendicular to the axis of the solenoid:
(i) inside,
(ii) outside.
(b)it has a radius of 3cm and is perpendicular to the axis of the solenoid with its center lying on the axis of the solenoid.
( c ) it has a radius greater than 2cm and axis of the solenoid subtend an angle of 60∘ with the normal to the area (the center of the circular surface being on the axis of the solenoid).
(d) the plane of the circular area is parallel to the axis of the solenoid.
View Solution
Knowledge Check
A solenoid of length 0.5 m has a radius of 1 cm and is made up of 500 turns. It carries a current of 5 A. The magnetic field inside the solenoid is
A3.14×10−3TB6.28×10−3TC9.14×10−3TD1.68×10−3TA long solenoid of 50 cm length having 100 turns carries a current of 2.5A. The magnetic field at centre of solenoid is:
A3.14×10−4 TB6.28×10−5 TC3.14×10−5 TD6.28×10−4 T
A long solenoid of 50 cm length having 100 turns carries a current of 2.5A. The magnetic field at centre of solenoid is:
View SolutionFind the length of a solenoid of radius 2 cm and 800 turns per metre having self-inductance of 0.115 H.
View SolutionCalculate the self-inductance of an air-cored solenoid 50 cm long and 2 cm radius if the number of turns in the coil of solenoid is 400.
View SolutionA long solenoid of length 'l' having carries a current I. Deduce the expression for the magnetic field in the interior of the solenoid.
View SolutionA solenoid having 500 turns and length 2m has radius of 2 cm. Then self-inductance (in milithenry) of solenoid is
View SolutionA solenoid of length 0.4m and having 500 turns of wire carries a current of 3amp.Calculate the torque requred to hold a coil (having radius 0.02cm current 2A and turns 50)in the middle of the solenoid with its axis perpendicular to the axis of the solenoid.(π2=10)
View Solution
