A hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
Thickness of hemispherical bowl is 0.25cm and inner radius, r=5cm
Outer radius, R=r+thickness
R=5+0.25 = 5.25cm
∴ Outer C.S.A of hemispherical bowl = 2πR2
Outer C.S.A of hemispherical bowl = 2×227×5.252 = 173.25cm2
Hence, Outer C.S.A of hemispherical bowl is 173.25cm2.
Topper's Solved these Questions
- SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEBOOK - RD SHARMACHAPTER - SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEEXERCISE - All Questions
128 Videos
- TABULAR REPRESENTATION OF STATISTICAL DATA BOOK - RD SHARMACHAPTER - TABULAR REPRESENTATION OF STATISTICAL DATA EXERCISE - All Questions
62 Videos
Similar Questions
Knowledge Check
The coefficient of friction between a hemispherical bowl and an insect is √0.44 and the radius of the bowl is 0.6m. The maximum height to which an insect can crawl in the bowl will be
A0.4mB0.2mC0.3mD0.1mThe coefficient of friction between a hemispherical bowl and an insect is √0.44 and the radius of the bowl is 0.6m. The maximum height to which an insect can crawl in the bowl will be .
A0.4mB0.2mC0.3mD0.1m
A hemispherical bowl is made of steel, 0.5 cm thick. The inside radius of the bowl is 4 cm. Find the volume of steel used in making the bowl.
View Solutiona hemispherical bowl is made of steel 0.5 cm thick. The inside radius of the bowl is 4 cm. Find the volume of steel used in making the bowl.
View SolutionThe radius of a hemisphere is 2.5 cm. Find its total surface area.
View SolutionA hollow hemispherical bowl of thickness 1 cm has an inner radius of 6 cm. Find the volume of metal required to make the bowl.
View SolutionA metallic pipe is 0.7 cm thick. Inner radius of the pipe is 3.5 cm and length is 5 dm. Find its total surface area.
View SolutionA stick is placed inside a hemispherical bowl as shown in Figure. The stick is horizontal and has a length of 2a. Eye of an observer is located at E such that it can just see the end A of the stick. A liquid is filled upto edge of the bowl and the end B of the stick becomes visible to the observer. Radius of the bowl is R. Find the refractive index (μ) of the liquid.
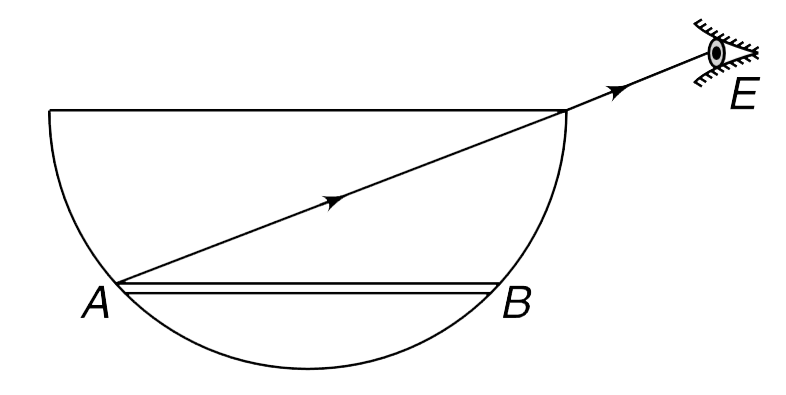
View Solution