For the thermal decomposition of acetaldehyde, CH3CHO(g)→CH4(g)+CO(g), the following rate data were obtained :
ExperimentInitial pressure (torr)Initial rate of increase in total pressure (torr) 1 300 0.61(r1) 2 200 0.27(r2)
Predict the order of reaction.
The correct Answer is:2
To determine the order of the reaction for the thermal decomposition of acetaldehyde, we will use the provided rate data from two experiments.
1. Identify the Rate Law:
The rate of a reaction can generally be expressed as:
Rate=k(A)n
where k is the rate constant, (A) is the concentration (or pressure in this case) of the reactant, and n is the order of the reaction.
2. Set Up the Ratios:
From the data provided:
- Experiment 1: Initial pressure P1=300 torr, Rate R1=0.61
- Experiment 2: Initial pressure P2=200 torr, Rate R2=0.27
We can write the rate expressions for both experiments:
R1=k(P1)nandR2=k(P2)n
3. Form the Ratio of Rates:
Taking the ratio of the rates from both experiments gives:
R1R2=k(P1)nk(P2)n
The k cancels out:
R1R2=(P1)n(P2)n
4. Substitute the Values:
Substitute R1=0.61, R2=0.27, P1=300, and P2=200:
0.610.27=(300)n(200)n
5. Simplify the Equation:
This simplifies to:
0.610.27=(300200)n
0.610.27=(32)n
6. Calculate the Left Side:
Calculate 0.610.27:
0.610.27≈2.26
7. Set Up the Equation:
Now we have:
2.26=(32)n
8. Take Logarithms:
To solve for n, take the logarithm of both sides:
log(2.26)=nlog(32)
9. Calculate n:
Rearranging gives:
n=log(2.26)log(32)
Using a calculator:
n≈0.3540.176≈2.01
10. Conclusion:
Since n≈2, we conclude that the order of the reaction is 2.
The rate of a reaction can generally be expressed as:
Rate=k(A)n
where k is the rate constant, (A) is the concentration (or pressure in this case) of the reactant, and n is the order of the reaction.
From the data provided:
- Experiment 1: Initial pressure P1=300 torr, Rate R1=0.61
- Experiment 2: Initial pressure P2=200 torr, Rate R2=0.27
R1=k(P1)nandR2=k(P2)n
Taking the ratio of the rates from both experiments gives:
R1R2=k(P1)nk(P2)n
The k cancels out:
R1R2=(P1)n(P2)n
Substitute R1=0.61, R2=0.27, P1=300, and P2=200:
0.610.27=(300)n(200)n
This simplifies to:
0.610.27=(300200)n
0.610.27=(32)n
Calculate 0.610.27:
0.610.27≈2.26
Now we have:
2.26=(32)n
To solve for n, take the logarithm of both sides:
log(2.26)=nlog(32)
Rearranging gives:
n=log(2.26)log(32)
Using a calculator:
n≈0.3540.176≈2.01
Since n≈2, we conclude that the order of the reaction is 2.
Topper's Solved these Questions
- CHEMICAL KINETICSBook:PRADEEPChapter:CHEMICAL KINETICSExercise:CURIOSITY QUESTION
Explore 2 Videos
- CHEMICAL KINETICSBook:PRADEEPChapter:CHEMICAL KINETICSExercise:TEST YOUR GRIP (MULTIPLE CHOICE QUESTIONS)
Explore 25 Videos
- CHEMICAL KINETICSBook:PRADEEPChapter:CHEMICAL KINETICSExercise:ADVANCED PROBLEMS FOR COMPETITIONS
Explore 14 Videos
- BIOMOLECULES Book:PRADEEPChapter:BIOMOLECULES Exercise:IMPORTANT QUESTIONS (FOR BOARD EXAMINATION)
Explore 25 Videos
- CHEMISTRY IN EVERYDAY LIFE Book:PRADEEPChapter:CHEMISTRY IN EVERYDAY LIFE Exercise:IMPORTANT QUESTION FOR BOARD EXAMINATION
Explore 30 Videos
Similar Questions
The following initial rate data were obtained at 300K for the reactions:
2A+B→C+D

Deducethe rate law.
View SolutionFor the first order thermal decomposition reaction, the following data were obtained:
C2H5Cl(g)→C2H4(g)+HCl(g)
Time/secondTotal pressure/atm 0 0.30 300 0.50
Calculate the rate constant. [Given : log 2 = 0.301, log 3 = 0.4771, log 4 = 0.6021]
View Solution
Knowledge Check
For the first order gas phase decomposition reaction,
A(g)→B(g)+C(g)
if P0 is the initial pressure of A and Pt is total pressure after time t, thenAk=2.303tlog(P0)PtBk=2.303tlog(P0)Pt−P0Ck=2.303tlog(P0)Pt−2P0Dk=2.303tlog(P0)2P0−PtFor the reaction 2NO(g)+H2(g)→N2O(g)+H2O(g), at 900K following data are observed.
Initial pressure of NO (atm)Initial pressure ofH2o(atm)Initial rate of pressure decrease(atm−1min)0.1500.400.0200.0750.400.0050.1500.200.010
Find the order of reaction.A3B2C1D0Conisder the reaction represented by the equation:
CH3Cl(g)+H2O(g)→CH3OH(g)+HCl(g)
These kinetics data were obtained for the given reaction concentrations:
Initial conc (M)Initial rate of disappearance[CH3Cl][H2O]of CH3Cl(Ms−1)0.20.210.40.220.40.48
If H2O is taken in large excess, the order of the reaction will beA1B0C3D2
For a zero order chemical reaction,
2NH3(g)→N2(g)+3H2(g)
rate of reaction=0.1atm/sec.Initially only NH3 is present and its pressure =3atm . Claculate total pressure at t=10sec.
View SolutionThe following data were obtained during the first order thermal
decomposition of SO2Cl2 at a constant volume SO2Cl2(g)→SO2(g)+Cl2(g)
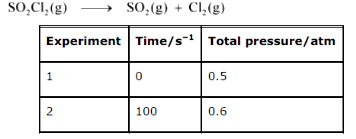
Calculate the rate of the reaction when total pressure is 0.65 atm
View SolutionFor the first order thermal decomposition reaction, the following data were obtained :
C2H5Cl(g)→C2H4(g)+HCl(g)
Time/Sec Total pressure/atm0 0.30300 0.50
Calculate the rate constant
(Given : log 2= 0.301, log 3 = 0.4771, log 4=0.6021)
View SolutionThe following initial rate data were obtianed for the reaction
2NOg+Br(g)→2NOBr(g)
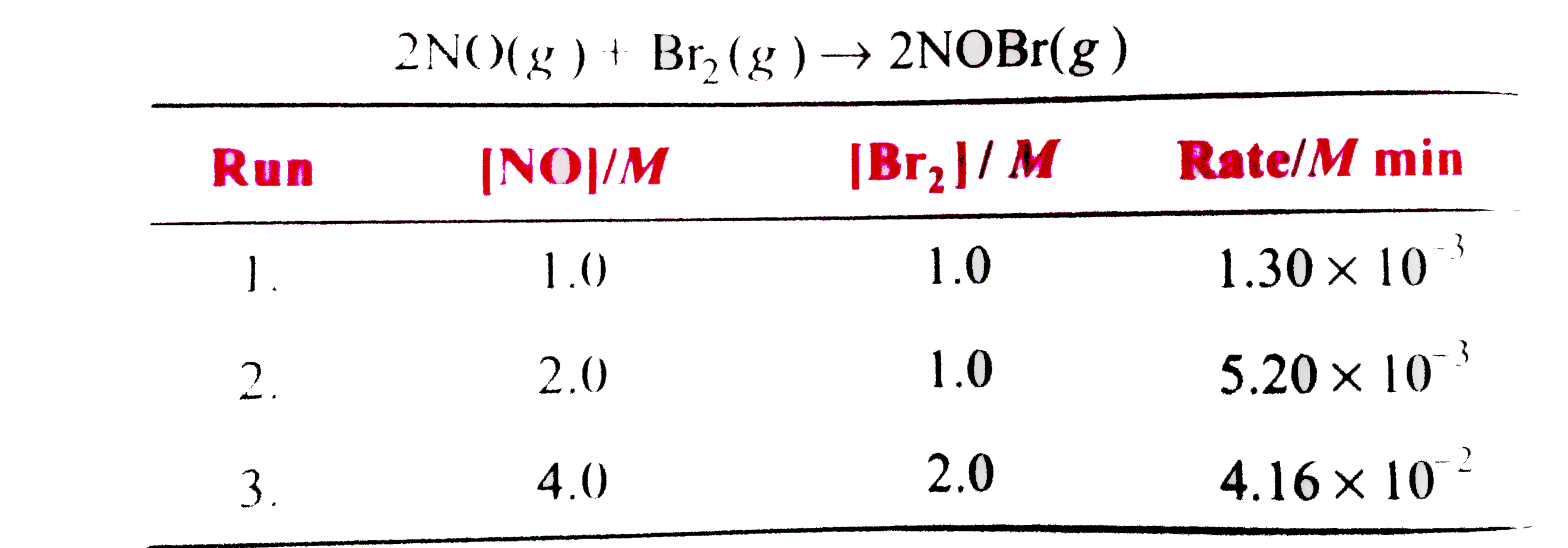
Determine the reaction rat law and the value of te rate constant.
View SolutionCalculate the half life of the first-order reaction:
C2H4O(g)→CH4(g)+CO(g)
The initial pressure of C2H4O(g) is 80mm and the total pressure at the end of 20min is 120mm.
View Solution