Explain qualitatively was elevation of boiling point of solution using Raoult's law.
Step-by-Step Solution:
1. Understanding Raoult's Law: Raoult's Law states that the vapor pressure of a solvent in a solution (P_solution) is directly proportional to the mole fraction of the solvent (X_solvent) in the solution. Mathematically, it can be expressed as:
Psolution=Xsolvent×P0solvent
where P0solvent is the vapor pressure of the pure solvent.
2. Effect of Adding a Non-Volatile Solute: When a non-volatile solute is added to a solvent, it does not contribute to the vapor pressure since it does not evaporate. This results in a decrease in the mole fraction of the solvent (X_solvent), leading to a reduction in the vapor pressure of the solution compared to that of the pure solvent.
3. Lowering of Vapor Pressure: The addition of the solute lowers the vapor pressure of the solvent because fewer solvent molecules are available to escape into the vapor phase. This lowering of vapor pressure can be quantified as:
ΔP=P0solvent−Psolution
where ΔP is the decrease in vapor pressure.
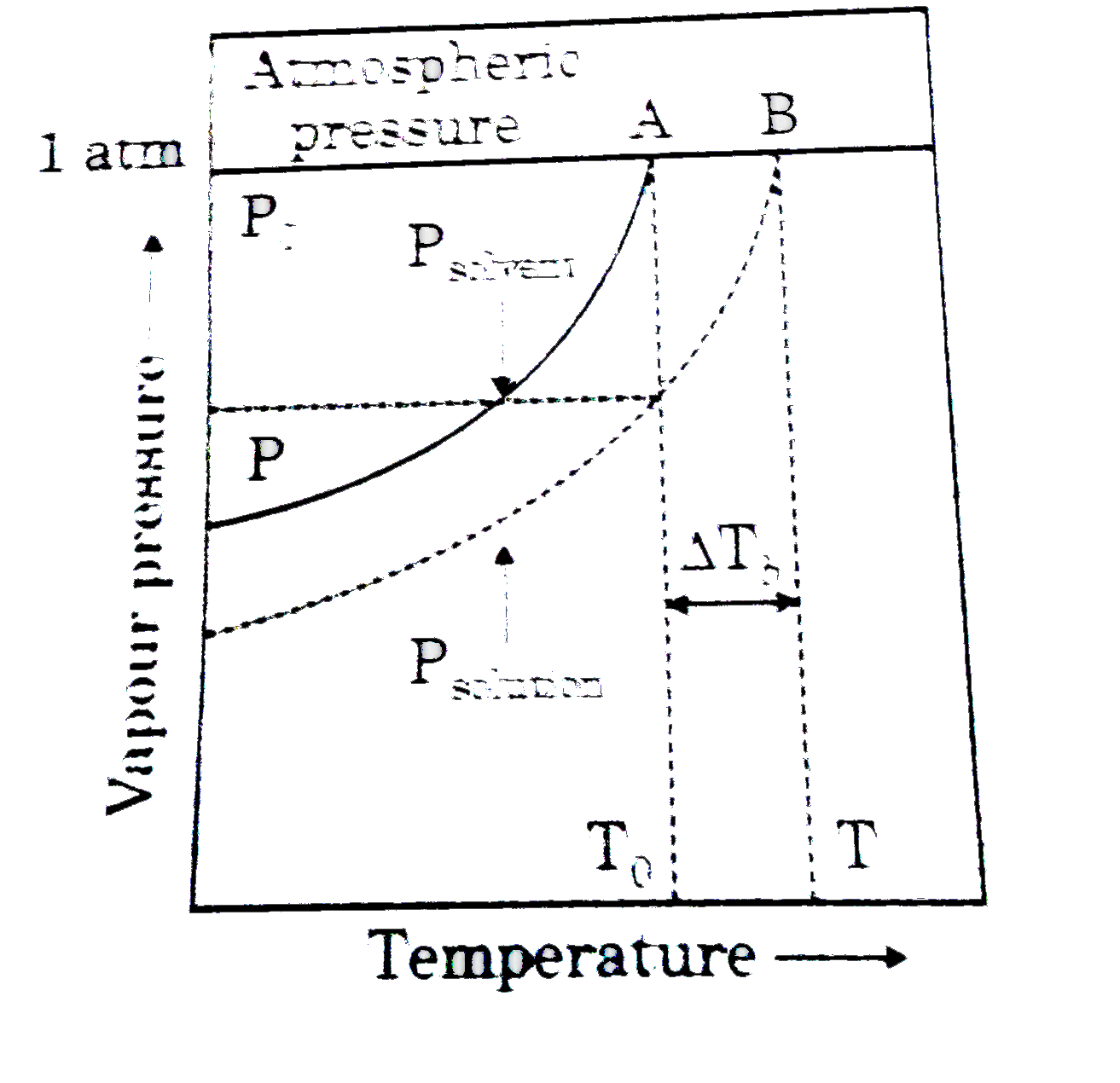
4. Boiling Point Elevation: The boiling point of a liquid is the temperature at which its vapor pressure equals the atmospheric pressure. Since the vapor pressure of the solution is lower than that of the pure solvent, the temperature must be increased to reach the boiling point. This increase in temperature is known as the elevation of boiling point (ΔTb).
5. Relationship Between Vapor Pressure and Boiling Point: The elevation in boiling point is directly proportional to the decrease in vapor pressure. This relationship can be expressed as:
ΔTb=Kb⋅m
where Kb is the ebullioscopic constant of the solvent and m is the molality of the solution.
6. Conclusion: Therefore, the elevation of boiling point of a solution is a direct consequence of the lowering of vapor pressure due to the presence of a non-volatile solute. The more solute particles present, the greater the lowering of vapor pressure, and consequently, the higher the boiling point of the solution.
Psolution=Xsolvent×P0solvent
where P0solvent is the vapor pressure of the pure solvent.
ΔP=P0solvent−Psolution
where ΔP is the decrease in vapor pressure.
ΔTb=Kb⋅m
where Kb is the ebullioscopic constant of the solvent and m is the molality of the solution.
Topper's Solved these Questions
- SOLUTIONSBOOK - PRADEEPCHAPTER - SOLUTIONSEXERCISE - ADDITIONAL QUESTIONS (LONG ANSWER QUESTIONS)
20 Videos
- SOLUTIONSBOOK - PRADEEPCHAPTER - SOLUTIONSEXERCISE - HIGHER ORDER THINKING SKILLS (OUESTIONS PROBLEMS WITH ANSWERS/SOLUTIONS) (HOTS PROBLEMS)
20 Videos
- SOLUTIONSBOOK - PRADEEPCHAPTER - SOLUTIONSEXERCISE - ADDITIONAL QUESTIONS (VERY SHORT ANSWER QUESTIONS)
41 Videos
- REDOX REACTIONS BOOK - PRADEEPCHAPTER - REDOX REACTIONS EXERCISE - Assertion reason type question
16 Videos
- SOME BASIC CONCEPTS OF CHEMISTRYBOOK - PRADEEPCHAPTER - SOME BASIC CONCEPTS OF CHEMISTRYEXERCISE - Competition (FOCUS) JEE (Main and Advanced)/Medical Entrance SPECIAL (VIII. Assertion-Reason Type Questions)(Type II)
13 Videos
Similar Questions
Knowledge Check
A solution which obeys Raoult's law is
ANormalBMolarCIdealDSaturatedA solution that obeys Raoult’s law is:
ANormalBMolarCIdealDSaturatedFigure explains elevation in boiling point when a non-volatile solute is added to a solvent. Variation of vapour pressure with temperaure and elevation in boiling point is marked.
Given that ΔTb is the elevation in boiling point of the solvent in a solution of molarity m then limm→0(ΔTbm) is equal to:AKb (molal elevation constant)BLv (latent heat of vaporisation)CΔS (entropy change)Dx (mole fraction of solute)
Explain why non ideal solutions deviate from Raoult's law.
View SolutionThe correct relation between elevation of boiling point and mass of solute is
View SolutionThe correct reaction between elevation of boiling point and molar of solute is
View SolutionThe elevation in boiling point for one molal solution of a solute in a solvent is called
View SolutionThe elevation in boiling point for one molal solution of a solute in a solvent is called
View Solution
