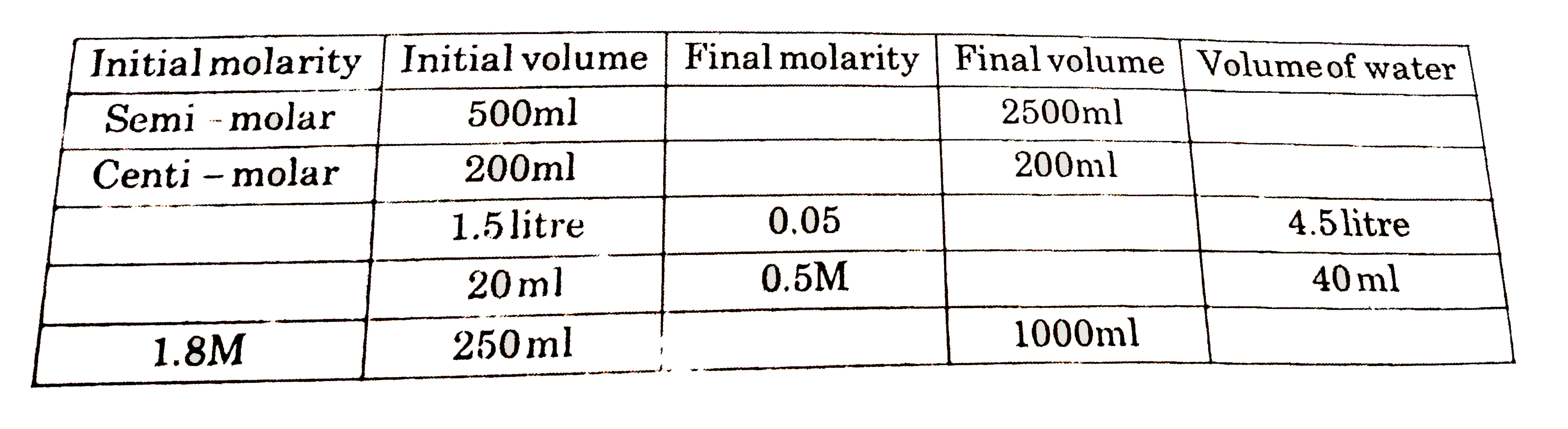
$V_{1}$ cc of solution having molarity $M_{1}$ is diluted to have molarity
$M_{2}$ . Derive expression (in terms of $M_{1}, M_{2} and V_{1})$ for the
volume of water required to be added.

Video Solution

Text Solution

Generated By DoubtnutGPT

To derive the expression for the volume of water required to be added when a solution of volume $V_{1}$ and molarity $M_{1}$ is diluted to a new molarity $M_{2}$ , we can follow these steps:
Step 1: Understand the concept of dilution
When a solution is diluted, the number of moles of solute remains constant before and after dilution. Therefore, we can use the relationship between molarity, volume, and moles of solute.
Step 2: Write the equation for the initial solution
The number of moles of solute in the initial solution can be expressed as:
$Moles of solute = M_{1} \times V_{1}$
Step 3: Write the equation for the diluted solution
After adding water, the new volume of the solution becomes $V_{1} + X$ , where $X$ is the volume of water added. The molarity of the diluted solution is $M_{2}$ , so the moles of solute in the diluted solution can be expressed as:
$Moles of solute = M_{2} \times (V_{1} + X)$
Step 4: Set the two expressions for moles of solute equal
Since the number of moles of solute remains unchanged, we can set the two expressions equal to each other:
$M_{1} \times V_{1} = M_{2} \times (V_{1} + X)$
Step 5: Expand and rearrange the equation
Expanding the right side gives:
$M_{1} \times V_{1} = M_{2} \times V_{1} + M_{2} \times X$
Now, rearranging the equation to isolate $X$ :
$M_{1} \times V_{1} - M_{2} \times V_{1} = M_{2} \times X$
$(M_{1} - M_{2}) \times V_{1} = M_{2} \times X$
Step 6: Solve for $X$
Now, we can solve for $X$ :
$X = \frac{(M_{1} - M_{2}) \times V_{1}}{M_{2}}$
Final Expression
Thus, the volume of water $X$ that needs to be added to dilute the solution is given by:
$X = \frac{(M_{1} - M_{2}) \times V_{1}}{M_{2}}$
---

Updated on:7/8/2024

The expression relating molarity (M) of a solution with its molarity (m) is
A $m = \frac{1000 M}{1000 d + M M_{B}}$
B $m = \frac{1000 M}{1000 d - M M_{a}}$
C $m = \frac{1000 d + M M_{a}}{1000 M}$
D $m = \frac{1000 d - M M_{B}}{1000 M}$
Two solution of $H_{2} S O_{4}$ of molarities x and y are mixed in the ratio of $V_{1} m L : V_{2} m L$ to form a solution of molarity $M_{1}$ . If they are mixed in the ratio of $V_{2} m L : V_{1} m L$ , they form a solution of molarity $M_{2}$ . Given $V_{1} / V_{2} = \frac{x}{y} > 1 and \frac{M_{1}}{M_{2}} = \frac{5}{4}$ , then $x : y$ is
A $2 : 1$
B $4 : 1$
C $1 : 2$
D $3 : 1$
The expression relating mole fraction of solute $(χ_{2})$ and molarity $(M)$ of the solution is: (where $d$ is the density of the solution in $g L^{- 1}$ and $M w_{1}$ and $M w_{2}$ are the molar masses of solvent and solute, respectively
A $x_{2} = \frac{M \times M w_{1}}{M (M w_{1} \times M w_{2}) + 1000 d}$
B $x_{2} = \frac{M \times M w_{1}}{M (M w_{1} \times M w_{2}) + d}$
C $x_{2} = \frac{M \times M w_{1}}{M (M w_{1} \times M w_{2}) - 1000 d}$
D $x_{2} = \frac{M \times M w_{1}}{M (M w_{1} \times M w_{2}) - d}$