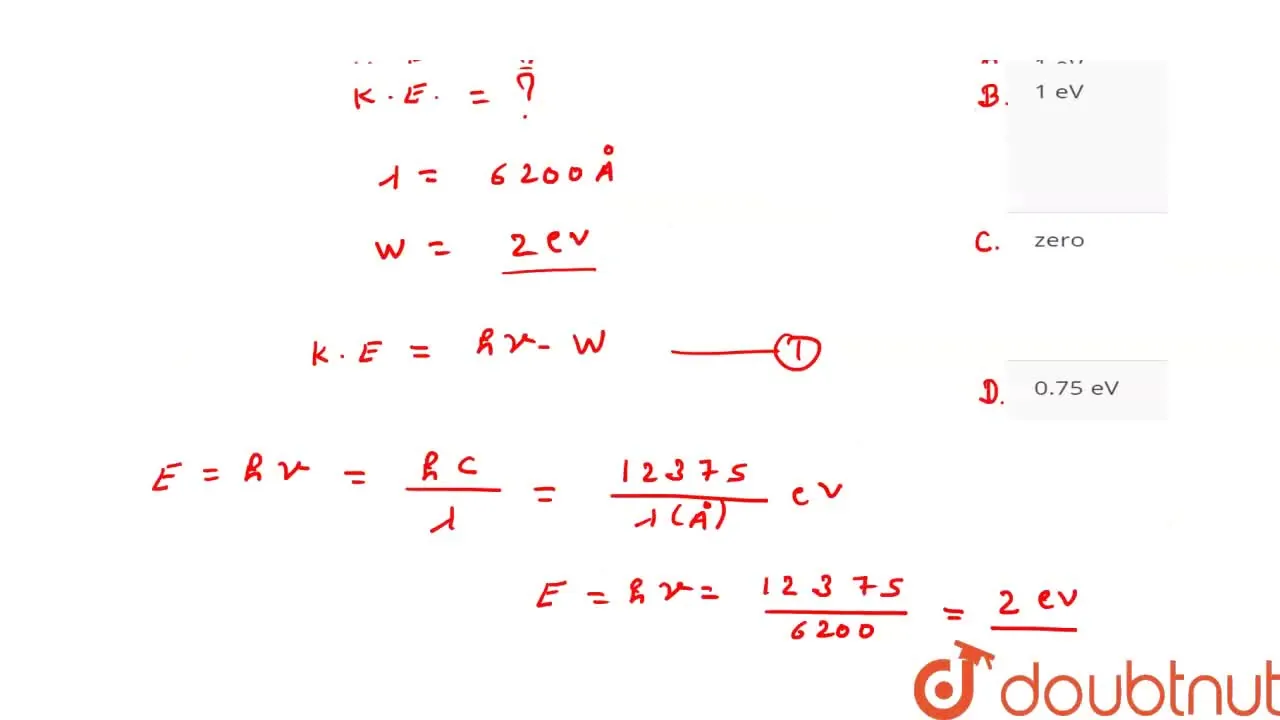
If light of wavelength 6200Å falls on a photosensitive surface of work function 2 eV, the kinetic energy of the most energetic photoelectron will be
0.5 eV
1 eV
zero
0.75 eV
The correct Answer is:C
but
Related Playlists
- BOOK - MARVEL PUBLICATIONCHAPTER - ELECTRONS AND PHOTONS EXERCISE - TEST YOUR GRASP - 1715videos
ELECTRONS AND PHOTONS
- BOOK - MARVEL PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS EXERCISE - TEST YOUR GRASP - 1630videos
ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS
- BOOK - MARVEL PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - TEST YOUR GRASP20videos
ELECTROSTATICS
Similar Questions
A light of wavelength 5000Å falls on a photosensitive plate with photoelectric work function 1.90 eV. Kinetic energy of the emitted photoelectrons will be (h=6.63×10−34J.s)
View SolutionLight of wavelength 5000Å falls on a sensitive plate with photoelectric work function of 1.9eV. The kinetic energy of the photoelectron emitted will be
View SolutionLight of wavelength 5000Å falls on a sensitive plate with photoelectric work function of 1.9eV. The kinetic energy of the photoelectron emitted will be
View SolutionLight of wavelenght 5000Å fall on a metal surface of work function 1.9eV Find
a. The energy of photon
b. The kinetic energy of photoelectronsView SolutionLight of wavelength 4000 Å falls on a photosensitive plate with photoelectric work function 1.96 eV. The K.E. of photoelectrons emitted will be :
View SolutionLight of wavelength 2000Å is incident on a metal surface of work function 3.0 eV. Find the minimum and maximum kinetic energy of the photoelectrons.
View SolutionA radiation of 2000Å falls on the metal whose work function is 4.2 eV. Then the kinetic energy of the fastest photo eletron is
View SolutionLight of wavelength 2000 Å falls on a metallic surface whose work function is 4.21 eV. Calculate the stopping potential
View SolutionLight of wavelength 250 nm falls on a photosensitive material with work function 3 eV. Find the stopping potential for this material.
View SolutionThe photoelectric work - function of potassium is 2.3 eV. If light
having a wavelength of 2800Å falls on potassium, find
(a) the kinetic energy in electron volts of the most energetic electrons ejected.
(b) the stopping potential in volts.View Solution