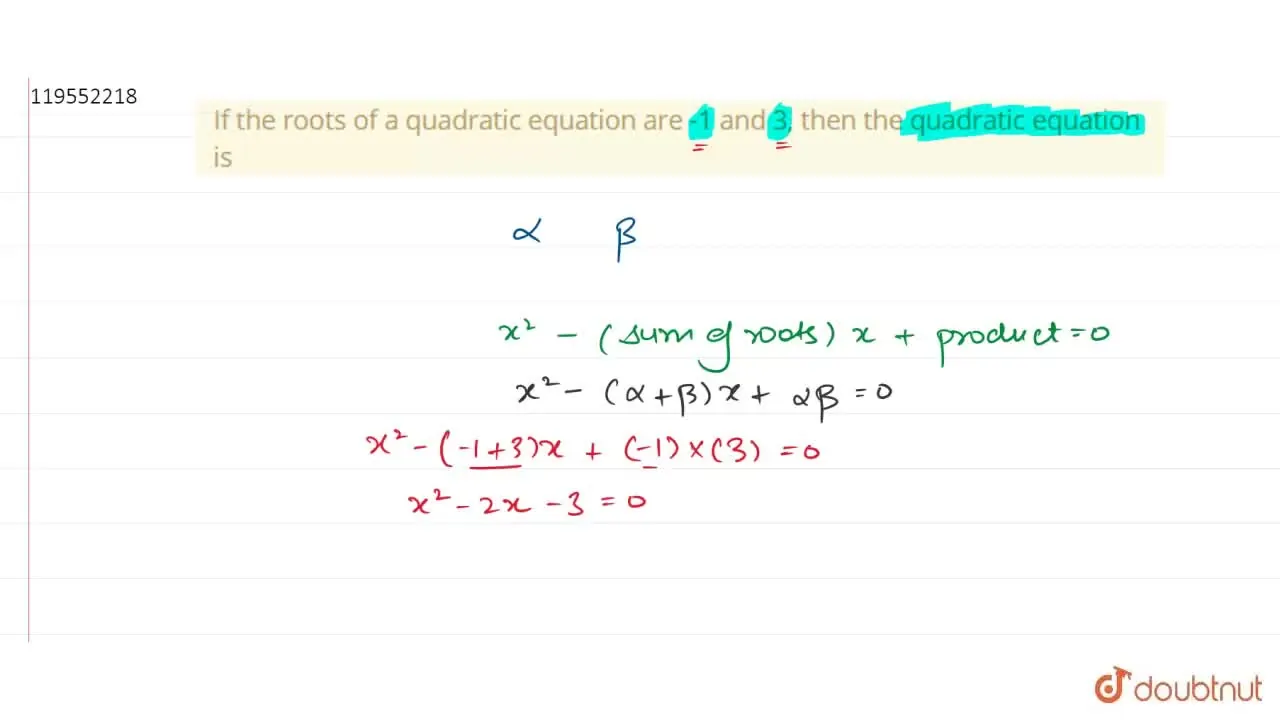
If the roots of a quadratic equation are -1 and 3, then the quadratic equation is

A

$x^{2} - 2 x - 3 = 0$

B

$x^{2} + 2 x - 3 = 0$

C

$x^{2} - 2 x + 3 = 0$

D

$x^{2} + 2 x + 3 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

|

Answer

Step by step video, text & image solution for If the roots of a quadratic equation are -1 and 3, then the quadratic equation is by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023

Class 10 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If the roots of a quadratic equation are m + n and m - n, then the quadratic equation will be :
AA. $x^{2} + 2 m x + m^{2} - m n + n^{2} = 0$
BB. $x^{2} + 2 m x + {(m - n)}^{2} = 0$
CC. $x^{2} - 2 m x + m^{2} - n^{2} = 0$
DD. $x^{2} + 2 m x + m^{2} - n^{2} = 0$
Question 2 - Select One
If the roots of the quadratic equation are $\frac{- 1}{2}$ and $\frac{3}{2}$ , then the equation is
A $4 x^{2} - 6 x + 3 = 0$
B $4 x^{2} - 5 x + 3 = 0$
C $4 x^{2} - 7 x + 3 = 0$
D $4 x^{2} - 4 x - 3 = 0$
Question 3 - Select One
The roots of a quadratic equation are $5$ and $- 2$ . Then, the equation is
A $x^{2} - 3 x + 10 = 0$
B $x^{2} - 3 x - 10 = 0$
C $x^{2} + 3 x - 10 = 0$
D $x^{2} + 3 x + 10 = 0$

TARGET PUBLICATION-QUADRATIC EQUATIONS-Multiple Choice Questions

Home
Ask Doubt
Profile