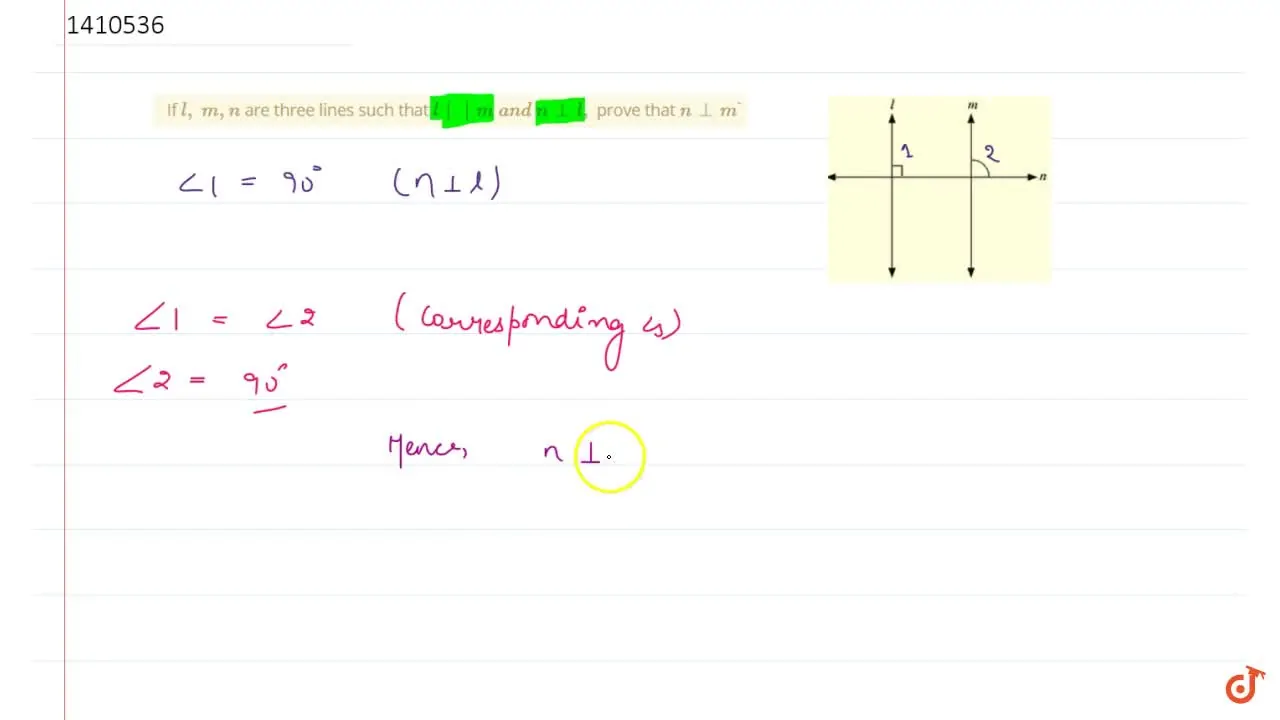
If $l, m, n$ are three lines such that $l ∣ ∣ m$ and $n ⊥ l,$ prove that $n ⊥ m .$

Video Solution

Text Solution

Generated By DoubtnutGPT

To prove that line $n$ is perpendicular to line $m$ given that line $l$ is parallel to line $m$ and line $n$ is perpendicular to line $l$ , we can follow these steps:
1. Identify the Given Information:
- We have three lines: $l$ , $m$ , and $n$ .
- It is given that $l ∥ m$ (lines $l$ and $m$ are parallel).
- It is also given that $n ⊥ l$ (line $n$ is perpendicular to line $l$ ).
2. Understand the Implications of the Given Information:
- Since $n ⊥ l$ , the angle formed between line $n$ and line $l$ is $90^{\circ}$ .
- Let's denote this angle as $∠ 1 = 90^{\circ}$ .
3. Use the Properties of Parallel Lines:
- Since $l ∥ m$ and $n$ acts as a transversal line intersecting both $l$ and $m$ , we can apply the property of corresponding angles.
- The angle formed between line $n$ and line $m$ at the point of intersection can be denoted as $∠ 2$ .
4. Establish the Relationship Between the Angles:
- According to the Corresponding Angles Postulate, if two parallel lines are cut by a transversal, then each pair of corresponding angles are equal.
- Therefore, $∠ 1 = ∠ 2$ .
5. Substituting the Known Values:
- We know that $∠ 1 = 90^{\circ}$ .
- Hence, $∠ 2 = 90^{\circ}$ .
6. Conclude the Proof:
- Since $∠ 2 = 90^{\circ}$ , it follows that line $n$ is perpendicular to line $m$ .
- Therefore, we can conclude that $n ⊥ m$ .
Final Statement:
Thus, we have proven that if $l ∥ m$ and $n ⊥ l$ , then $n ⊥ m$ .
---

Updated on:7/8/2024

If $l$ , m, n are the direction consines of a line, then
A $l + m + n = 0$
B $l + m + n = 1$
C $l^{2} + m^{2} + n^{2} = 1$
D $l^{2} + m^{2} + n^{2} = 0$
If l,m,n are direction cosines of the line then $- l, - m, - n$ can be
Aonly direction ratios of the line
Bonly direction cosines of the line
Cdirection cosines and direction ratios of the line
Dneither direction cosines nor direction ratios of the line
If l, m, n are direction cosines of a line then $l ˆ i + m ˆ j + n ˆ k$ is________
ANull vector
Bthe unit vector along the line.
CAny vector along the line
Da vector perpendicular to the line