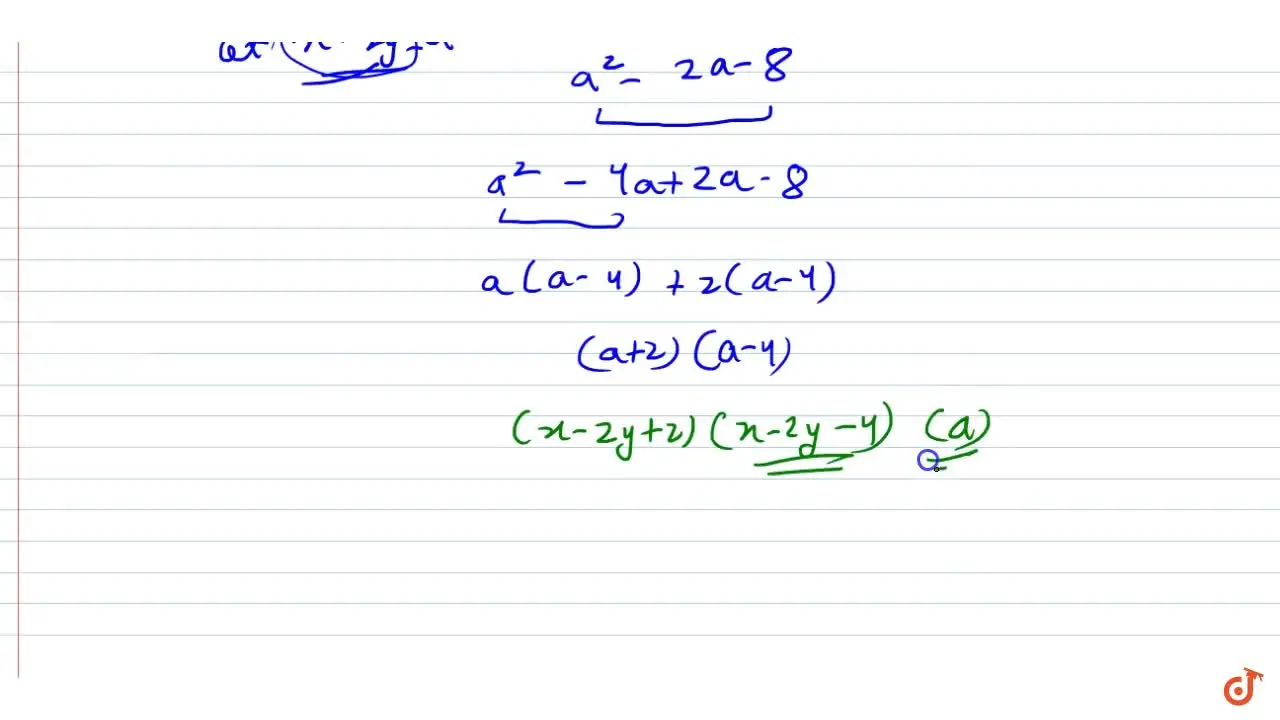The factors of x2+4y2+4y−4xy−2x−8 are (a)(x−2y−4)(x−2y+2) (b) (x−y+2)(x−4y−4) (c)(x+2y−4)(x+2y+2) (d) none of these
Video Solution
Text Solution
Correct option is (A)
The value of x2+4y2+4y−4xy−2x−8 is
=x2+4y2+4y−4xy−2x−8
=(x−2y)2+2(2y−x)−8
=(x−2y)2−2(x−2y)−8
Let A=x−2y
=(A−4)(A+2)
Re-substitute the value of A
Thus,
x2+4y2+4y−4xy−2x−8=(x−2y−4)(x−2y+2)
|
Updated on:21/07/2023
Related Playlists
Similar Questions
The factors of x2+4y2+4y−4xy−2x−8 are (a)(x−2y−4)(x−2y+2) (b) (x−y+2)(x−4y−4) (c)(x+2y−4)(x+2y+2) (d) none of these
View SolutionWhat are the factors of x2+4y2+4y−4xy−2x−8 ?
View SolutionThe factor form x4+x2y2+y4 is
View SolutionThe factors of (x4−7x2y2+y4) are
View SolutionFactorize: x4+x2y2+y4
View SolutionFactorize the following: 4x2−y2+4y−4
View SolutionFactorise : x4+y4−2x2y2
View Solution(3x−4y)2−(4x+3y)2=______.
View SolutionThe equation of the circle passing through (12,−1) and having pair of straight lines x2−y2+3x+y+2=0 as its two diameters is : (A) 4x2+4y2+12x−4y−15=0 (B) 4x2+4y2+15x+4y−12=0 (C) 4x2+4y2−4x+8y+5=0 (D) none of these
View SolutionFactorise x4+x2y2+y4.
View Solution