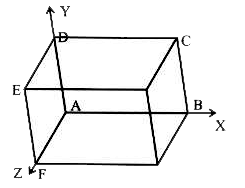
A loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
B0L2Wb
2B0L2Wb.
2B0L2Wb.
4B0L2Wb.
The correct Answer is:B
To find the magnetic flux passing through the loop ABCDEFA, we can follow these steps:
Step 1: Identify the Coordinates of the Points
The coordinates of the points are given as:
- A(0,0,0)
- B(L,0,0)
- C(L,L,0)
- D(0,L,0)
- E(0,L,L)
- F(0,0,L)
Step 2: Determine the Magnetic Field
The magnetic field is given as:
B=B0(^i+^k)T
Step 3: Calculate the Area of the Loop
The loop consists of two rectangular areas:
1. Area ABCD lies in the xy-plane.
2. Area AFE lies in the yz-plane.
Area ABCD:
- The area vector A1 for the rectangle ABCD is perpendicular to the xy-plane and points in the z-direction:
A1=L2^k
Area AFE:
- The area vector A2 for the rectangle AFE is perpendicular to the yz-plane and points in the x-direction:
A2=L2^i
Step 4: Total Area Vector
The total area vector A is the sum of the two area vectors:
A=A1+A2=L2^k+L2^i=L2(^i+^k)
Step 5: Calculate the Magnetic Flux
The magnetic flux Φ through the loop is given by the dot product of the magnetic field B and the area vector A:
Φ=B⋅A
Substituting the values:
Φ=B0(^i+^k)⋅L2(^i+^k)
Step 6: Perform the Dot Product
Calculating the dot product:
Φ=B0L2(^i⋅^i+^i⋅^k+^k⋅^i+^k⋅^k)
=B0L2(1+0+0+1)=B0L2⋅2
Final Answer
Thus, the magnetic flux through the loop is:
Φ=2B0L2Wb
The coordinates of the points are given as:
- A(0,0,0)
- B(L,0,0)
- C(L,L,0)
- D(0,L,0)
- E(0,L,L)
- F(0,0,L)
The magnetic field is given as:
B=B0(^i+^k)T
The loop consists of two rectangular areas:
1. Area ABCD lies in the xy-plane.
2. Area AFE lies in the yz-plane.
- The area vector A1 for the rectangle ABCD is perpendicular to the xy-plane and points in the z-direction:
A1=L2^k
- The area vector A2 for the rectangle AFE is perpendicular to the yz-plane and points in the x-direction:
A2=L2^i
The total area vector A is the sum of the two area vectors:
A=A1+A2=L2^k+L2^i=L2(^i+^k)
The magnetic flux Φ through the loop is given by the dot product of the magnetic field B and the area vector A:
Φ=B⋅A
Φ=B0(^i+^k)⋅L2(^i+^k)
Calculating the dot product:
Φ=B0L2(^i⋅^i+^i⋅^k+^k⋅^i+^k⋅^k)
=B0L2(1+0+0+1)=B0L2⋅2
Thus, the magnetic flux through the loop is:
Φ=2B0L2Wb
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Single Answer Questions Level -V11videos
ELECTRO MAGNETIC INDUCTION
- BOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Comprehension Type Questions22videos
ELECTRO MAGNETIC INDUCTION
- BOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Level - III64videos
ELECTRO MAGNETIC INDUCTION
- BOOK - NARAYNACHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - EXERCISE -443videos
ELECTRIC CHARGES AND FIELDS
- BOOK - NARAYNACHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - LEVEL-II(H.W)14videos
ELECTRO MAGNETIC WAVES
Similar Questions
A loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View SolutionA loop made of straight edegs has six corners at A(0,0,0),B(L,O,0)C(L,L,0),D(0,L,0)E(0,L,L) and F(0,0,L). Where L is in meter. A magnetic field B=B0(ˆi+ˆk)T is present in the region. The flux passing through the loop ABCDEFA (in that order) is
View Solution
Knowledge Check
- Question 1 - Select One
A conductor AB of length l moves in xy plane with velocity →v=v0(ˆi−ˆj). A magnetic field →B=B0(ˆi+ˆj) exists in the region. The induced emf is
AzeroB2B0lv0CB0lv0D√2B0lv0 - Question 2 - Select One
A square of side L meters lies in the x-y plane in a region, where the magnetic field is give by B=B0(2ˆi+3ˆj+4ˆk)T, where B0 is constant. The magnitude of flux passing through the square is
A2B0L2WbB3B0L2WbC4B0L2WbD√29B0L2Wb
A loop A(0,0,0)B(5,0,0)C(5,5,0)D(0,5,0)E(0,5,10) and F(0,0,10) of straight edges has six corner points and two sides. The magnetic field in this region is →B=(3ˆi+4ˆk) T. The quantity of flux through the loop ABCDEFA (in Wb) is
 View Solution
View SolutionL1=L0+rt. What does L_(0) represent in the given expression?
View SolutionConsider a loop ABCDEFA. With coordinates A (0, 0, 0), B(5, 0, 0), C(5, 5, 0), D(0, 5, 0) E(0, 5, 5) and F(0, 0, 5). Find magnetic flux through loop due to magnetic field →B=3ˆi+4ˆk
View SolutionA loop ABCA of straight edges has three corner points A (8, 0, 0), B (0, 8, 0 ) and C (0, 0, 8). The magnetic field in this region is →B=5(ˆi+ˆj+ˆk)T0 . The quantity of flux through the loop ABCA (in Wb) is _____.
View SolutionA square shaped, conducting wire loop of side L, total mass m and total resistance R initially lies in the horizontal x−y plane, with corners at (x,y,z)=(0,0,0),(0,L,0),(L,0,0),and(L,L,0). There is a uniform upward magnetic field in the space within and around the loop. The side of the loop that extends from (0,0,0)→(L,0,0) is held in place on the x-axis, the rest of the loop is released, it begins to rotate due to the gravitational torque.
(a) Find the net torque (magnitude and direction)that acts on the loop when it has rotated through an anglr ϕ from its original orientation and is rotating dounward at an angular speed ω.
(b) Find the angular acceleration of the loop at the instant described in part (a).
( c) Compared to teh case with zero magnetic field, does it take the loop a longer or shorter time to rotate through 90∘ ? Explain.
(d) Is mechanical energy conserved as the loop rotates downward ? Explain.View SolutionA square of side L meters lies in the x−y plane in a region where tbe magnctic fleld is given by →B=B0(2ˆi+3ˆj+4ˆk) tesla _(3) where B0 is a constant. If the magnitude of flux passing through the square is yBaL2 weber, then find the value of y.
View Solution

