Two long parallel wires are separated by a distance of 2m. They carry a current of 1A each in opposite direction. The magnetic induction at the midpoint of a straight line connecting these two wires is
zero
2×10−7T
4×10−5T
4×10−7T
The correct Answer is:4
To solve the problem of finding the magnetic induction at the midpoint of two long parallel wires carrying currents in opposite directions, we can follow these steps:
Step 1: Understand the Setup
We have two long parallel wires separated by a distance of 2 meters, with each carrying a current of 1 A in opposite directions. The midpoint between the two wires is 1 meter away from each wire.
Step 2: Use the Formula for Magnetic Field due to a Long Straight Current-Carrying Wire
The magnetic field (B) at a distance (r) from a long straight wire carrying current (I) is given by the formula:
B=μ0I2πr
where:
- μ0 is the permeability of free space (μ0=4π×10−7T m/A),
- I is the current in amperes,
- r is the distance from the wire in meters.
Step 3: Calculate the Magnetic Field from Each Wire at the Midpoint
Since the midpoint is 1 meter away from each wire, we can calculate the magnetic field due to each wire at this point.
For Wire 1 (carrying current I1=1A):
B1=μ0I12πr1=4π×10−7×12π×1=4×10−72=2×10−7T
For Wire 2 (carrying current I2=1A in the opposite direction):
B2=μ0I22πr2=4π×10−7×12π×1=4×10−72=2×10−7T
Step 4: Determine the Direction of the Magnetic Fields
Using the right-hand rule:
- For Wire 1, the magnetic field at the midpoint will be directed out of the page.
- For Wire 2, the magnetic field at the midpoint will be directed into the page.
Step 5: Calculate the Net Magnetic Field
Since the magnetic fields from the two wires are in opposite directions, we subtract the magnitudes:
Bnet=B1−B2=2×10−7−2×10−7=0T
Conclusion
The magnetic induction at the midpoint of the straight line connecting the two wires is:
Bnet=0T
We have two long parallel wires separated by a distance of 2 meters, with each carrying a current of 1 A in opposite directions. The midpoint between the two wires is 1 meter away from each wire.
The magnetic field (B) at a distance (r) from a long straight wire carrying current (I) is given by the formula:
B=μ0I2πr
where:
- μ0 is the permeability of free space (μ0=4π×10−7T m/A),
- I is the current in amperes,
- r is the distance from the wire in meters.
Since the midpoint is 1 meter away from each wire, we can calculate the magnetic field due to each wire at this point.
B1=μ0I12πr1=4π×10−7×12π×1=4×10−72=2×10−7T
B2=μ0I22πr2=4π×10−7×12π×1=4×10−72=2×10−7T
Using the right-hand rule:
- For Wire 1, the magnetic field at the midpoint will be directed out of the page.
- For Wire 2, the magnetic field at the midpoint will be directed into the page.
Since the magnetic fields from the two wires are in opposite directions, we subtract the magnitudes:
Bnet=B1−B2=2×10−7−2×10−7=0T
The magnetic induction at the midpoint of the straight line connecting the two wires is:
Bnet=0T
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBook:NARAYNAChapter:MOVING CHARGES AND MAGNETISMExercise:ILLUSTRATION
Explore 58 Videos
- MOVING CHARGES AND MAGNETISMBook:NARAYNAChapter:MOVING CHARGES AND MAGNETISMExercise:C.U.Q (AMPERE.S CIRCUITAL LAW MAGNETIC FIELD DUE TO STRAIGHT CONDUCTOR )
Explore 28 Videos
- MOVING CHARGES AND MAGNETISMBook:NARAYNAChapter:MOVING CHARGES AND MAGNETISMExercise:Level =I (H.W)
Explore 42 Videos
- MAGNETISM AND MATTERBook:NARAYNAChapter:MAGNETISM AND MATTERExercise:EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
Explore 17 Videos
- NUCLEAR PHYSICSBook:NARAYNAChapter:NUCLEAR PHYSICSExercise:LEVEL-II-(H.W)
Explore 9 Videos
Similar Questions
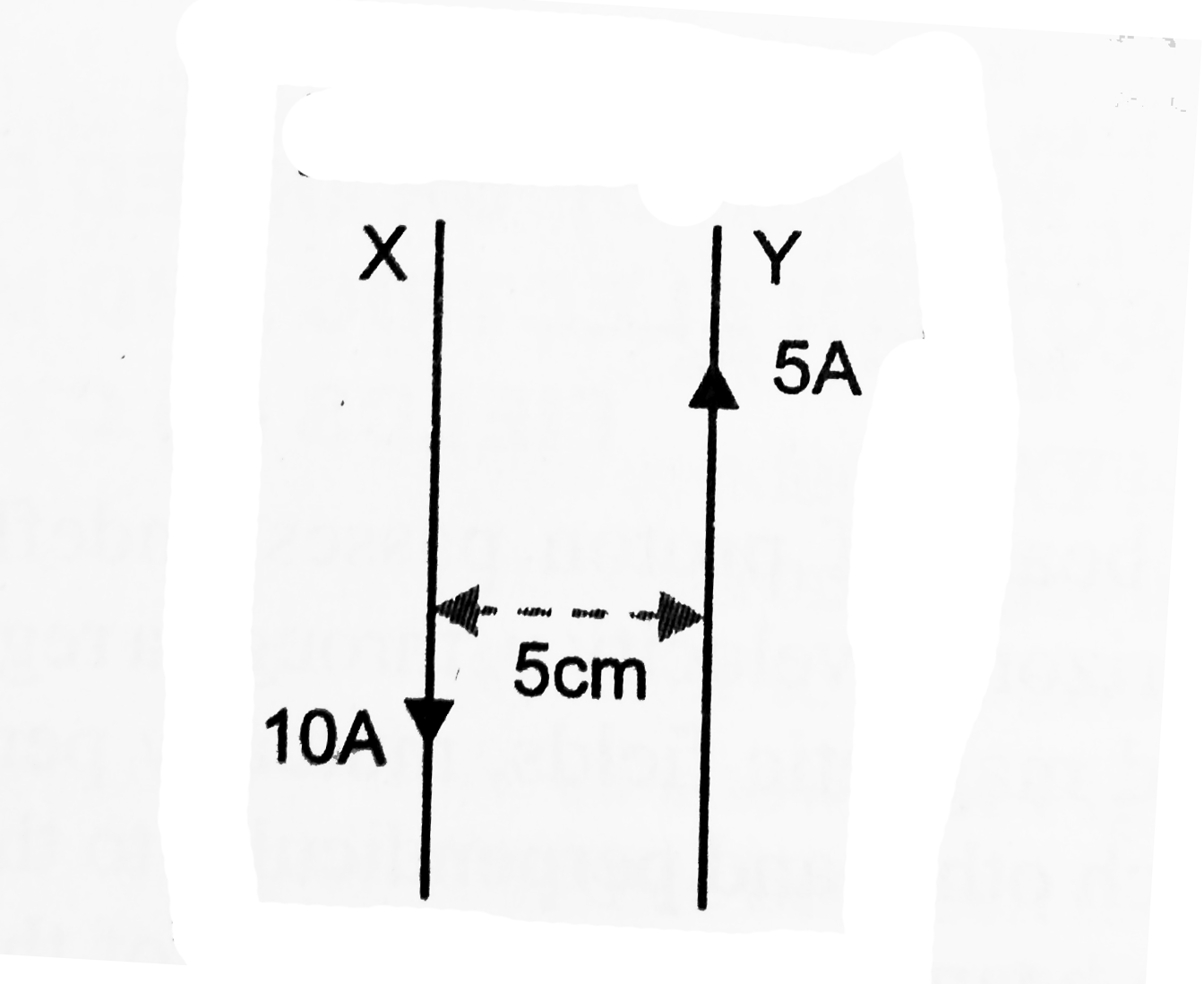
Two long parallel straight wires X and Y separated by a distance 5cm in air carry currents of 10A and 5A respectively in opposite directions. Calculate the magnitude and direction of force on a 20cm length of the wire Y. Figure
View SolutionTwo free parallel wires carrying currents in opposite direction
View Solution
Knowledge Check
Two thin, long, parallel wires, separated by a distance 'd' carry a current of 'i' A in the same direction. They will
Arepel each other with a force of μ0i2/(2πd)Battract each other with a force of μ0i2/(2πd)Crepel each other with a force of μ0i2/(2πd2)Dattract each other with a force of μ0i2/(2πd2)The magnetic induction at any point due to a long straight wire carrying a current is
AProportional to the distance from the wireBInversely proportional to the distance from wireCInversely proportional to the square of the distance from the wireDDoes not depend on distanceTwo long straight horizontal parallel wires one above the other are separated by a distance '2a' . If the wires carry equal currents in opposite directions, the magnitude of the magnitude induction in the plane of the wires at a distance 'a' above the upper wire is
Aμ02πaBμ02πa+μ0i4πaCμ0i2πa−μi4πaDμ0i3πa
Two free parallel wires carrying currents in opposite directions
View SolutionTwo free parallel wires carrying currents in opposite directions
View SolutionTwo free parallel wires carrying currents in opposite directions
View SolutionTwo free parallel wires carrying currents in opposite directions
View SolutionTwo free parallel wires carrying currents in opposite directions
View Solution