An α− particle moves from E to W in a magnetic field perpendicular to the plane of the paper and into the paper. The particle is defelcted towards
East
West
South
North
The correct Answer is:3
To solve the problem, we need to determine the direction in which an alpha particle, moving from East to West, will be deflected when it enters a magnetic field that is directed into the paper (perpendicular to the plane of the paper).
1. Identify the Direction of Motion of the Alpha Particle:
- The alpha particle is moving from East to West.
2. Identify the Direction of the Magnetic Field:
- The magnetic field is directed into the paper. This is often represented by dots (•) on the paper, indicating that the field lines are coming out towards you.
3. Determine the Charge of the Alpha Particle:
- An alpha particle is a positively charged particle (it consists of 2 protons and 2 neutrons).
4. Use the Right-Hand Rule:
- To find the direction of the force acting on the charged particle due to the magnetic field, we can use the right-hand rule.
- Point your thumb in the direction of the velocity of the particle (East to West).
- Point your fingers in the direction of the magnetic field (into the paper).
- Your palm will then face the direction of the force acting on a positive charge.
5. Determine the Direction of Deflection:
- According to the right-hand rule, the force acting on the positively charged alpha particle will be directed downwards (towards the South).
6. Conclusion:
- Therefore, the alpha particle will be deflected towards the South.
Final Answer:
The alpha particle is deflected towards the South.
---
- The alpha particle is moving from East to West.
- The magnetic field is directed into the paper. This is often represented by dots (•) on the paper, indicating that the field lines are coming out towards you.
- An alpha particle is a positively charged particle (it consists of 2 protons and 2 neutrons).
- To find the direction of the force acting on the charged particle due to the magnetic field, we can use the right-hand rule.
- Point your thumb in the direction of the velocity of the particle (East to West).
- Point your fingers in the direction of the magnetic field (into the paper).
- Your palm will then face the direction of the force acting on a positive charge.
- According to the right-hand rule, the force acting on the positively charged alpha particle will be directed downwards (towards the South).
- Therefore, the alpha particle will be deflected towards the South.
The alpha particle is deflected towards the South.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - NARAYNACHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - LEVEL-I(C.W)
58 Videos
- MOVING CHARGES AND MAGNETISMBOOK - NARAYNACHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Level-II(C.W)
63 Videos
- MOVING CHARGES AND MAGNETISMBOOK - NARAYNACHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE - 4
20 Videos
- MAGNETISM AND MATTERBOOK - NARAYNACHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
17 Videos
- NUCLEAR PHYSICSBOOK - NARAYNACHAPTER - NUCLEAR PHYSICSEXERCISE - LEVEL-II-(H.W)
9 Videos
Similar Questions
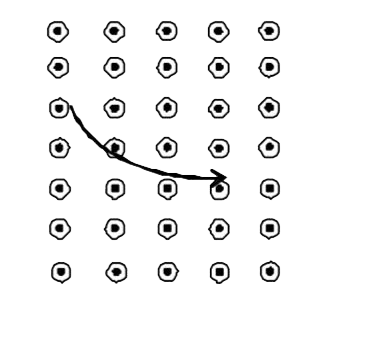
In a region of space a uniform magnetic field perpendicular to the plane of the page and directed towards reader exists. A particle has a trajectory as shown in fig. The particle is-
View SolutionA charged particle enters a uniform magnetic field perpendicular to it. The magnetic field
View Solution
Knowledge Check
A charged particle moves through a magnetic field perpendicular to its direction. Then
Akinetic energy changes but the momentum is constantBthe momentum changes but the kinetic energy is constantCboth momentum and kinetic energy of the particle are not constantDboth momentum and kinetic energy of the particle are constantA charged particle moves through a magnetic field perpendicular to its direction. Then
AKinetic energy changes but the momentum is constantBthe momentum changes but the kinetic energy is constantCboth momentum and kinetic energy of the particle are not constantDboth momentum and kinetic energy of the particle are constantA charged particle moves through a magnetic field perpendicular to its direction. Then
AAcceleration remain unchangedBvelocity remains unchangedCspeed of the particle remains unchangedDDirection of the particle remains unchanged
When a charged particle moves perpendicular to a magnetic field, then:
View SolutionA particle is moving in a uniform magnetic field, then
View SolutionWhen a charged particle moves perpendicular to a magnetic field, then:
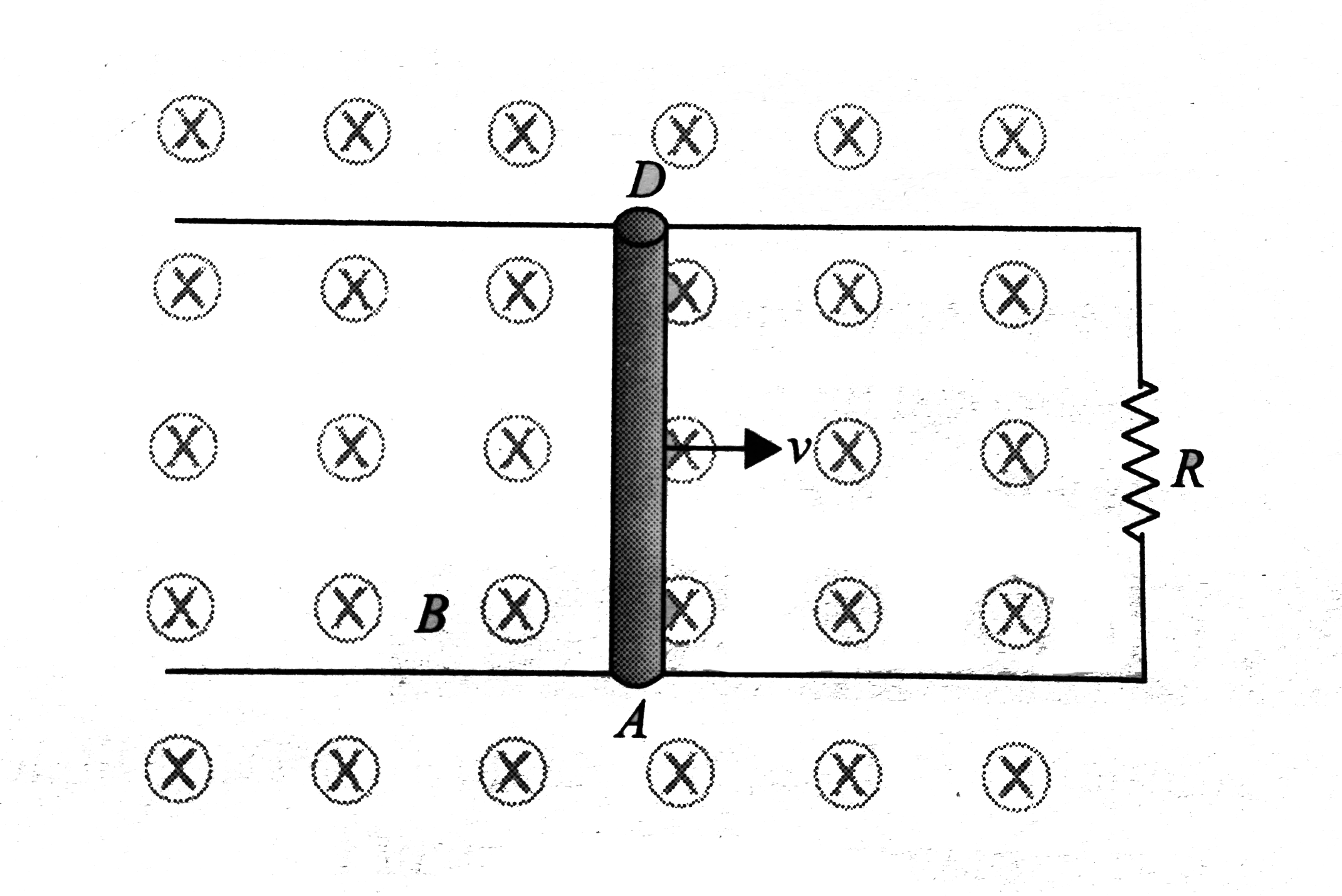
View SolutionThe counductor AD moves to the right in a uniform magnetic field directed into the plane of the paper.
View SolutionA charged particle is moving in a magnetic field of strength B perpendicular to the direction of the field. If q and m denote the charge and mass of the particle respectively, then the frequency of rotation of the particle is
View Solution
