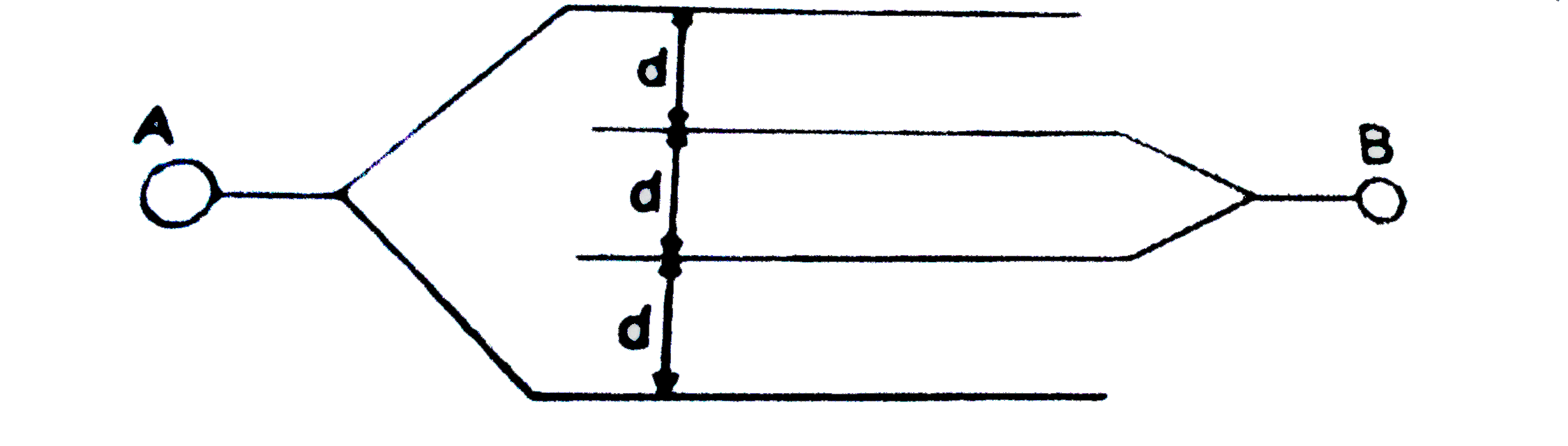
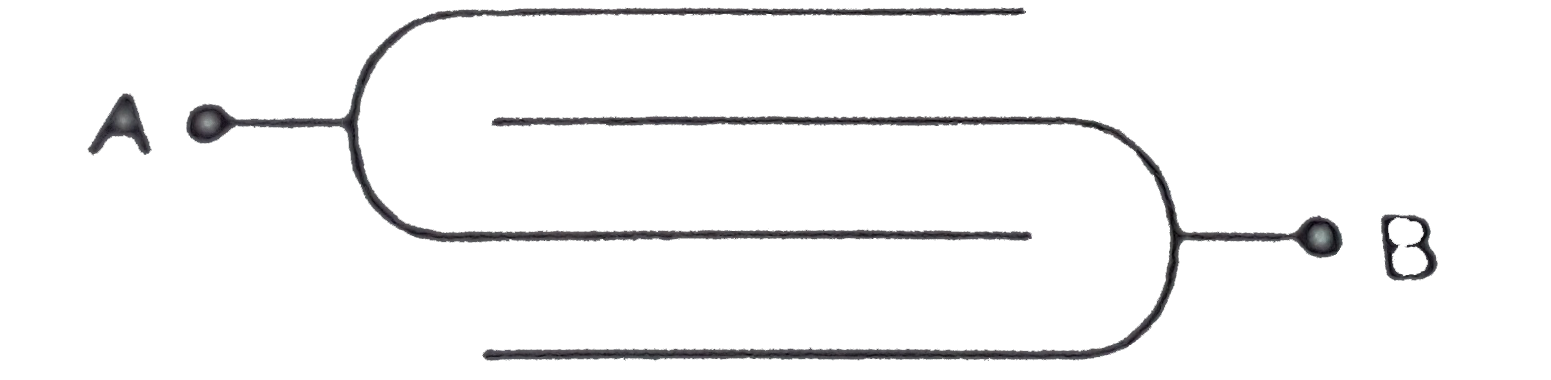
Find the equivalent capacitance between A and B.A: area of each plate, d: separation between the plates.
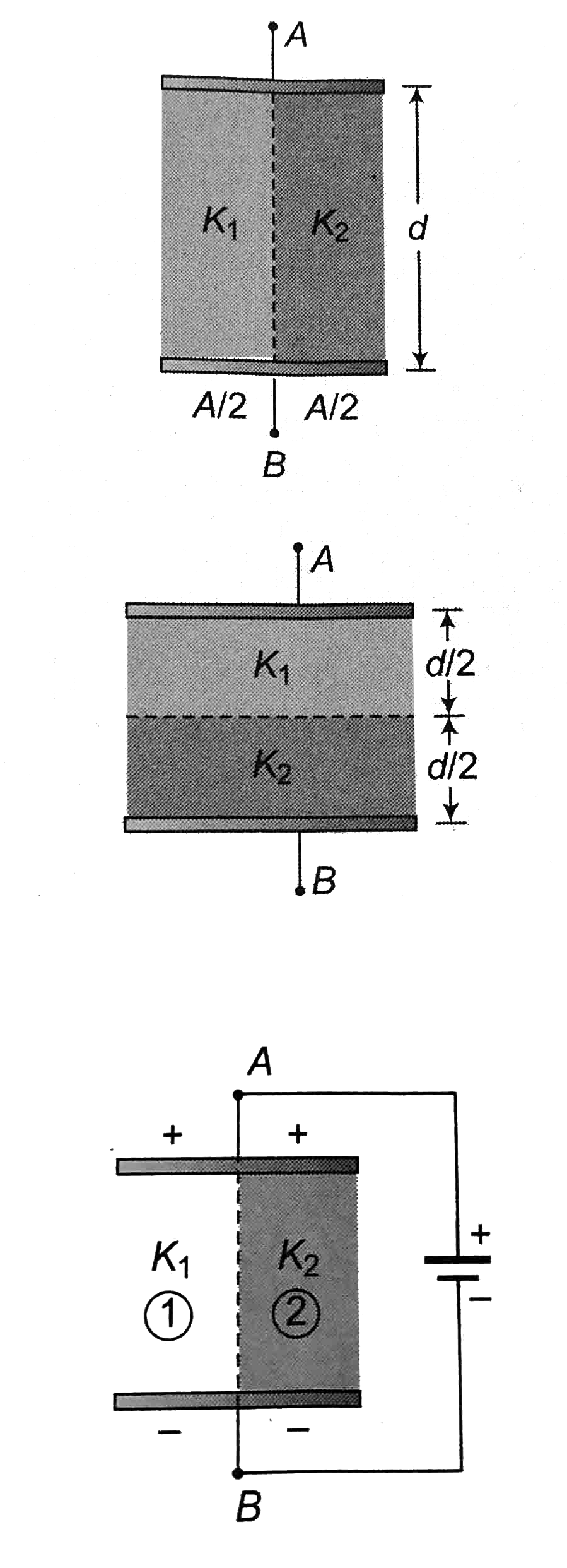
(a) Capacitor ①,C_(1)=(K_(1)(A)/(2)in_(0))/(d)=(K_(1)Ain_(0))/(2d)
Capacitor ②,C_(2)=(K_(2)(A)/(2)in_(0))/(d)=(K_(2)Ain_(0))/(2d)
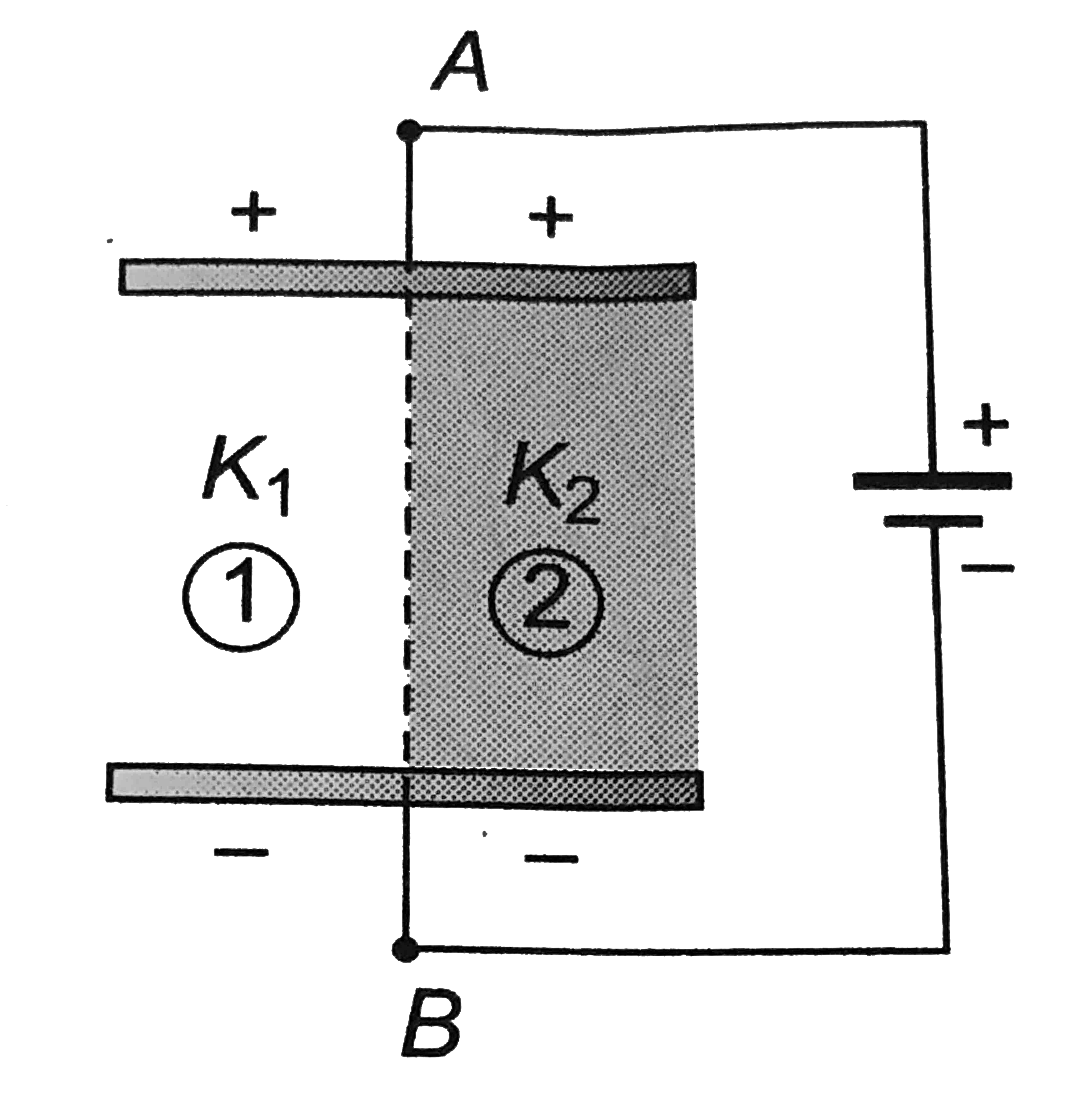
Now connect a battery between A and B, as shown. The positive plates of two capacitors are connected to A and negative plates to B, hence C1 and C2 are in parallel. here, we are deciding series/parallel with the help of polarity.
Ceq=C1+C2=(K1+K22)A∈0d
(b) C1=K1A∈0d/2=2K1A∈0d
C2=K2A∈0d/2=2K2A∈0d
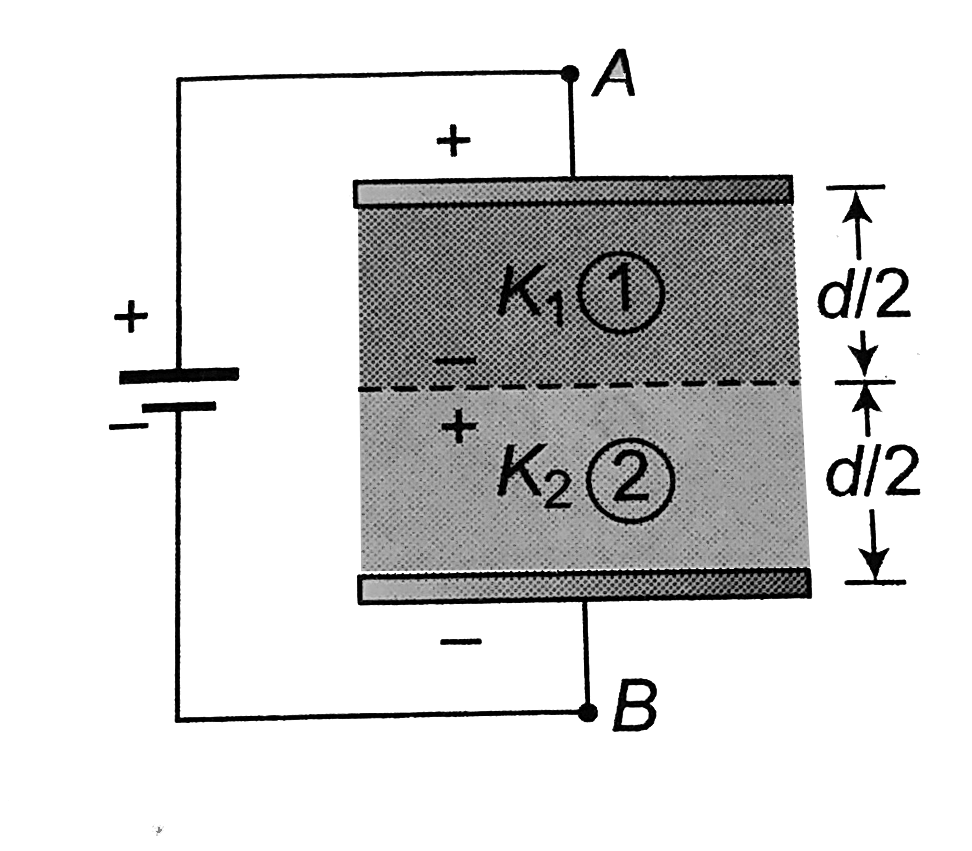
Now, check polarity by connecting a battery between A and B. As shown in diagram, between A and B, we have, +,−,+,−i.e.,C1 and C2 are in series.
Ceq=C1C2C1+C2=(2K1K2K1+K2)A∈0d
 .
.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Four metal plates are arranged as shown in the figure. Capacitance between X and Y (A→ Area of each plate, d→distance between the plates) is
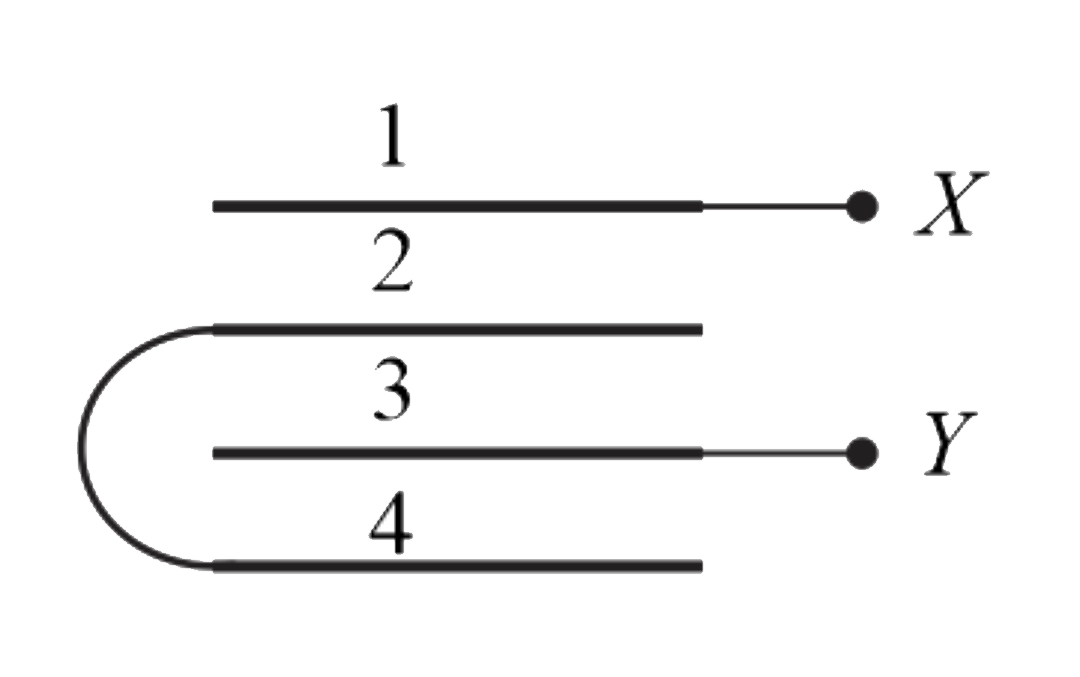 A32ε0AdB2ε0AdC23εAdD3ε0Ad
A32ε0AdB2ε0AdC23εAdD3ε0AdThe diagram shows an arrangement of identical metal plates placed parallel to each other and the variation of potential between the paltes by dotted lilne. Using the details given in diagram, the equivalent capacitance connected across the plate No. 2 and plate No. 4 is
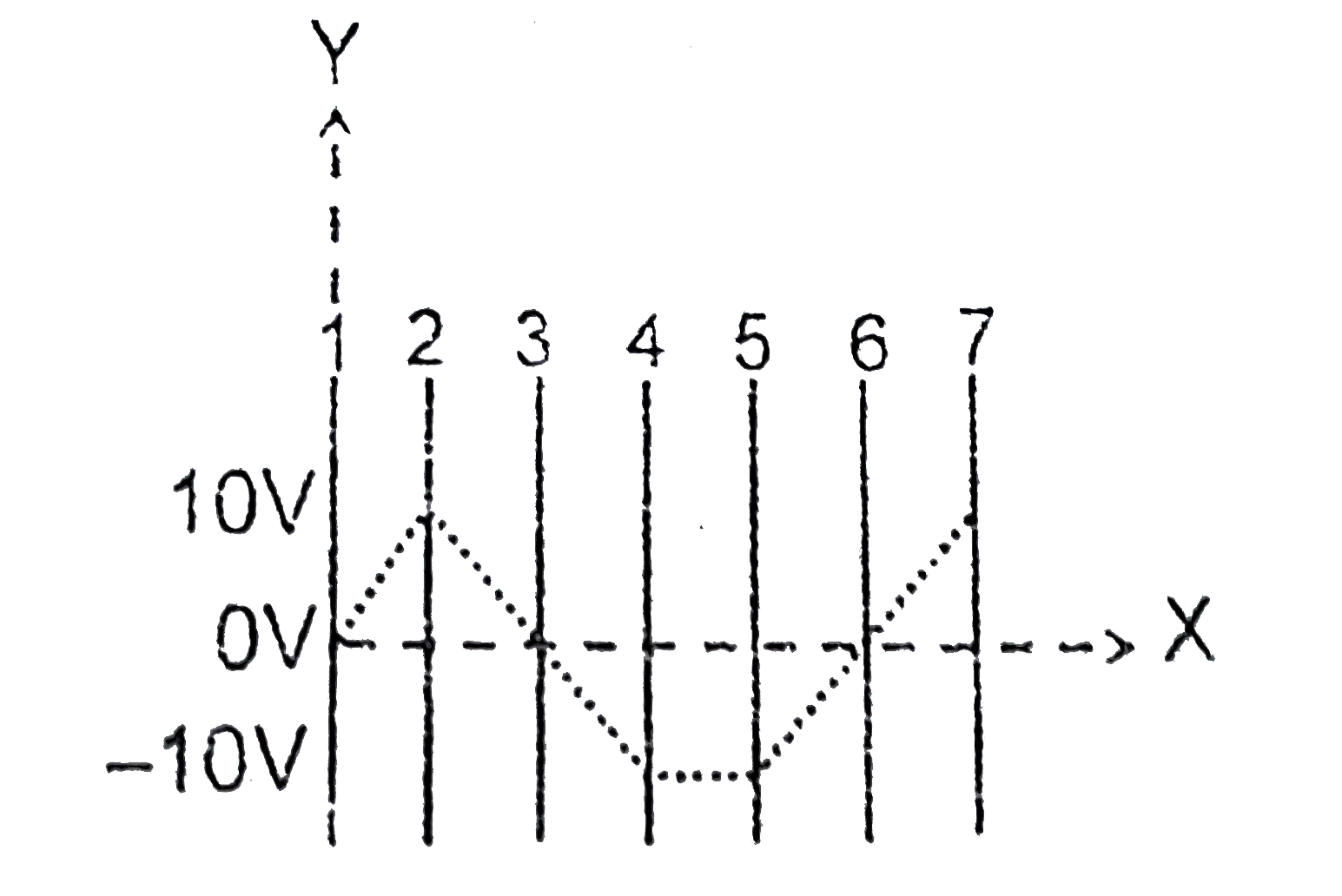
(Separation between the consecutive plates =L, area of each plate =A)A3ε0ALB3ε0A4LC6ε0A5LDε0AL
The equivalent capacitance between A and B is neo A/d. Take plate area as A and separation between the plates as d. Find n. A. .B
View SolutionThe equivalent capacitance between P and Q in the figure is (area of each plate is A and separation between two consecutive plates is d)
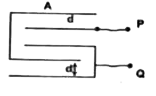
View SolutionFind out equivalent capacitance between A and B. (take each plate Area = A and distance between two conjugative plates is d)
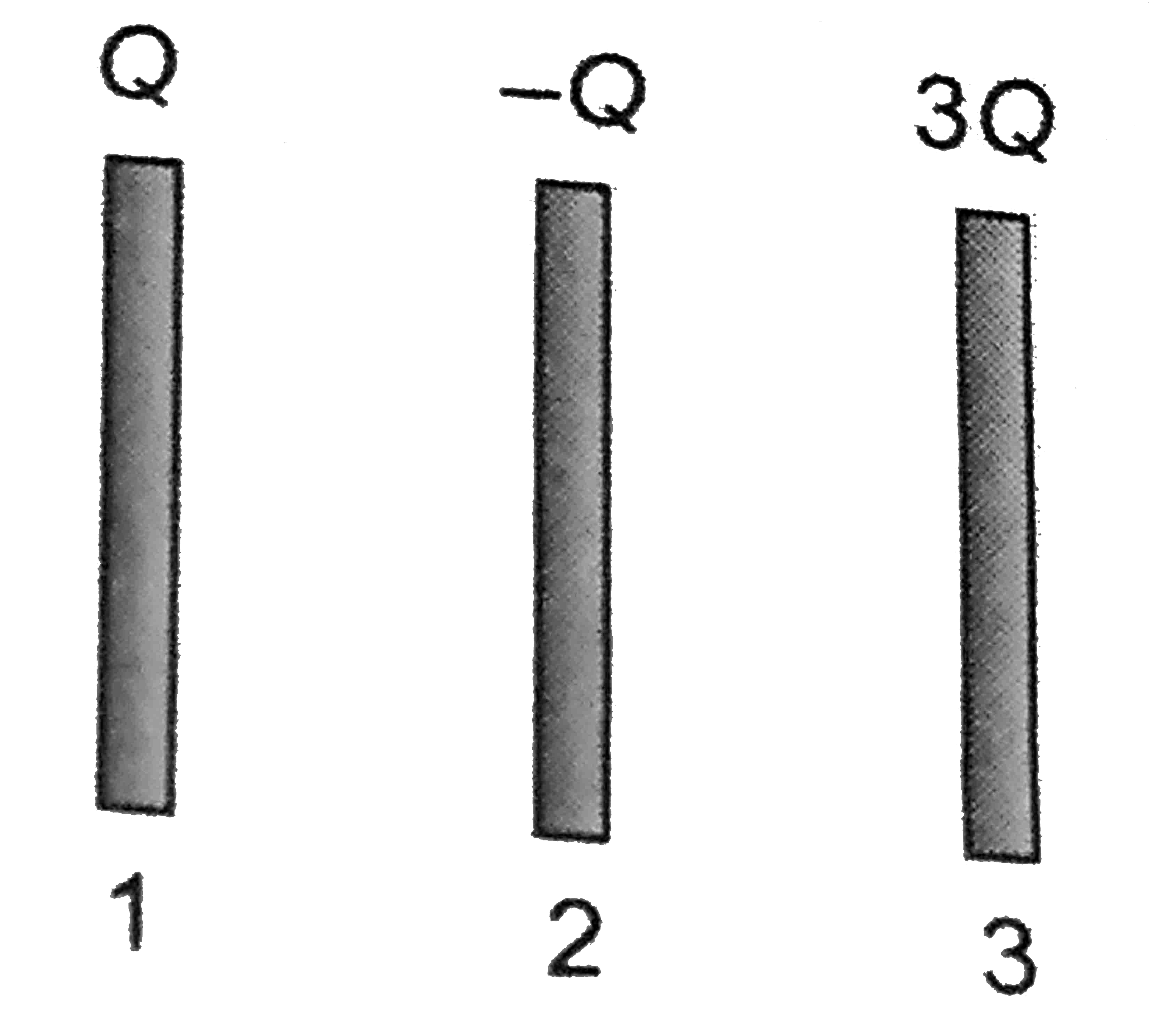
View Solution(a) Find the potential difference between the plates of a parallel plate capacitor of capacitance 5μF if a charge +800μC is placed on one plate and-200μC on another plate.
(b) The charege given to plate are as shown. The capacitance between the adjacent plates is C. find the charge on outer surface of plate 3, and potential difference between plate 1 and 2.
(c) If A is area of each plate and d is separation between adjacent plates, find the charge supplied by battery.
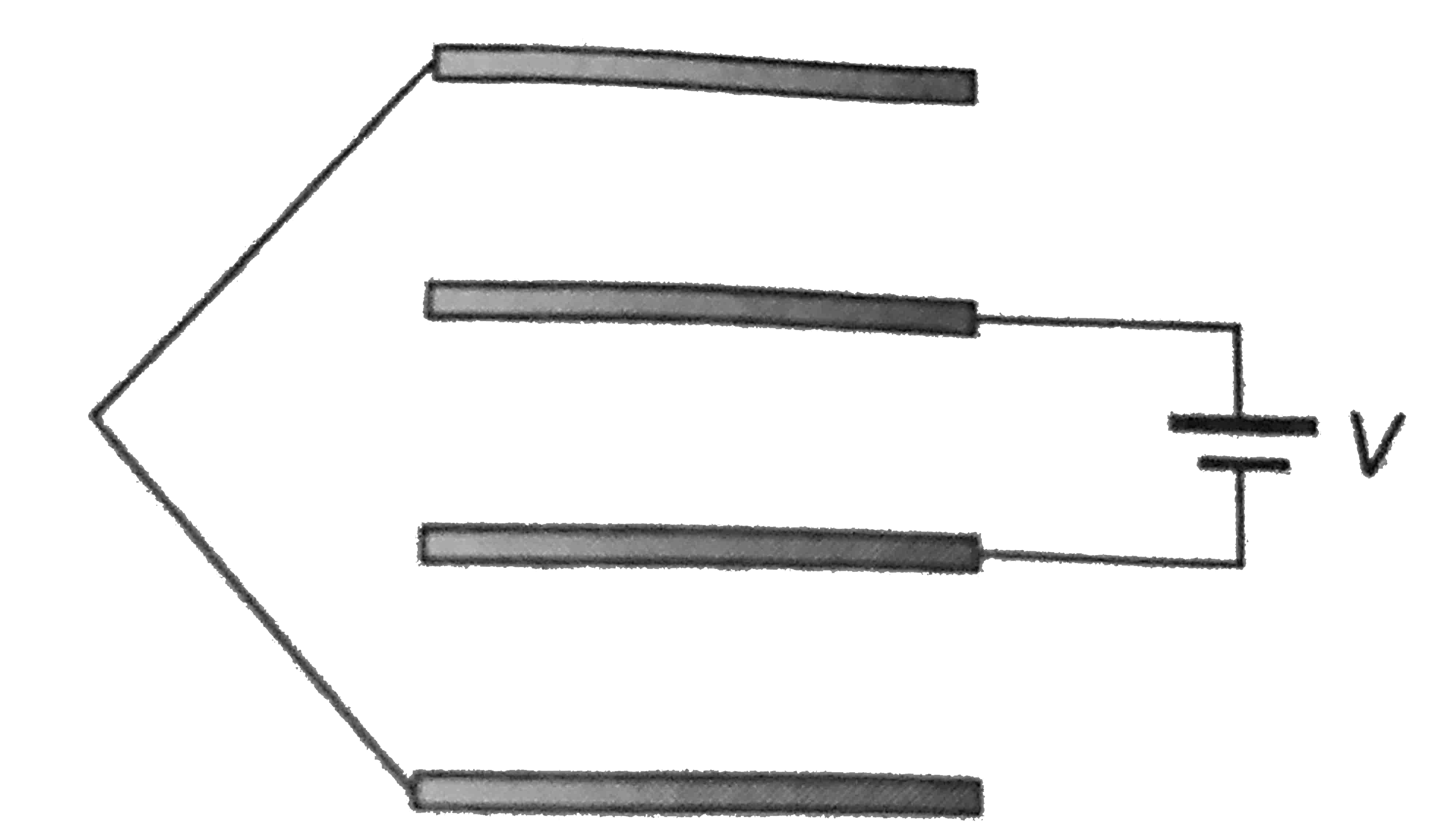
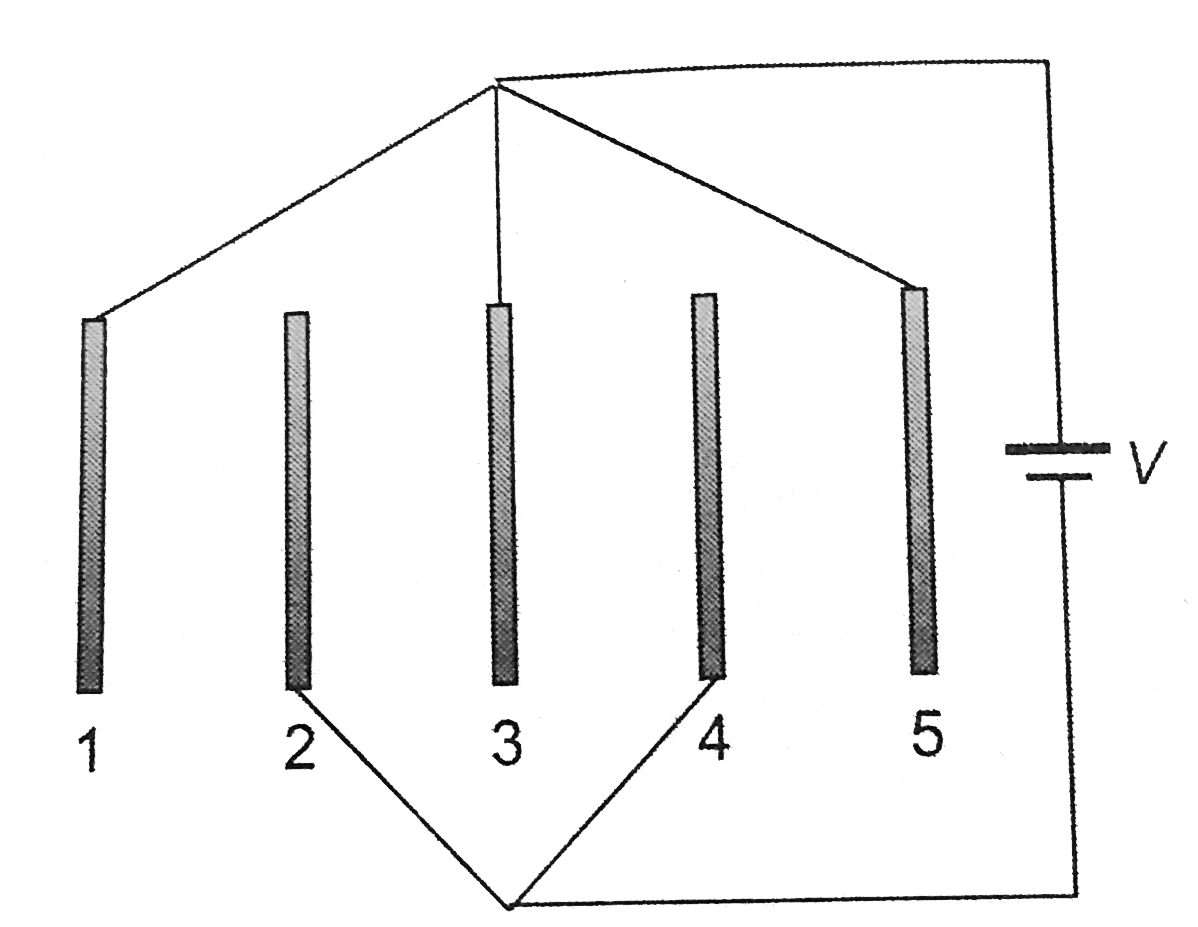
(d) Five idential capacitor plates each of area A are arranged such that adjacent plates are at a distance d apart. the plates are connected to a source of emf V as shown. what is the magnitude and nature of charge on plates 1 and 3 respectively?
View SolutionFind the charge on each plate given area of each plate is A and separation between two consecutive plates is d.

View SolutionFind the equivalent capacitance between point a and b in the following arrangement of the plates .

View Solution