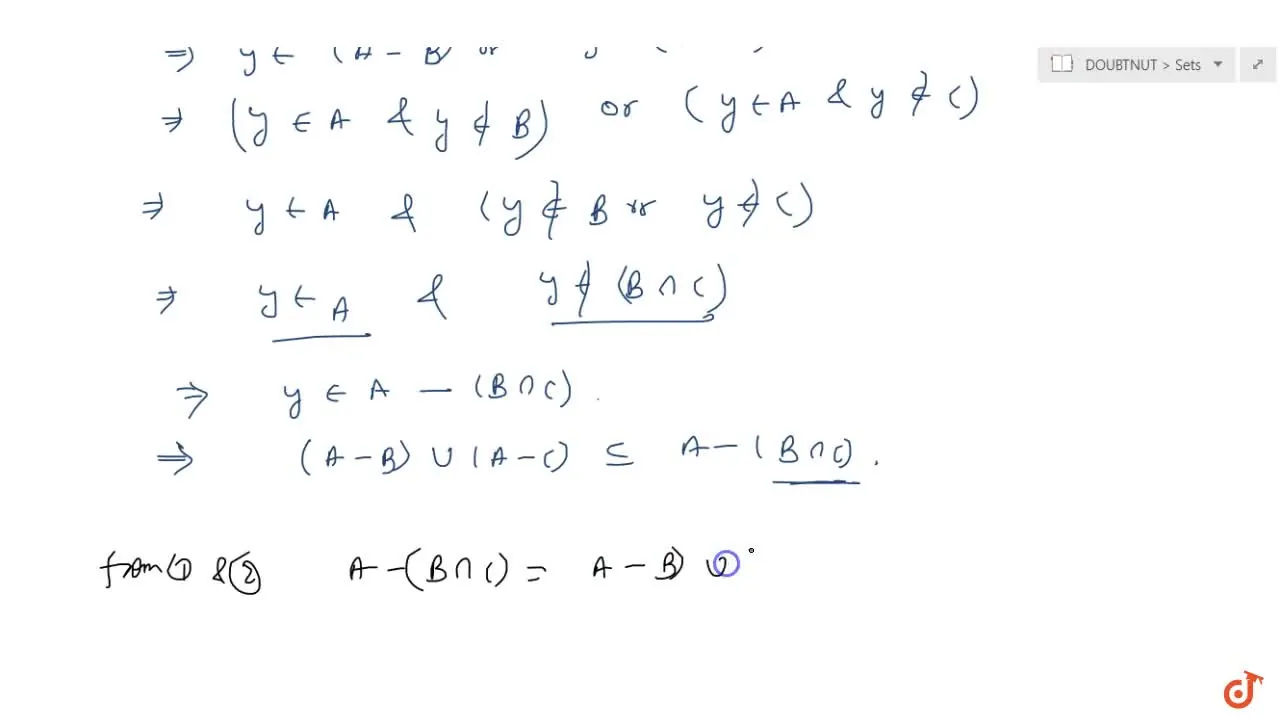If A and B are two sets then A−(B∩C)=(A−B)∪(A−C)
Video Solution
|
Answer
Step by step video & image solution for If A and B are two sets then A-(BnnC)=(A-B)uu(A-C) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Similar Questions
If A and B are two sets then A−(B∩C)=(A−B)∪(A−C)
View SolutionIf A and B are two sets then A−(B∪C)=(A−B)∩(A−C)
View SolutionIf A and B are two sets then (A−B)∪(B−A)=(A∪B)−(A∩B)
View SolutionIf AandB are two sets, then (A−B)∪(B−A)∪(A∩B) is equal to A∪B (b) A∩B (c) A (d) B
View SolutionIf A and B are two sets ,then (A−B)∪(B−A)∪(A∩B) equals
View SolutionIf A and B are any two sets then (A−B)∪B=A∪B
View SolutionIf A and B are two sets, then A∩(A∪B) equals
View SolutionIf A and B are two sets, then A∩(A∪B) equals
View SolutionIf A and B are two sets then prove A=A∩(A∪B).
View SolutionIf A and B are two sets, then (A∪B)'∪(A'∩B) is equal to
View Solution