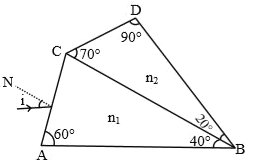
A prism of refractive index n1 & another prism of reactive index n2 are stuck together without a gap as shown in the figure.The angle of the prisms are as shown. n1&n2depend onλ,the wavelength of light according to n1=1.20+10.8×104λ2&n2=1.45+1.80×104λ2 where λ is in nm.

(i)Calculate the wavelength λ0for which rays incident at any angle on the interfaceBCpass through without bending at that interface.
(ii) for light of wavelength λ0,find the angle of incidencei on faceACsuch that the deviation produced by the combination of prism is minimum.
(a) The ray incident on BC passes undeflected through it
If n1=n2 for wavelength λ0
1.20+10.8×104λ20=1.45+1.8×104λ20
(10.8−1.8)×104λ20=1.45−1.20
λ20=9×1040.25⇒λ0=3×1020.5=600nm
(b) For λ0=600nm, n1=n2=1.2+10.8×104(600)2=1.5
Now complete the prism, angle of prism, A=60∘

When angle of deviation is minimum, r=A/2=30∘
Refraction on AC: 1×sini=μ×sinr
sini=1.5×sin30∘=0.75
i=sin−1(0.75)
Topper's Solved these Questions
- REFRACTION OF LIGHT BY PLANE SURFACESBOOK - CP SINGHCHAPTER - REFRACTION OF LIGHT BY PLANE SURFACESEXERCISE - Exercises
110 Videos
- REFRACTION AT SPHERICAL SURFACESBOOK - CP SINGHCHAPTER - REFRACTION AT SPHERICAL SURFACESEXERCISE - EXERCISES
105 Videos
- SEMICONDUCTORSBOOK - CP SINGHCHAPTER - SEMICONDUCTORSEXERCISE - Exercises
140 Videos
Similar Questions
A prism of refractive index n1 and another prism of refractive index n2 are stuck together (as shown in the figure). n1andn2 depend on λ, the wavelength of light, according to the relation
n1=1.2+10.8×10−14λ2andn2=1.45+1.8×10−14λ2
The wavelength for which rays incident at any angle on the interface BC pass through without bending at that interface will be _________ nm.
View Solution∫423x2+1(x2−1)3dx=λn2 where λ,n∈N and gcd(λ,n)=1, then find the value of λ+n
View Solution
Knowledge Check
A small angled prism of refractive index 1.4 is combined with another small angled prism of refractive index 1.6 to produce disperison without deviation. If the angle of first prism is 6(∘), then the angle of the second prism is
A8∘B6∘C4∘D2∘A ray of light travels from a medium of refractive index n1 to
a medium of refractive index n2. If angle of incidence is i and
angle of refraction is r, than sinisinr is equal toAa n1Bb n2Cc n2n1Dd n1n2The wavelength of light in vacuum is λ. The wavelength of light in a medium of refractive index n will be
AnλBλnCλn2Dn2λ
If (√2n2+n−λ√2n2−n)=1√2 (where λ is real number),then
View SolutionA ray of light undergoes deviation of 30∘ when incident on a n equilateral prism of refractive index √2. The and made by the ray inside the prism with the base of the prism is ________________.
View Solution
A right angled triangular prism of refractive index μ1 is kept floating on a liquid (surface) having refractive index μ2. The condition such tat light incident normally passes through base of prism as shown in figure.
View SolutionWhen light of wavelength λ on an equilateral prism, kept on its minimum deviation position, it is found that the angle of deviation equals the angle the angle of the prism itself. The refractive index of the material of the prism for the wavelength λ is
View SolutionA prism having an apex angle of 4∘ and refractive index of 1.50 is located in front of a vertical plane mirror as shown in the figure. A horizontal ray of light is incident on the prism. The total angle through which the ray is deviated is:
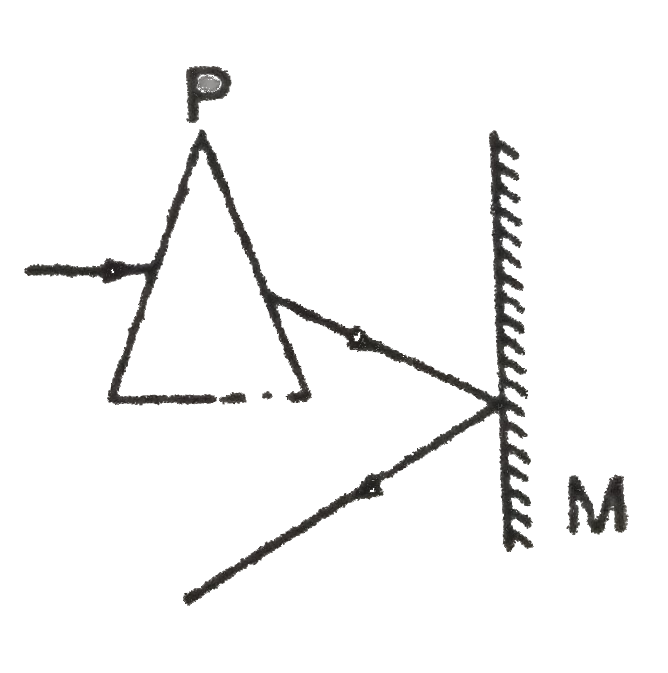
View Solution
