A block of weight 100N is lying on a rough horizontal surface.If coefficient of friction 1√3.The least possible force that can move the block is
100√3N
100√3N
50√3N
50N
The correct Answer is:D
To solve the problem of finding the least possible force that can move a block of weight 100 N on a rough horizontal surface with a coefficient of friction of 1√3, we can follow these steps:
1. Identify the Given Values:
- Weight of the block, W=100N
- Coefficient of friction, μ=1√3
2. Understand the Forces Acting on the Block:
- The weight of the block acts downwards.
- The normal force N acts upwards.
- A horizontal force F is applied at an angle θ to reduce the frictional force.
3. Resolve the Applied Force F:
- The horizontal component of the force is Fcosθ.
- The vertical component of the force is Fsinθ.
4. Calculate the Normal Force:
- The normal force N can be expressed as:
N=W−Fsinθ=100−Fsinθ
5. Determine the Frictional Force:
- The frictional force f is given by:
f=μN=μ(100−Fsinθ)
- Substituting μ:
f=1√3(100−Fsinθ)
6. Set Up the Equation for Motion:
- For the block to just start moving, the applied force's horizontal component must equal the frictional force:
Fcosθ=1√3(100−Fsinθ)
7. Rearranging the Equation:
- Rearranging gives:
Fcosθ+1√3Fsinθ=100√3
- Factoring out F:
F(cosθ+1√3sinθ)=100√3
8. Maximize the Denominator:
- To minimize F, we need to maximize cosθ+1√3sinθ.
- The maximum value of cosθ+μsinθ can be found using the formula:
√1+μ2
- Substituting μ=1√3:
⎷1+(1√3)2=√1+13=√43=2√3
9. Calculate the Least Possible Force F:
- Substitute back into the equation for F:
F=100/√32√3=1002=50N
Final Answer:
The least possible force that can move the block is F=50N.
- Weight of the block, W=100N
- Coefficient of friction, μ=1√3
- The weight of the block acts downwards.
- The normal force N acts upwards.
- A horizontal force F is applied at an angle θ to reduce the frictional force.
- The horizontal component of the force is Fcosθ.
- The vertical component of the force is Fsinθ.
- The normal force N can be expressed as:
N=W−Fsinθ=100−Fsinθ
- The frictional force f is given by:
f=μN=μ(100−Fsinθ)
- Substituting μ:
f=1√3(100−Fsinθ)
- For the block to just start moving, the applied force's horizontal component must equal the frictional force:
Fcosθ=1√3(100−Fsinθ)
- Rearranging gives:
Fcosθ+1√3Fsinθ=100√3
- Factoring out F:
F(cosθ+1√3sinθ)=100√3
- To minimize F, we need to maximize cosθ+1√3sinθ.
- The maximum value of cosθ+μsinθ can be found using the formula:
√1+μ2
- Substituting μ=1√3:
⎷1+(1√3)2=√1+13=√43=2√3
- Substitute back into the equation for F:
F=100/√32√3=1002=50N
The least possible force that can move the block is F=50N.
Topper's Solved these Questions
Similar Questions
A block of mass M is kept on as rough horizontal surface. The coefficient of static frictionbetween the block and the surface is μ. The block is to be pulled by applying a force to it. What minimum force is needed to slide the block? In which direction should this force act?
View SolutionA weight W rests on a rough horizontal plane,If the angle of friction is θ,the least force that can move the body along the plane will be
View Solution
Knowledge Check
A block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?
 A(M+m)(μ2−μ1)gB(M−m)(μ2−μ1)gC(M−m)(μ2+μ1)gD(M+m)(μ2+μ1)g
A(M+m)(μ2−μ1)gB(M−m)(μ2−μ1)gC(M−m)(μ2+μ1)gD(M+m)(μ2+μ1)gA cubical block of side L rests on a rough horizonta surface with coefficient of friction μ. A horizontal force F is applied on the block as shown. If the coefficient of friction is sufficiently high so that the block does not slide before toppling, the minimum force required to topple the block is
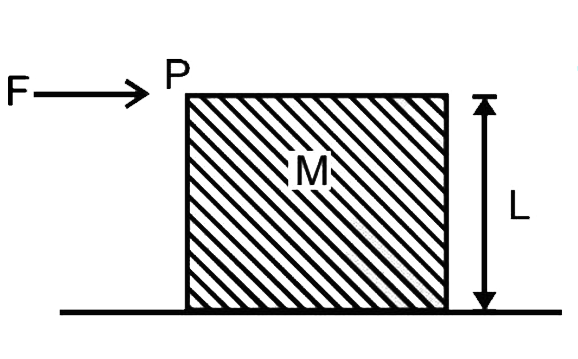 AInfinitesimalBmg/4Cmg/2Dmg(1−μ)
AInfinitesimalBmg/4Cmg/2Dmg(1−μ)A cubical block of side L rests on a rough horizontal surface with coefficient of friction μ. A horizontal force F is applied on the block as shown. If the coefficient of frictions sufficienty high so that the block does not slide before toppling, the minimum force required to topple the block is :
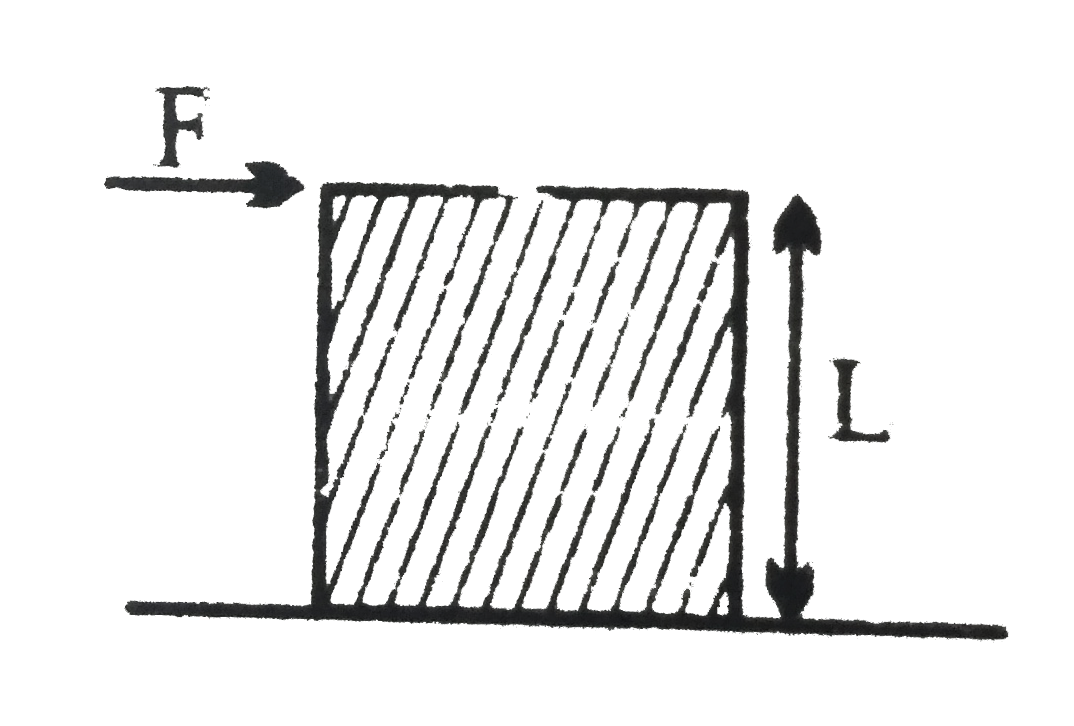 . AinfinitesimalBmg/4Cmg/2Dmg(1−μ)
. AinfinitesimalBmg/4Cmg/2Dmg(1−μ)
A block of mass M is being pulley along horizontal surface .The coefficient of friction the block and the surface is μ If another block of mass M/2 is placed on the block and it is pulled is again pulled on the surface , the coefficient of friction the block and the surface will be
View SolutionA cubical block of side L rests on a rough horizonta surface with coefficient of friction μ. A horizontal force F is applied on the block as shown. If the coefficient of friction is sufficiently high so that the block does not slide before toppling, the minimum force required to topple the block is

View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a force of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionA block of mass M is using on a rough horizontal surface. μR is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View Solution