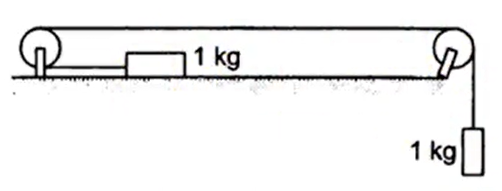
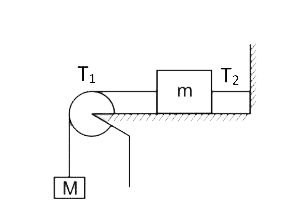
Consider the system as shown in the figure. The pulley and the string are light and all the surfaces are frictionless. The tension in the string is (g=10m/s2) .

0N
1N
2N
5N
The correct Answer is:D
For the block on the surface T=m1a
For the hanging block m2g−T=m2a
Topper's Solved these Questions
Similar Questions
Consider4 the situation shown in figure. Bothe the pulleys and the string are light and all the surfaces are friction less. a. Find the acceleration of the mass M. b. Find the tension in the string c. Calculate the force exerted by the clamp on the pulley A in the figure.

View SolutionCalculate the tension in the string shown in figure. The pulley and the string are light and all surfaces are frictionless. Take g=10 m/s2.
View Solution
Knowledge Check
Consider the system as shown in the figure. The pulley and the string are light and all the surfaces are frictionless. The tension in the string is (g=10m/s2) .
 A0NB1NC2ND5N
A0NB1NC2ND5NConsider the system shown in figure. The pulley and the string are light and all the surface are frictionless. The tension in the string is (Take g=10m/s2)
 A0NB1NC2ND5N
A0NB1NC2ND5NFor the system shown in the figure, the pulleys are light and frictionless. The tension in the string will be (Assume all surfaces to be smooth)
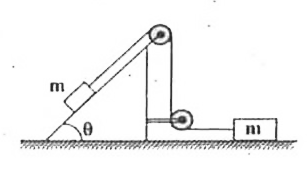 A23mgsinθB32mgsinθC12mgsinθD2mgsinθ
A23mgsinθB32mgsinθC12mgsinθD2mgsinθ
Consider the situation shown in figure. Both the pulleys and the strings are light and all the surfaces are frictionless. Calculate
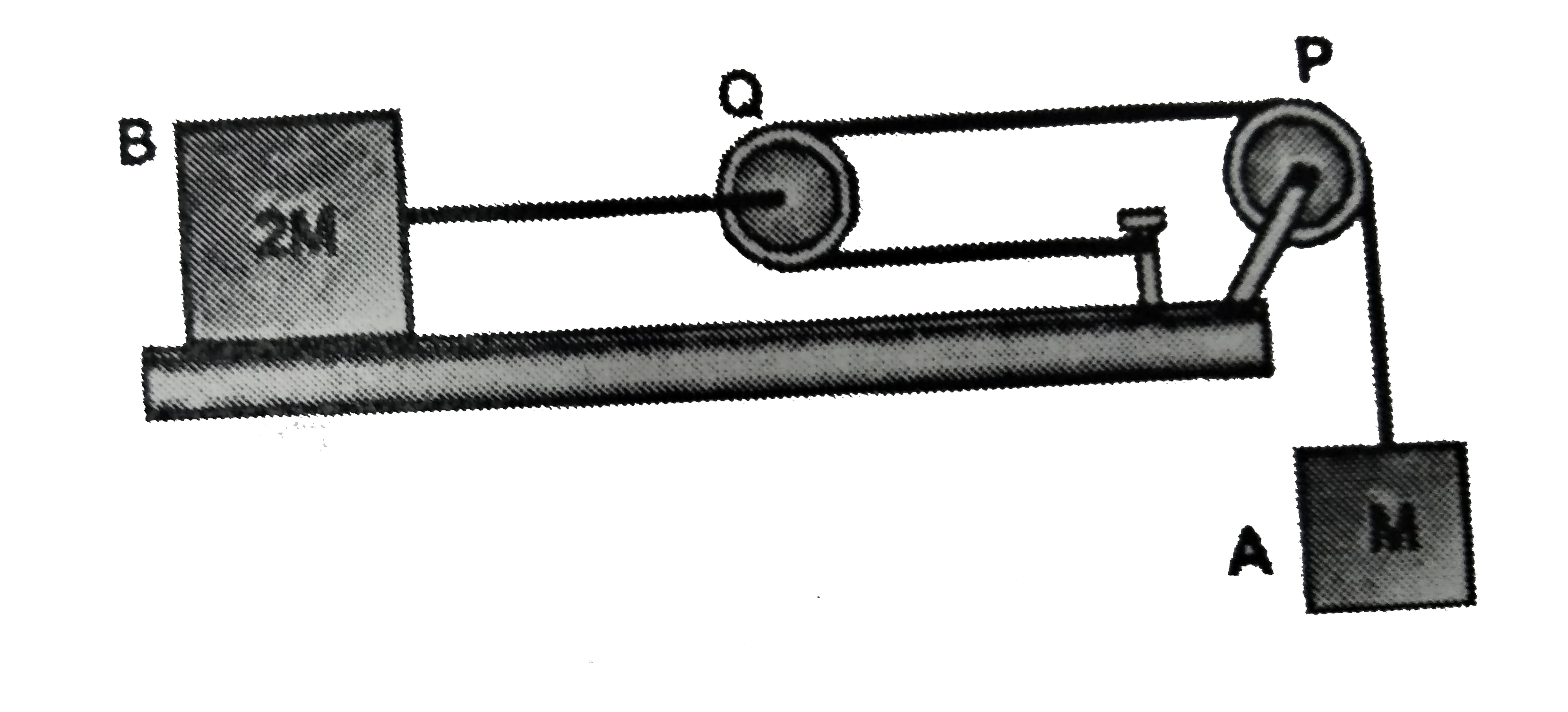
(a) the acceleration of mass M,
(b) tension in the string PQ and
(c ) force exerted by the pulley P.
View SolutionIn the situation shown in figure, both the pulleys and the strings are light and all the surfaces are frictionless. The acceleration of mass M, tension in the string PQ and force exerted by the clamp on the pulley, will respectively be -
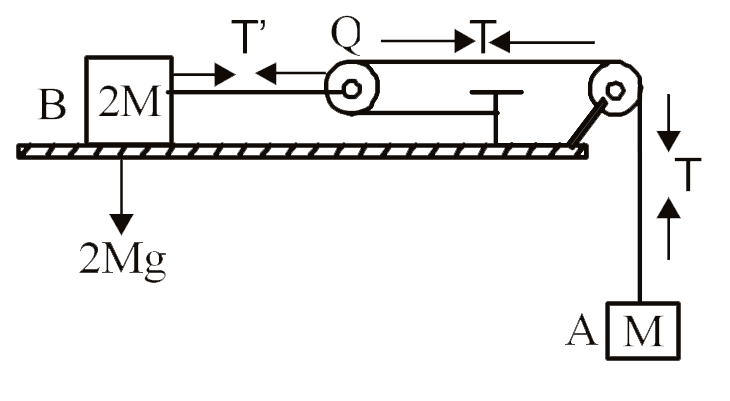
View SolutionFor the system shown in figure, the tensions in the string
View SolutionCalculate the tension in the string shown in
(a) The pulleys and the string are light and all surfaces are frictionless. (g=10m/s2)
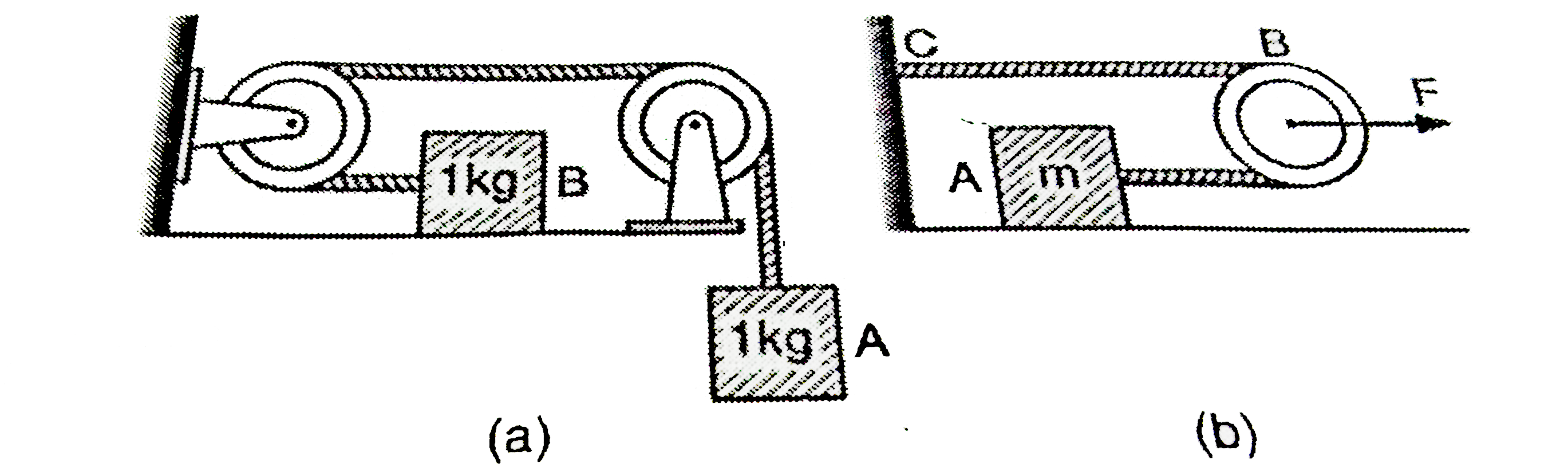
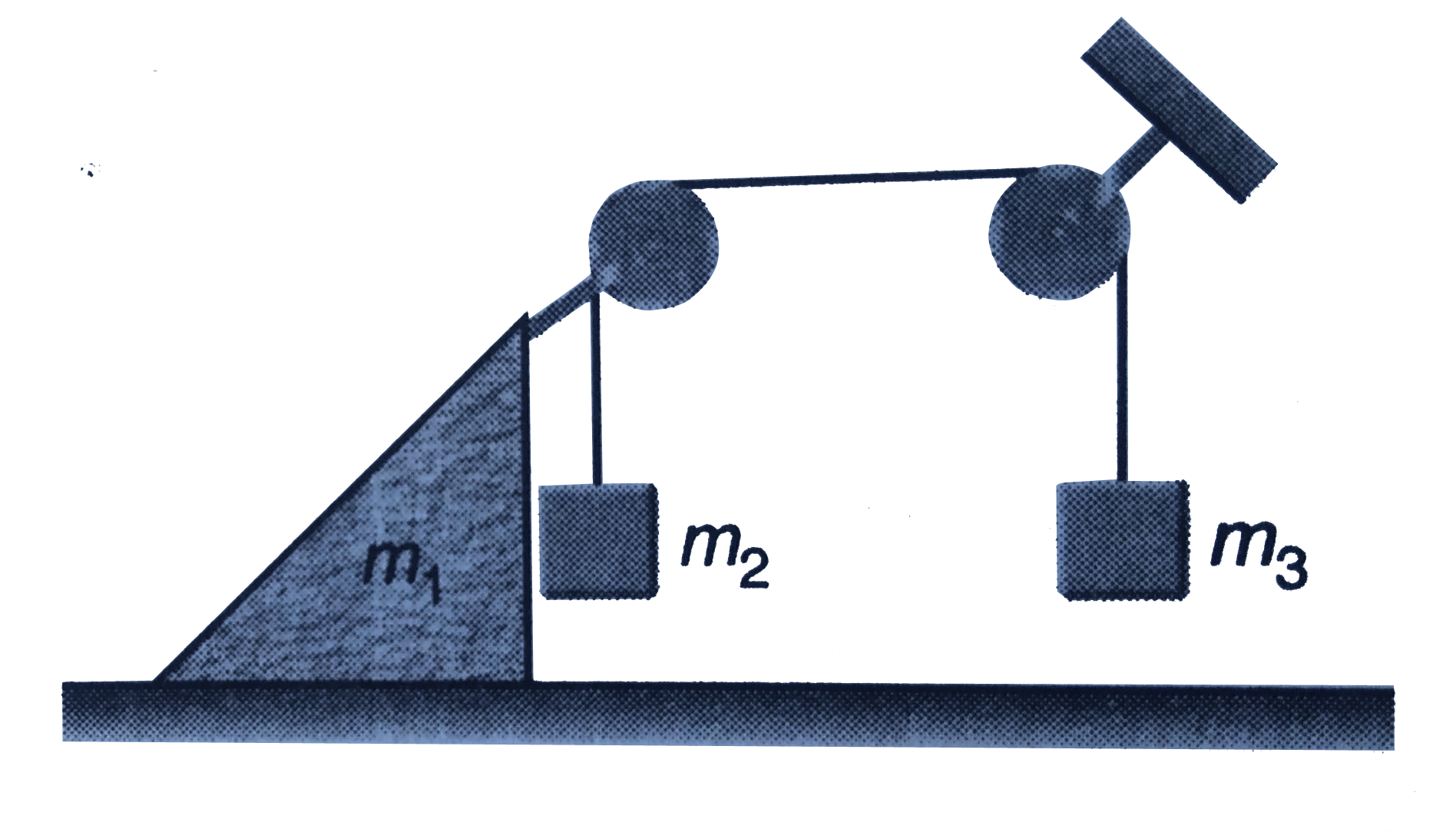
View SolutionIn the shown arrangement , both pulleys and the string are massless and all the surface are frictionless. Find the acceleration of the wedge.
View Solution
