A thin brass sheet at 10∘C and a thin steel sheet at 20∘C have the same surface area. The common temperature at which both would have the same area is (Coefficient of linear expansion for brass and steel are respectively, 19×10−6/∘C are 11×10−6/∘C)
−3.75∘C
−2.75∘C
2.75∘C
3.75∘C
The correct Answer is:A
To solve the problem, we need to find the common temperature at which the areas of the brass and steel sheets become equal after thermal expansion.
1. Identify Initial Conditions:
- Let the initial temperature of the brass sheet, T1=10∘C.
- Let the initial temperature of the steel sheet, T2=20∘C.
- Both sheets have the same initial area, A1=A2.
2. Define the Coefficients of Linear Expansion:
- Coefficient of linear expansion for brass, α1=19×10−6/∘C.
- Coefficient of linear expansion for steel, α2=11×10−6/∘C.
- The area expansion coefficient β is related to the linear expansion coefficient α by β=2α.
3. Write the Area Expansion Formula:
- The area of the brass sheet at temperature T will be:
A′1=A1(1+β1(T−T1))=A1(1+2α1(T−10))
- The area of the steel sheet at temperature T will be:
A′2=A2(1+β2(T−T2))=A2(1+2α2(T−20))
4. Set the Areas Equal:
- Since A′1=A′2, we can set the equations equal to each other:
A1(1+2α1(T−10))=A2(1+2α2(T−20))
- Since A1=A2, we can cancel A1 and A2:
1+2α1(T−10)=1+2α2(T−20)
5. Simplify the Equation:
- Cancel the 1s from both sides:
2α1(T−10)=2α2(T−20)
- Divide by 2:
α1(T−10)=α2(T−20)
6. Substitute the Values of Coefficients:
- Substitute α1 and α2:
19×10−6(T−10)=11×10−6(T−20)
7. Eliminate the Common Factor:
- Divide both sides by 10−6:
19(T−10)=11(T−20)
8. Expand and Collect Terms:
- Expand both sides:
19T−190=11T−220
- Rearranging gives:
19T−11T=−220+190
8T=−30
9. Solve for T:
- Divide by 8:
T=−308=−3.75∘C
Final Answer:
The common temperature at which both sheets would have the same area is T=−3.75∘C.
- Let the initial temperature of the brass sheet, T1=10∘C.
- Let the initial temperature of the steel sheet, T2=20∘C.
- Both sheets have the same initial area, A1=A2.
- Coefficient of linear expansion for brass, α1=19×10−6/∘C.
- Coefficient of linear expansion for steel, α2=11×10−6/∘C.
- The area expansion coefficient β is related to the linear expansion coefficient α by β=2α.
- The area of the brass sheet at temperature T will be:
A′1=A1(1+β1(T−T1))=A1(1+2α1(T−10))
- The area of the steel sheet at temperature T will be:
A′2=A2(1+β2(T−T2))=A2(1+2α2(T−20))
- Since A′1=A′2, we can set the equations equal to each other:
A1(1+2α1(T−10))=A2(1+2α2(T−20))
- Since A1=A2, we can cancel A1 and A2:
1+2α1(T−10)=1+2α2(T−20)
- Cancel the 1s from both sides:
2α1(T−10)=2α2(T−20)
- Divide by 2:
α1(T−10)=α2(T−20)
- Substitute α1 and α2:
19×10−6(T−10)=11×10−6(T−20)
- Divide both sides by 10−6:
19(T−10)=11(T−20)
- Expand both sides:
19T−190=11T−220
- Rearranging gives:
19T−11T=−220+190
8T=−30
- Divide by 8:
T=−308=−3.75∘C
The common temperature at which both sheets would have the same area is T=−3.75∘C.
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - III
23 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - NCERT BASED QUESTIONS
25 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - I (C.W.)
24 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV
39 Videos
- THERMODYNAMICSBOOK - NARAYNACHAPTER - THERMODYNAMICSEXERCISE - Exercise
187 Videos
Similar Questions
What should be the sum of lengths of an aluminium and steel rod at 0∘C is, so that all the temperature their difference in length is 0.25m. (Take coefficient of linear expansion for aluminium and steel at 0∘C as 22×10−6/.∘C and 11×10−5/.∘C respectively.)
View SolutionA steel wire AB of length 85cm at 10∘C is fixed rigidly at points A and B in an aluminium frame as shown. If the temperature of the system is raised to 110∘C , what extra stress will be produced in the wire relative to aluminium frame. Assume that coefficient of linear expansion for aluminium and steel are 23×10−6/∘C and 11×10−6/∘C respectively and Young's moduls for steel is 2×1011 pa.
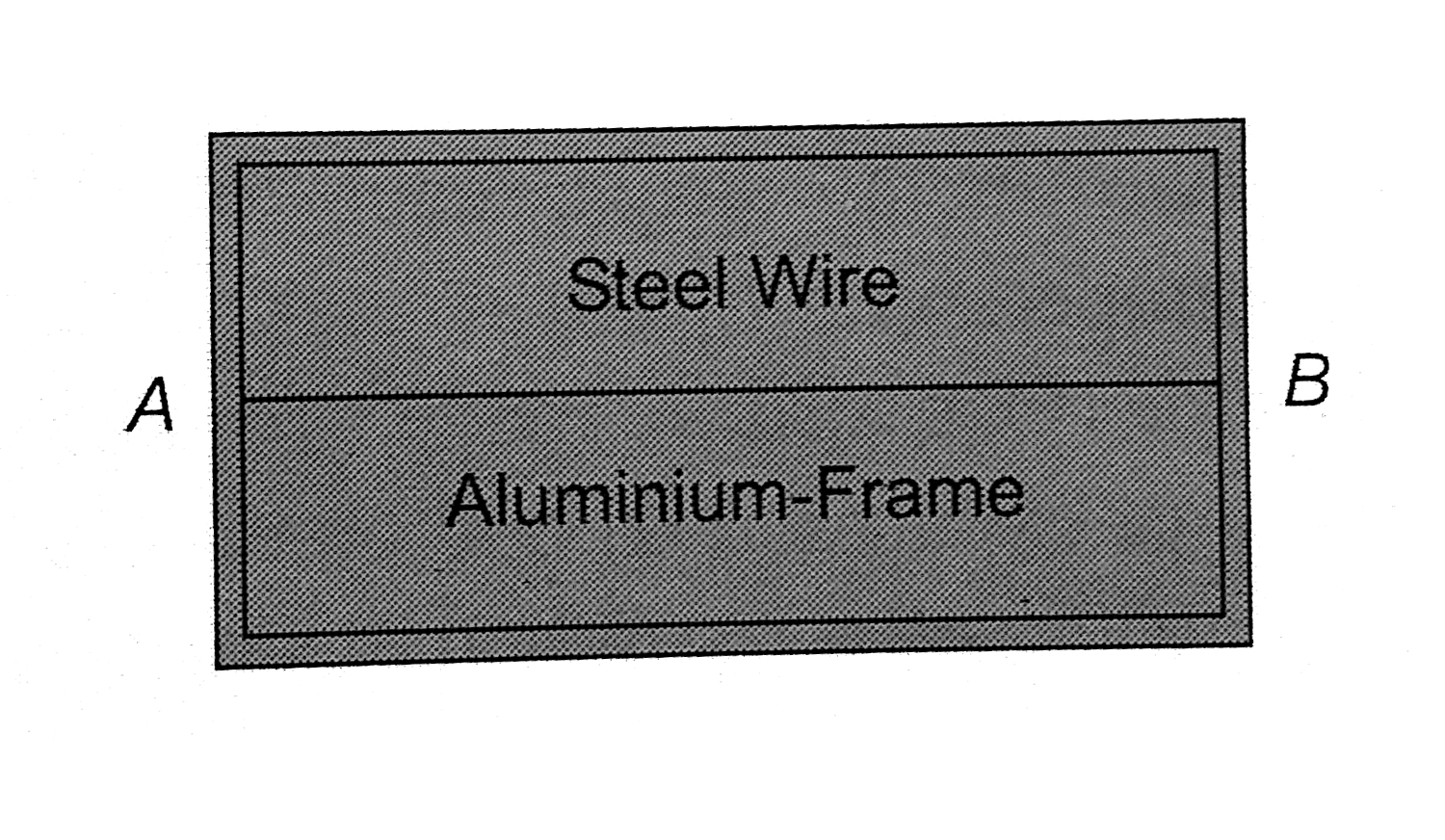
View Solution
Knowledge Check
A thin brass sheet at 20∘C and a thin steel sheet at 30∘C have the same surface area. The common tempreture at which both would have the same area is (Coefficient of linear expansion for brass and steel are respectively, 19×10−6/∘C are 11×10−6/∘C)
A−6.250CB+6.250CC−3.250CD+3.250CA steel scale is correct at 0∘C. The length of a brass tube measured by it at 40∘C is 4.5 m. The correct length of the tube at 0∘C is (Coefficients of linear expansion of steel and brass are 11×10−6/∘Cand19×10−6/∘C respectively.)
A4.001mB5.001mC4.999mD4.501mTwo thin metal strips, one of brass and the other of iron are fastended together parallel to each other. Thickness of each strip is 2mm. If the strips are of equal length at 0∘C. The radius of the are formed by the bimetaalic strip when heated to 80∘C is (Coefficient of linear expansion of brass=19×10−6/∘C&
of iron=12×10−6/∘C).A3.57mB2.67mC3.12mD4.56m
At 40∘C, a brass rod has a length 50 cm and a diameter 3.0 mm. it is joined to a steel rod of the same length and diameter at the same temperature. What is the change in the length of the composite rod when it is heated to 240∘C? The coefficient of liear expansion of brass and steel are 2.0×10−5.∘C−1 and 1.2×10−5.∘C−1 respectively:
View SolutionA brass scale is graduated at 10∘C. What is the true length of a zinc rod which measures 60.00 cm on this scale at 30∘C?
Coefficient of linear expansion of brass=18×10−6K−1.
View SolutionA brass rod length 50 cm and diamteer 3.0 mm is joined to a steel rod of the same length and diameter. What is the change in length of the combined rod at 250∘C if the original length are at 40∘C ? Coefficient of linear expansion of brass and steel are 2.10×10−5.∘C−1 and 1.2×10−5^(∘)C−1 respectively.
View SolutionConsider a brass rod and a steel rod (80 cm longer than brass rod) at 0∘C. It is observed that on increasing temperatures of the two rods by same amount difference in lengths of the two rods does not change. Given that the thermal coefficient of linear expansion for steel and brass are 11×10−6.∘C−1 and 19×10−6.∘C−1 respectively. The sum of lengths of the two rods at 0∘C is
View SolutionIf a cylinder of diameter 1.0cm at 30∘C is to be slid into a hole of diameter 0.9997cm in a steel plate at the same temperature, the minimum required rise in the temperature of the plate is: (Coefficient of linear expansion of steel=12×10−6/∘C)
View Solution