A disc rotates with angular velocity ω and kinetic energy E. Then its angular momentum
Iω
L=Eω
L=2Eω
L=ωE
The correct Answer is:C
KE=12Lω
Topper's Solved these Questions
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level -II(H.W)
47 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-V
72 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - NCERT based questions
15 Videos
- PHYSICAL WORLDBOOK - NARAYNACHAPTER - PHYSICAL WORLDEXERCISE - C.U.Q
10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV
39 Videos
Similar Questions
A thin uniformring of radius R carrying uniform charge Q and mass M rotates about its axis with angular velocity ω. The ratio of its magnetic moment and angular momentum is:
View SolutionA particle performing uniform circular motion gas angular momentum L. If its angular frequency is double and its kinetic energy halved, then the new angular momentum is :
View Solution
Knowledge Check
The body is rotating with uniform angular velocity (ω ) having rotational kinetic energy ( E) . Its angular momentum (L ) is :
A2EωBE2ωCEω2DE2ωA thin disc of radius R and mass M has charge q uniformly distributed on it. It rotates with angular velocity ω. The ratio of magnetic moment and angular momentum for the disc is
Aq2MBR2MCq22MD2MqIf a body of mass 'm' and radius of gyration K rotates with angular velocity ω, then the angular momentum of the body will be
Am2kωBmk2ωCmkω2Dmkω
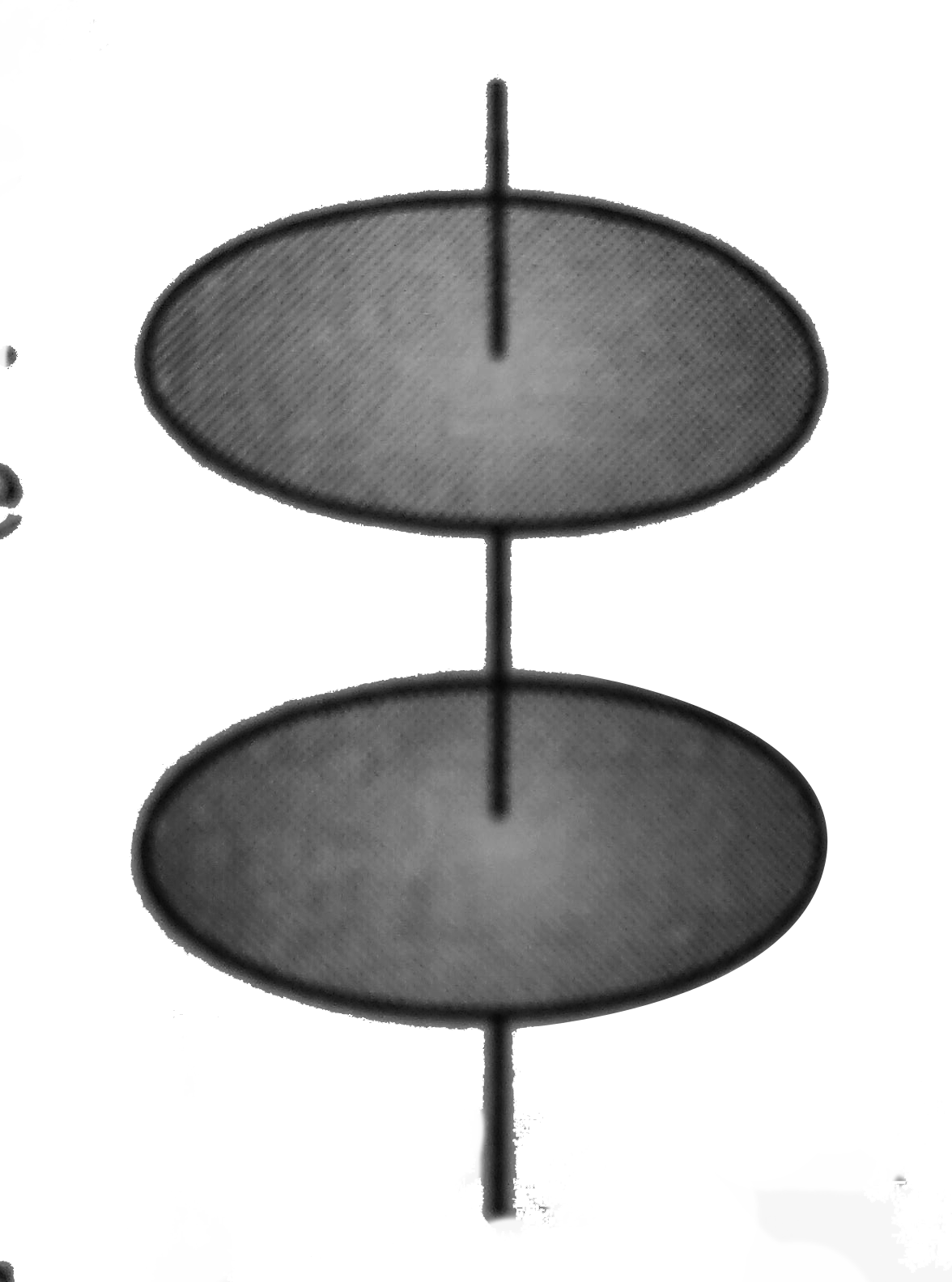
Two identical discs are positioned on a vertical axis as shown in the figure. The bottom disc is rotating at angular velocity ω0 and has rotational kinetic energy K0. Change in the rotational kinetic energy of the system is
View SolutionA horizontal disc rotates freely with angular velocity ω about a vertical axes through its centre. A rig having the same mass and radius as the disc, is now gently placed coaxially on the disc. After some time, the two rotate with a common angular velocity. then.
View SolutionA disc is rotating with angular velocity ω. If a child sits on it, what is conserved?
View SolutionA round disc of moment of inertia I2 about its axis perpendicular to its plane and passing through its centre is placed over another disc of moment of inertia I1 rotating with an angular velocity ω about the same axis. The final angular velocity of the combination of discs is.
View SolutionA skater rotating about a vertical axis pulls her arms inward. Ignoring all frictional effects, which of the following statements are true? Denote the magnitude of her angular velocity by ω, the magnitude of her angular momentum by L, and her kinetic energy by Ek.
View Solution