A disc of moment of inertia 4kgm2 is spinning freely at 3rads−1. A second disc of moment of inertia 2kgm2 slides down the spindle and they rotate together. (a) What is the angular velocity of the combination ? (b) What is the change in kinetic energy of the system?
(a) Since there are no external torques acting, we may apply the conservation of angular momentum,
Ifωf=Iiωi⇒(6kgm2)ωf=(4kgm2)(3rads−1)
Thus ωf=2rads−1
(b) The kinetic energies before and after the collision are
Ki=12Iiω2i,Kf=12Ifω2f=12J
The change is ΔK=Kf−Ki=−6J
In order for the two discs to spin together at the same rate, there had to be friction between them . the loss in kinetic energy is converted into thermal energy.
Similar Questions
A disc is rotating with angular speed ω1. The combined moment of inertia of the disc and its axle is I1. A second disc of moment of inertia I2 is dropped on to the first and ends up rotating with it. Find the angular velocity of the combination if the original angular velocity of the upper disc was (a) zero (b) ω2 in the same direction as ω1 and (c) ω2 in a direction opposite to ω1.
View SolutionA disc of moment of inertia I1 is rotating freely with angular speed ω1 when another non-rotating disc of moment of inertia I2 is dropped on it. The two discs then rotate as one unit. Find the final angular speed.
View Solution
Knowledge Check
A body of moment of inertia 2kg-m^(2) has a rotational kinetic energy of 4 J. What is the angular momentum of the body ?
A√2 kg m2/sB2 kg m2/sC4 kg m2/sD8 kg m2/sA disc of moment of inertia 2kg−m23 revolving with 8rad/s is placed on anothere disc of moment of inertia 4kg−m2 revolving 4rad/s.What is the angular frequency of composite disc?
A4rad/sB316rad/sC163rad/sD165rad/sA disc of moment of inertia I1 is rotating freely with angular speed ω1 when another non-rotating disc of moment of inertia I2 is dropped on it. The two discs then rotate as one unit. Find the final angular speed.
AI1ω1I2BI2ω1I2+I2CI1ω2I1+I2D(I1+I2)I2ω1
An ice skater having moment of inertia l rotating with angular speed ω suddenly opens her arms, which reduces her angular velocity to ω4. Calculate the change in moment of inertia of the dancer.
View SolutionA fan of moment of inertia 0.6kgm2 is moving with a speed of 0.5 r.p.s. The angular momentum of the fan is
View SolutionA disc of moment of inertia 3kgm2 is acted upon by a constant torque of 60 Nm, if the disc is at rest, then the time after which the angular velocity of the disc is 90 rad/s, will be
View SolutionA disc of mass 4.8 kg and radius 1 m is rolling on a horizontal surface without sliding with angular velocity of 600 rotations/min. What is the total kinetic energy of the disc ?
View Solution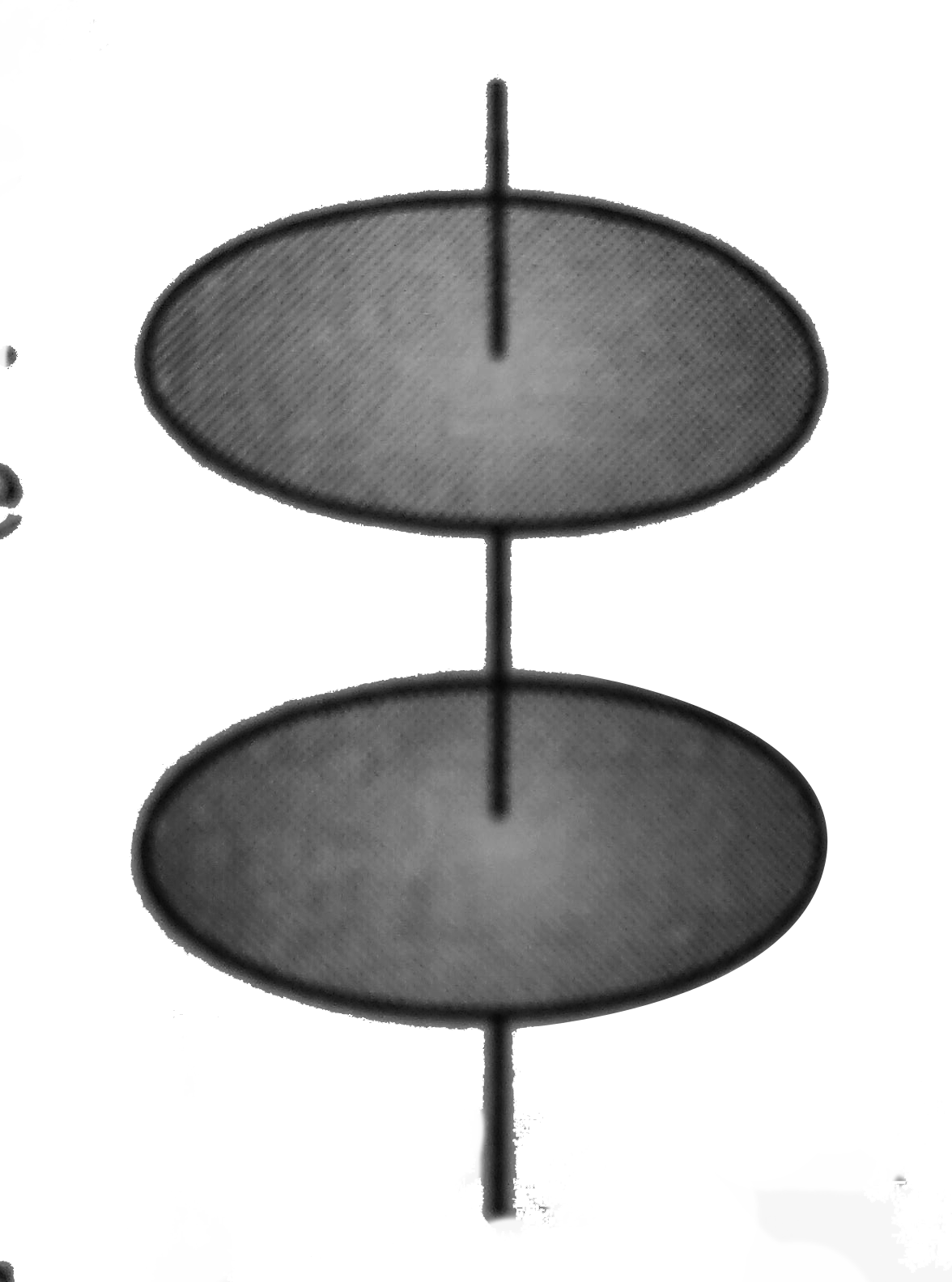
Two identical discs are positioned on a vertical axis as shown in the figure. The bottom disc is rotating at angular velocity ω0 and has rotational kinetic energy K0. Change in the rotational kinetic energy of the system is
View Solution
