In the previous question let V0 be the volume of each container. All other details remain the same. The number of moles of gas in the container at temperature 2T0 will be
p0V02RT0
p0V0RT0
2p0V03RT0
p0V03RT0
The correct Answer is:D
pV0R.2T0=4p0V03R.2T0=2p0V02RT0
Topper's Solved these Questions
Similar Questions
Equal volume of all gases always contains equal number of moles.
View SolutionTwo containers are filled with ideal gases at the same temperature. In the container on the left is a gas of molar mass 2M, volume 2V, and number of moles ⋅2n. In the container on the right is a gas of molar mass M, voluine V, arid moles n. The ratio of the pressure of the gas on the left to- the pressure of the gas on the right container is
'(##CEN_KSR_PHY_JEE_C13_E01_024_Q06##)'View Solution
Knowledge Check
- Question 1 - Select One
In the previous questions, if the mass of the body is half the mass of the earth, and all other data remain the same, then t is equal to
A√2H/gB√H/gC√2H/3gD√4H/3g - Question 2 - Select One
Two identical container each of volume V0 are joined by a small pipe. The containers contain identical gases at temperature T0 and pressure P0. One container is heated to temperature 2T0 while maintaining the other at the same temperature. The common pressure of the gas is P and n is the number of moles of gas in container at temperature 2T0.
AP=2P0BP=43P0Cn=2P0V03RT0Dn=2P0V02RT0 - Question 3 - Select One
Two cylinder A and B having piston conneted by massless rod (as shown in figure). The cross-sectional area two cylinders are same & equal to 'S'.The cylinder A contains m gm of an ideal gas at Pressure P & temperature T0 The cylinder B contain identical gas at same temperature T0 but has different mass. The piston is held at the state in the position so that volume of gas in cylinder A & cylinder B are same and is equal to V0. The walls & piston of cylinder A are thermally insulated, whereas cylinder B is maintained at temperature T0 reservoir. The whole system is in vacuum. Now the piston is slowly released and it moves towards left & machanical equilibrium is reached at the state when the volume of gas in cylinder A becomes V02 Then (here gamma for gas =1.5)
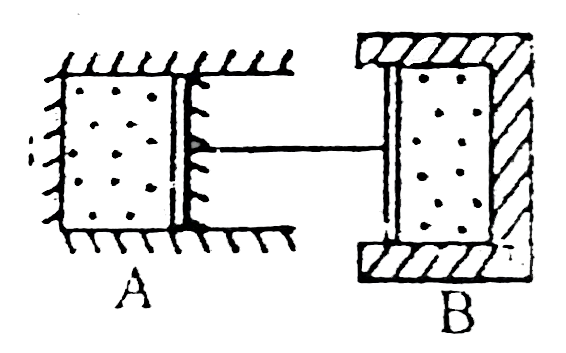
If work done by gas in cylinder B is WB & work done by gas in cylinder A is WA thenAWA=−WBB|WA|>|WB|C|WA|<|WB|Dwe can't say anything
Two cylinder A and B having piston conneted by massless rod (as shown in figure). The cross-sectional area two cylinders are same & equal to 'S'.The cylinder A contains m gm of an ideal gas at Pressure P & temperature T0 The cylinder B contain identical gas at same temperature T0 but has different mass. The piston is held at the state in the position so that volume of gas in cylinder A & cylinder B are same and is equal to V0. The walls & piston of cylinder A are thermally insulated, whereas cylinder B is maintained at temperature T0 reservoir. The whole system is in vacuum. Now the piston is slowly released and it moves towards left & machanical equilibrium is reached at the state when the volume of gas in cylinder A becomes V_(0)/2 Then (here gamma for gas =1.5)
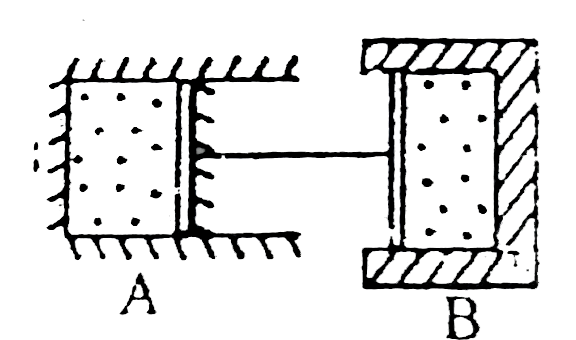
The mass of gas in cylinder BView SolutionTwo cylinder A and B having piston conneted by massless rod (as shown in figure). The cross-sectional area two cylinders are same & equal to 'S'.The cylinder A contains m gm of an ideal gas at Pressure P & temperature T0 The cylinder B contain identical gas at same temperature T0 but has different mass. The piston is held at the state in the position so that volume of gas in cylinder A & cylinder B are same and is equal to V0. The walls & piston of cylinder A are thermally insulated, whereas cylinder B is maintained at temperature T0 reservoir. The whole system is in vacuum. Now the piston is slowly released and it moves towards left & machanical equilibrium is reached at the state when the volume of gas in cylinder A becomes V02 Then (here gamma for gas=1.5)
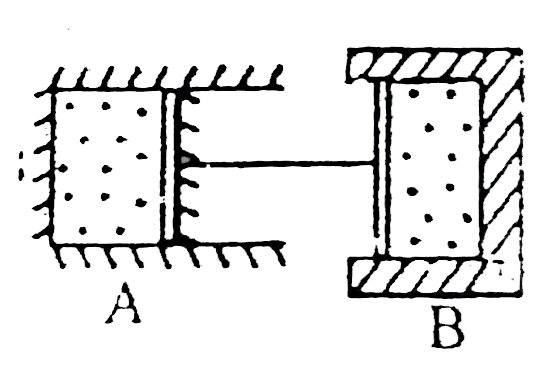
The change in internal energy of gas in cylinder AView SolutionTwo cylinder A and B having piston conneted by massless rod (as shown in figure). The cross-sectional area two cylinders are same & equal to 'S'.The cylinder A contains m gm of an ideal gas at Pressure P & temperature T0 The cylinder B contain identical gas at same temperature T0 but has different mass. The piston is held at the state in the position so that volume of gas in cylinder A & cylinder B are same and is equal to V0. The walls & piston of cylinder A are thermally insulated, whereas cylinder B is maintained at temperature T0 reservoir. The whole system is in vacuum. Now the piston is slowly released and it moves towards left & machanical equilibrium is reached at the state when the volume of gas in cylinder A becomes V02 Then (here gamma for gas =1.5)
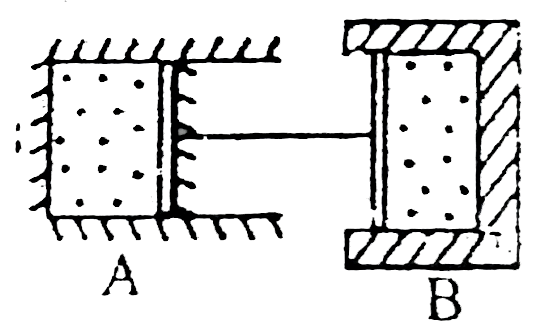
What will be the compressive force in conneting rod at equilibriumView SolutionAn ideal gas has pressure p0 in container of volume V0.The number of molecules of the gas is doubled without changing the temperature. What is the new value of pressure times volume?
View SolutionTwo adiabatic containers have volumes V1 and V2 respectively. The first container has monoatomic gas at pressure p1 and temperature T1. The second container has another monoatomic gas at pressure p2 and temperature T2. When the two containers are connected by a narrow tube, the final temperature and pressure of the gases in the containers are P and T respectively. Then
View Solution

