Determine the number of enegry states in the hydrogen atom corresponding to the principle quantum number n $= 2$ and calculate the energies of these states.
Strategy: An energy state of the hydrogen atom can be respresented by the wave function $ψ_{n, l, m_{1}}$ , which can be specified by sunstituting the values of the three quantum numbers.

Video Solution

Text Solution

Verified by Experts

For $n = 2, l$ can have the values $0$ and $1$ . For $l = 0, m_{l}$ can only be $0$ , corresponding to the state $ψ_{2, 0, 0}$ . For $l = 1, m_{l}$ can have the values $- 1, 0$ , or $1$ corresponding to the states $ψ_{2, 1, - 1}, ψ_{2, 1, 0}$ , and $ψ_{2, 1, 1}$ .
Hence, we have four allowed states:
$\begin{matrix} 2 s & ψ_{2, 0, 0} 2 p_{x} & ψ_{2, 1, - 1} 2 p_{y} & ψ_{2, 1, 0} 2 p_{z} & ψ_{2, 1, - 1,} \end{matrix}$
Since all of these states have the same principle quantum number $n = 2$ , they also have the sam energy, which can be calculated using Eq. For $n = 2$ , we get
$E_{2} = = - \frac{13.6}{{(2)}^{2}} e V a \to m^{- 1}$
$= - 3.4 e V {atom}^{- 1}$

Updated on:21/07/2023

Hydrogen atoms are excited from ground state of the principle quantum number $4$ . Then the number of spectral lines observed will be
A $3$
B $6$
C $5$
D $2$
For an electron in hydrogen atom match values of total energy and its quantum number.

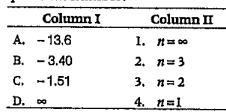A
B
C
D
The energy of hydrogen atom is its ground state is $- 13.6 e V$ The enrgy of the level corresponding to the quantum number $n = 5$ is
A $- 0.54 e V$
B $- 0.50 e V$
C $- 0.85 e V$
D $- 2.72 e V$