In,how many maxima can be obtained on the screen if wavelength of light used is and :
none of these
The correct Answer is:B
To solve the problem of how many maxima can be obtained on the screen in Young's Double Slit Experiment (YDSE) with a wavelength of light λ=200nm and slit separation d=700nm, we can follow these steps:
1. Understanding the Condition for Maxima:
In YDSE, maxima occur due to constructive interference. The condition for constructive interference is given by:
dsinθ=nλ
where n is an integer (0, 1, 2, ...), d is the distance between the slits, and λ is the wavelength of light.
2. Finding the Maximum Value of n:
To find the maximum number of maxima, we need to determine the largest integer n such that:
nλ≤d
Rearranging this gives:
n≤dλ
3. Substituting the Given Values:
Now we substitute the values of d and λ:
n≤700nm200nm=3.5
4. Determining the Maximum Integer n:
Since n must be an integer, the maximum value of n is:
nmax=3
5. Calculating the Total Number of Maxima:
The total number of maxima observed on the screen is given by the formula:
Total Maxima=2n+1
Substituting nmax=3:
Total Maxima=2×3+1=7
Final Answer:
Thus, the total number of maxima that can be obtained on the screen is 7.
---
In YDSE, maxima occur due to constructive interference. The condition for constructive interference is given by:
dsinθ=nλ
where n is an integer (0, 1, 2, ...), d is the distance between the slits, and λ is the wavelength of light.
To find the maximum number of maxima, we need to determine the largest integer n such that:
nλ≤d
Rearranging this gives:
n≤dλ
Now we substitute the values of d and λ:
n≤700nm200nm=3.5
Since n must be an integer, the maximum value of n is:
nmax=3
The total number of maxima observed on the screen is given by the formula:
Total Maxima=2n+1
Substituting nmax=3:
Total Maxima=2×3+1=7
Thus, the total number of maxima that can be obtained on the screen is 7.
Similar Questions
Knowledge Check
- Question 1 - Select One
In YDSE how many maxima can be obtained on the screen if wavelength of light used is 200nm and d = 700 nm.
A12B7C18Dnone of these - Question 2 - Select One
In YDSE, how many maximas can be obtained on a screen including central maxima in both sides of the central fringe if λ=3000Å,d=5000Å
A2B5C3D1 - Question 3 - Select One
In standard Young's double-slit experiment, 9 fringes are found to be formed in a portion of screen. Wavelength of light used is 600 nm. How many fringes will be formed in the same portion of screen if wavelength of light is changed to 450 nm?
A12B18C14D20
In Above question how many fringes will cross through the centre if an ideal paper piece is pasted on the other slit also ? the wavelength of light used is 600 nm.
View SolutionIn Young's double slit experiment, how many maxima can be obtained on a screen (including central maxima). If d=5λ2 (where λ is the wavelength of light)?
View SolutionA plane wavefront (wavelength=λ) is falling on YDSE apparatus as shown in the figure. The lower half of it is filled with water (refractive index =1.5). How many maximas can be theoretically obtained on the screen if d=98λ and D=20000λ (including the maxima at infinity)
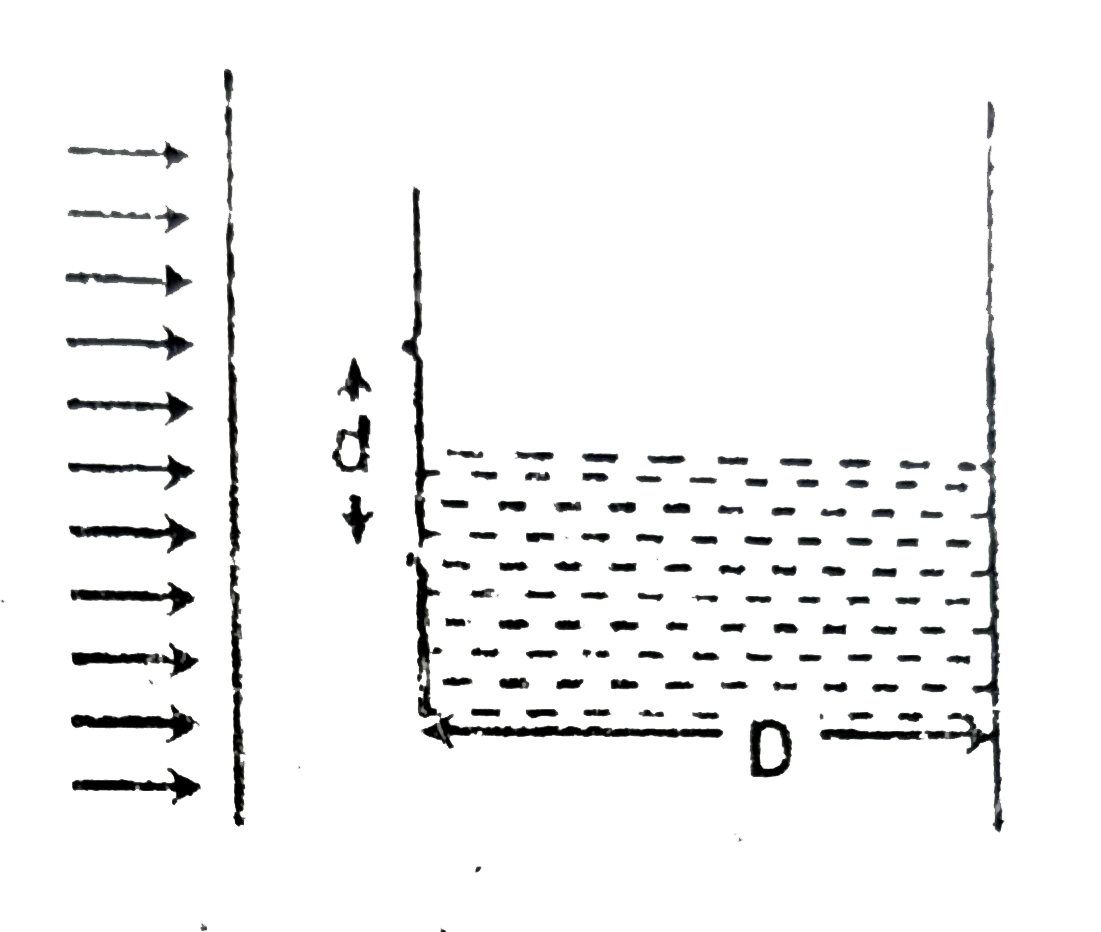 View Solution
View SolutionIn a standard YDSE slit width is 1mm and distance of screen from the slit is 1m. If wavelength of light is 632nm and bright fringe formed at y = 1.270 mm. Find path difference for the point
View SolutionIn a YDSE, distance between the slits and the screen is 1m, separation between the slits is 1mm and the wavelength of the light used is 5000nm. The distance of 100th maxima from the central maxima is:
View Solution

