When temperature of a material increases, its Young's modulus
increases
decreases
remains same
becomes infinity
The correct Answer is:B
Step by step video, text & image solution for When temperature of a material increases, its Young's modulus by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Level-v(Single answer)23videos
EXPERIMENTAL PHYSICS
- BOOK - NARAYNACHAPTER - EXPERIMENTAL PHYSICSEXERCISE - Multiple answer6videos
EXPERIMENTAL PHYSICS
- BOOK - NARAYNACHAPTER - ELECTROSTATICS AND GAUSS LAWEXERCISE - Intergers type question11videos
ELECTROSTATICS AND GAUSS LAW
- BOOK - NARAYNACHAPTER - MAGNETISMEXERCISE - LEVEL-II (H.W)24videos
MAGNETISM
Similar Questions
When temperature of a ferromagnetic material is increased by 10%, what is the percentage change in its magnetic susceptibility?
View SolutionA thin ring of radius R is made of a material of density ρand Young's modulus Y. If the ring is rotated about its centre in its own plane with angular velocity omega , find the small increases in its radius.
View Solution
Knowledge Check
- Question 1 - Select One
Young's modulus is
Athe ratio of linear strain to normal stressBthe ratio of normal stress to strainCproduct of linear and normal stressDsquare of the ratio of normal stress to linear strain - Question 2 - Select One
If the density of the material increase, the value of young's modulus
AincreasesBdecreasesCfirst increases, then decreasesDfirst decreases, then increases - Question 3 - Select One
The temperature of a wire is doubled. The Young's modulus of elasticity
Awill also doubleBwill becomes four timesCwill remain sameDwill decreases
In the given figure, if the dimensions of the two wires are same but materials are different, then Young's modulus is Extension
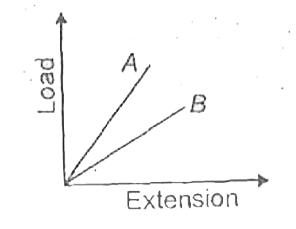 View Solution
View SolutionThe temperature of a wire is doubled. The young's modulus of elasticity
View SolutionAs temperture increases the Young's modulus of the material of a wire
View SolutionIn the given Fig. 7(CF).2, if the dimensions of the two wires are the same and materials are different, Young's modulus is
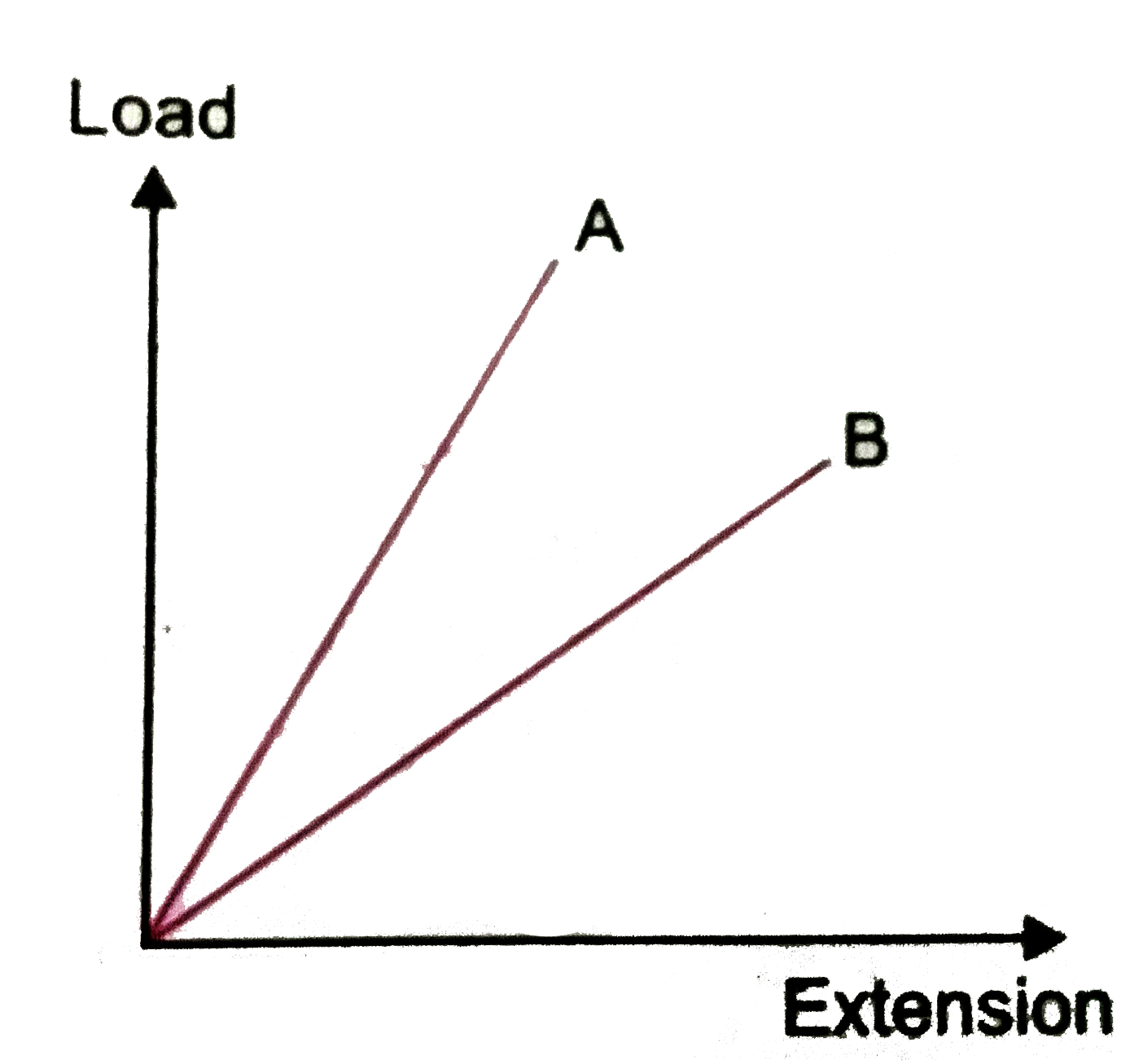 View Solution
View SolutionWith a rise of temperature, the Young’s modulus :
View Solution

