The magnetic field between the plates of a capacitor when r>R is given by-
μ0IDr2πR2
μ0ID2πR
μ0ID2πr
zero
The correct Answer is:C
To solve the problem regarding the magnetic field between the plates of a capacitor when r>R, we can follow these steps:
1. Understanding the Configuration:
- We have a parallel plate capacitor with plates of radius R.
- We are interested in the magnetic field at a distance r from the center of the capacitor plates, where r>R.
2. Applying Ampère's Law:
- According to Ampère's Law, the magnetic field B around a current-carrying conductor can be calculated using the formula:
B=μ0I2πr
- Here, μ0 is the permeability of free space, I is the current flowing through the capacitor, and r is the distance from the center of the capacitor.
3. Identifying the Region:
- Since we are looking at the region outside the plates of the capacitor (where r>R), we can directly use the formula derived from Ampère's Law.
4. Substituting Values:
- For r>R, the magnetic field B can be expressed as:
B=μ0I2πr
5. Final Expression:
- Therefore, the magnetic field between the plates of the capacitor when r>R is given by:
B=μ0I2πr
- We have a parallel plate capacitor with plates of radius R.
- We are interested in the magnetic field at a distance r from the center of the capacitor plates, where r>R.
- According to Ampère's Law, the magnetic field B around a current-carrying conductor can be calculated using the formula:
B=μ0I2πr
- Here, μ0 is the permeability of free space, I is the current flowing through the capacitor, and r is the distance from the center of the capacitor.
- Since we are looking at the region outside the plates of the capacitor (where r>R), we can directly use the formula derived from Ampère's Law.
- For r>R, the magnetic field B can be expressed as:
B=μ0I2πr
- Therefore, the magnetic field between the plates of the capacitor when r>R is given by:
B=μ0I2πr
Topper's Solved these Questions
- ELECTRO MAGNETIC WAVESBOOK - NARAYNACHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - LEVEL- II (C.W.)
15 Videos
- ELECTRO MAGNETIC WAVESBOOK - NARAYNACHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - LEVEL- III
10 Videos
- ELECTRO MAGNETIC WAVESBOOK - NARAYNACHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - C.U.Q
31 Videos
- ELECTRO MAGNETIC INDUCTIONBOOK - NARAYNACHAPTER - ELECTRO MAGNETIC INDUCTIONEXERCISE - Level-II (H.W)
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NARAYNACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSERTION & REASON
6 Videos
Similar Questions
A uniform magnetic field (B) is applied between the plates of a capacitor in the outward direction as shown in the figure. Surface charge density on the capacitor plates is σ.
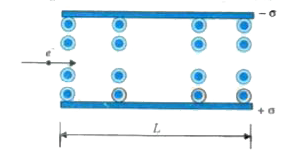
An electron is fired in between the plates, parallel to them, as shown in the figure. The electron is found to move straight in-between the plates of capacitor. Neglect the gravity. If L is the length of the plates then caleulate the time taken by the electron to cross the plates.
View Solution(a) A closed loop is held stationary in the magnetic field between the north and south poles of two permanent magnets held fixed. Can we hope to generate current in the loop by using very strong magnets?
(b) A closed loop moves normal to the constant electric field between the plates of a large capacitor. Is a current induced in the loop (i) when it is wholly inside the region between the capacitor plates (ii) when it is partially outside the plates of the capacitor? The electric field is normal to the plane of the loop.
(c) A rectangular loop and a circular loop are moving out of a uniform magnetic field region (Fig. 6.8) to a field-free region with a constant velocity v. In which loop do you expect the induced emf to be constant during the passage out of the field region? The field is normal to the loops.

(d) Predict the polarity of the capacitor in the situation described by Fig. 6.9.

View Solution
Knowledge Check

Magnetic field in space between the plates isA12μ0j2BzeroCμ0jD2μ0jDisplacement current is produced between the plates of a capacitor when potential difference between the plates
Ais maximumBis minimumCis zeroDis changing with timeDisplacement current exists between the plates of capacitor , when charge of the capacitor
AConstantBMaximumCChangingDBoth (1) &(3)
How will the electric field between the plates in parallel plate capacitor change, if the charge on the plates is doubled?
View SolutionThe space between the electrodes of a parallel-plate capacitor is filled with a uniform poorly conducting medium of conductivity σ and permittivity ε. The capacitor plates shaped as round discs are separted by a distance d. Neglecting the edge effects, find the magnetic field strength between the plates at a distance r from their axis if an ac voltage V=Vmcosωt is applied to the capacitor.
View SolutionThe electric field (→E) between two parallel plates of a capacitor will be uniform if.
View SolutionThe magnetic field between the circulate plates of radius 12cm separted by distance of 4mm of a parallel plate capacitor of capacitance 100pF. Along the axis of plates having conduction current of 0.15A is
View SolutionA : The displacement current goes through the gap between the plates of a capacitor when the charge on the capacitor does not change.
R : Displacement current arises only when the electric field is constant.
View Solution
