For adiabatic processes (γ=CpCv)
PγV=constant
TγV=constant
TVγ−1=constant
TVγ=constant
The correct Answer is:C
Step by step video, text & image solution for For adiabatic processes (gamma = (C_(p))/(C_(v))) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - TARGET PUBLICATIONCHAPTER - KINETIC THEORY OF GASES AND RADIATION EXERCISE - Evaluation test18videos
KINETIC THEORY OF GASES AND RADIATION
- BOOK - TARGET PUBLICATIONCHAPTER - KINETIC THEORY OF GASES AND RADIATION EXERCISE - critical thinking104videos
KINETIC THEORY OF GASES AND RADIATION
- BOOK - TARGET PUBLICATIONCHAPTER - INTERFERENCE AND DIFFRACTIONEXERCISE - EVALUATION TEST20videos
INTERFERENCE AND DIFFRACTION
- BOOK - TARGET PUBLICATIONCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENTEXERCISE - EVALUATION TEST19videos
MAGNETIC EFFECT OF ELECTRIC CURRENT
Similar Questions
Knowledge Check
- Question 1 - Select One
In an isobaric process, the ratio of heat supplied to the system (dQ) and work done by the system (dW) for diatomic gas is
AγBγ−1Cγγ+1Dγγ−1 - Question 2 - Select One
At a given temperature if Vrms is the root mean square velocity of the molecules of a gas and Vs be the velocity of sound in it, then these are related as (γ=CpCv)
Avrms=vsBvrms=√3γ×vsCvrms=√γ3×vsDvrms=(3γ)×vs - Question 3 - Select One
In an adiabatic process, R=23Cv. The pressure of the gas will be proportional to:
AT5/3BT5/2CT5/4DT5/6
Prove that the pressure and the volume of a degenerate electron gas are related by an equation similar to the Poisson equation, and find the adiabatic index γ=Cp/Cv.
View SolutionCoefficient of isothermal elasticity Eθ and coefficient of adiabatic elasticity Eϕ are related by (γ=Cp/Cv)
View SolutionAn ideal gas at pressure P is adiabatically compressed so that its density becomes n times the initial value. The final pressure of the gas will be (γ=CPCV)
View SolutionPi,Viare initial pressure and volumes and Vf is final volume of a gas in a thermodynamic process respectively. If PVn= constant, then the amount of work done by gas is:(γ=Cp/Cv). Assume same, initial states & same final volume in all processes.
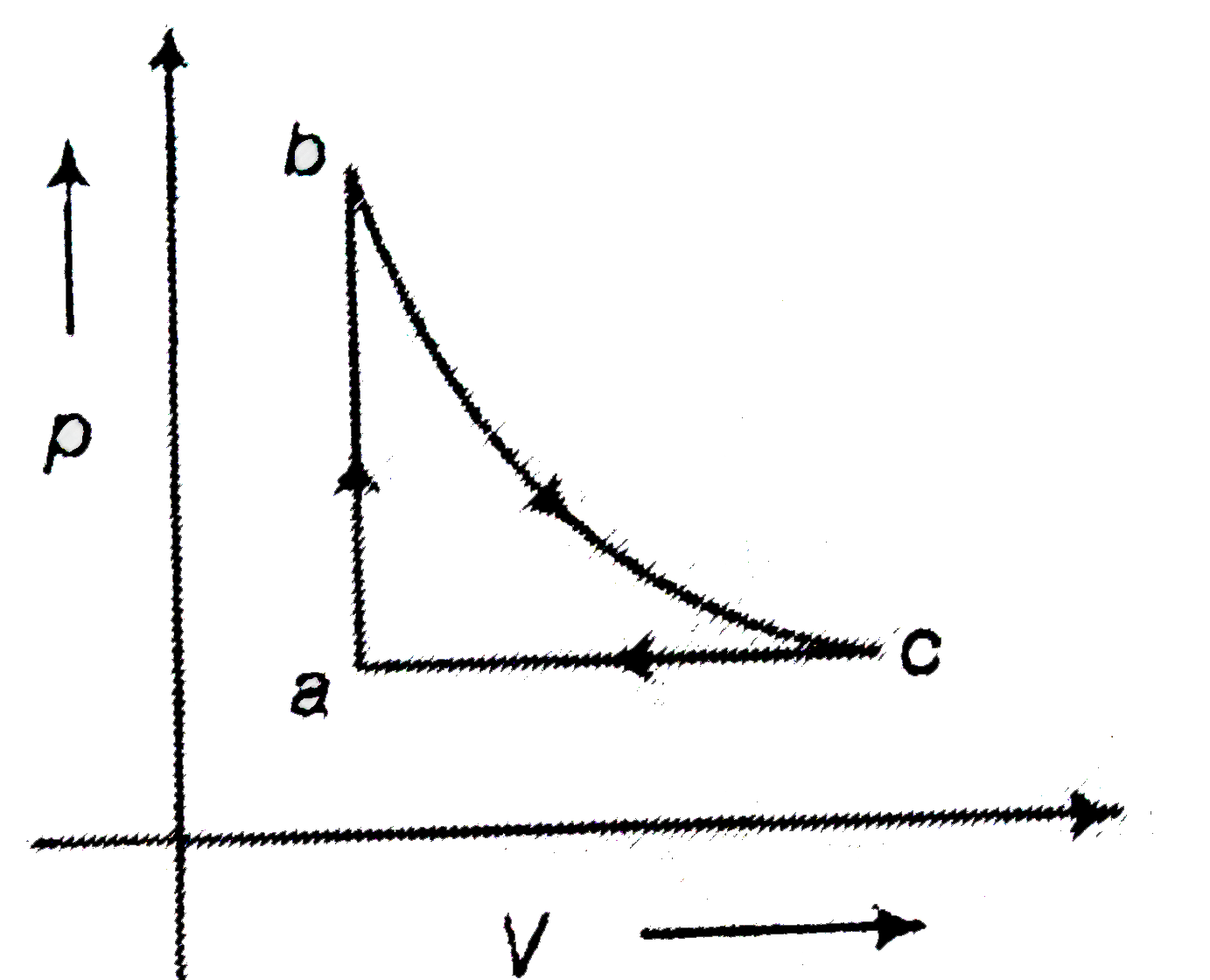
View Solution0.2moles of an ideal gas, is taken around the cycle abc as shown in the figure. The path b-c is adiabatic process, a-b is isovolumic process and c-a is isobaric process. The temperature at a and b are TA=300K and Tb=500K and pressure at a is 1 atmospere. find the volume at c.
(Given, γ=CpCv=53,R=8.205×10−2L/atm/mol-K)
 View Solution
View Solution

