A tent is in the shape of triangular prism resting on a reactangular base. If AB=AC,AD=0.8m,BC=3m and a length of the tent =6m,∠ABC=42∘ , then find,
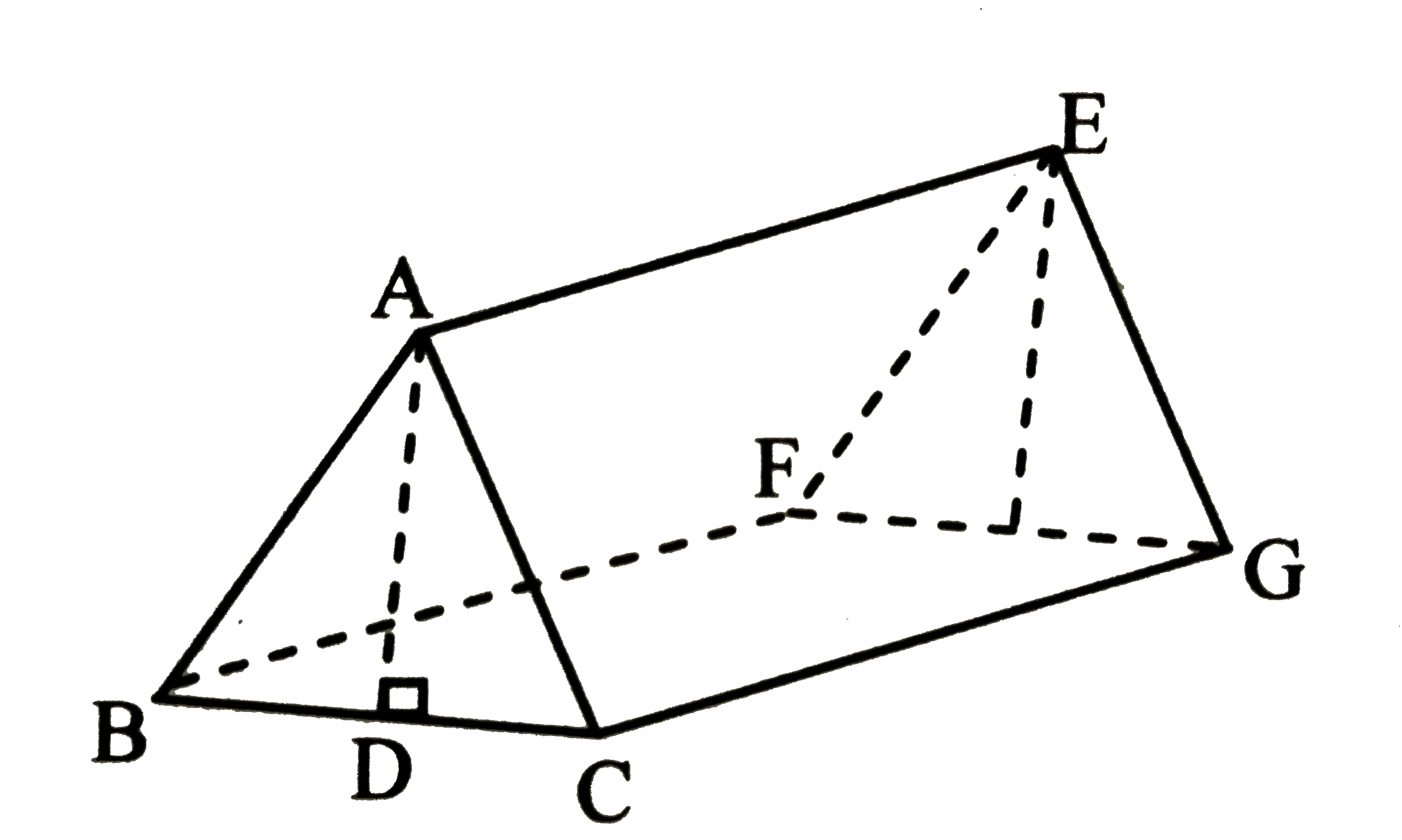
a. ∠ACB
b. AB
c. Volume of the tent
The correct Answer is:a. 42∘
b. 1.7m
c. 7.2m3
Step by step video & image solution for A tent is in the shape of triangular prism resting on a reactangular base. If AB = AC, AD = 0.8 m, BC= 3 m and a length of the tent = 6m, angle ABC = 42^(@) , then find, a. angle ACB b. AB c. Volume of the tent by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MENSURATIONBOOK - TARGET PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Textual Activity
1 Videos
- MENSURATIONBOOK - TARGET PUBLICATIONCHAPTER - MENSURATIONEXERCISE - Additional Problems for Practice (Based on Practice Set 7.4)
6 Videos
- LINEAR EQUATIONS IN TWO VARIABLES BOOK - TARGET PUBLICATIONCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - Chapter Assessment
20 Videos
- MODEL QUESTION PAPERBOOK - TARGET PUBLICATIONCHAPTER - MODEL QUESTION PAPEREXERCISE - Questions
23 Videos
Similar Questions
Knowledge Check
ΔABC is right-angled at C. If AC=5cmandBC=12cm find the length of AB.
A17B7C13D14In a metallic triangular sheet ABC, AB=BC=l. If M is mass of sheet, what is the moment of inertia about AC?
 AMl24BMl212CMl26DMl218
AMl24BMl212CMl26DMl218
In a right-angled triangle ABC, if angle B=90°, BC = 3 cm and AC = 5 cm, then the length of side AB is
View SolutionIn the figure, if ∠ACB=∠CDA,AC=6cm and AD = 3 cm, then find the length of AB
View SolutionFind length of AD in ΔABC if AB = 5 AC = 6 and BC = 8
View SolutionA tent is in the shape of a right circular cylinder up to a height of 3 m and conical above it. The total height of the tent is 13.5 m and the radius of its base is 14 m. Find the cost of cloth required to make the tent at the rate of ₹ 80 per square metre.[Take π=227]
View SolutionIn triangle ABC, right angled at B, AC = 20 m, ∠C=45∘, find the value of AB.
View SolutionIn a right angled triangle ABCABC=90∘,ACB=30∘ and AB=8cm. Find the length of the other sides of the triangle.
View Solution
