Given four non zero vectors ¯a,¯b,¯cand¯d. The vectors ¯a,¯band¯c are coplanar but not collinear pair by pairand vector ¯d is not coplanar with vectors ¯a,¯band¯candˆ¯a¯b=ˆ¯b¯c=π3,(¯d¯b)=β ,If (¯d¯c)=cos−1(mcosβ+ncosα) then m−n is :
cos−1(cosβ−cosα)
sin−1(cosβ−cosα)
sin−1(sinβ−sinα)
cos−1(tanβ−tanα)
The correct Answer is:A
Step by step video, text & image solution for Given four non zero vectors bar a,bar b,bar c and bar d. The vectors bar a,bar b and bar c are coplanar but not collinear pair by pairand vector bar d is not coplanar with vectors bar a,bar b and bar c and hat (bar a bar b) = hat (bar b bar c) = pi/3,(bar d bar b)=beta ,If (bar d bar c)=cos^-1(mcos beta+ncos alpha) then m-n is : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (More Than One Correct Option Type Questions)36videos
PRODUCT OF VECTORS
- BOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Statement I And Ii Type Questions)12videos
PRODUCT OF VECTORS
- BOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise For Session 410videos
PRODUCT OF VECTORS
- BOOK - ARIHANT MATHSCHAPTER - PROBABILITYEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)55videos
PROBABILITY
- BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)21videos
PROPERTIES AND SOLUTION OF TRIANGLES
Similar Questions
Knowledge Check
- Question 1 - Select One
IF ¯a,¯b,¯c are mutually perpendicular vectors having magnitudes 1,2,3 respectively
then [¯a+¯b+¯c ¯b−¯a ¯c]=?A0B6C12D18 - Question 2 - Select One
If ¯a,¯b,¯c are non-coplanar vectors then [¯a+2¯b ¯a+¯c ¯b]=
A0B[¯a,¯b,¯c]C−[¯a,¯b,¯c]D2[¯a,¯b,¯c]
If four vectors ¯a,¯b,¯c,¯d are coplanar, then (¯aׯb)×(¯cׯd)=
View Solution¯a,¯b,¯c are three coplanar vectors, If ¯a is not parallel to ¯b, Show that
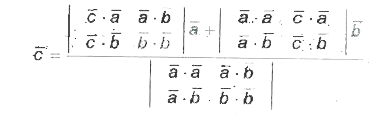 View Solution
View SolutionThe vectors ¯a and ¯b are non-collinear.If ¯a+(x+1)¯b and (2x−3)¯b−¯a are collinear,then the value of x is
View SolutionIf ¯a,¯b,¯c are three non zero vectors, then ¯a.¯b=¯a.¯c⇒
View SolutionIf the vectors ¯a=2¯i+3¯j+6¯k and ¯b are collinear and ∣∣¯b∣∣=21, then ¯b equals to
View SolutionIf ¯a,¯b,¯c are non coplanar vectors and λ is a real number then [λ(¯a+¯b)λ2¯bλ¯c]=[¯a¯b+¯c¯b] for
View Solution

