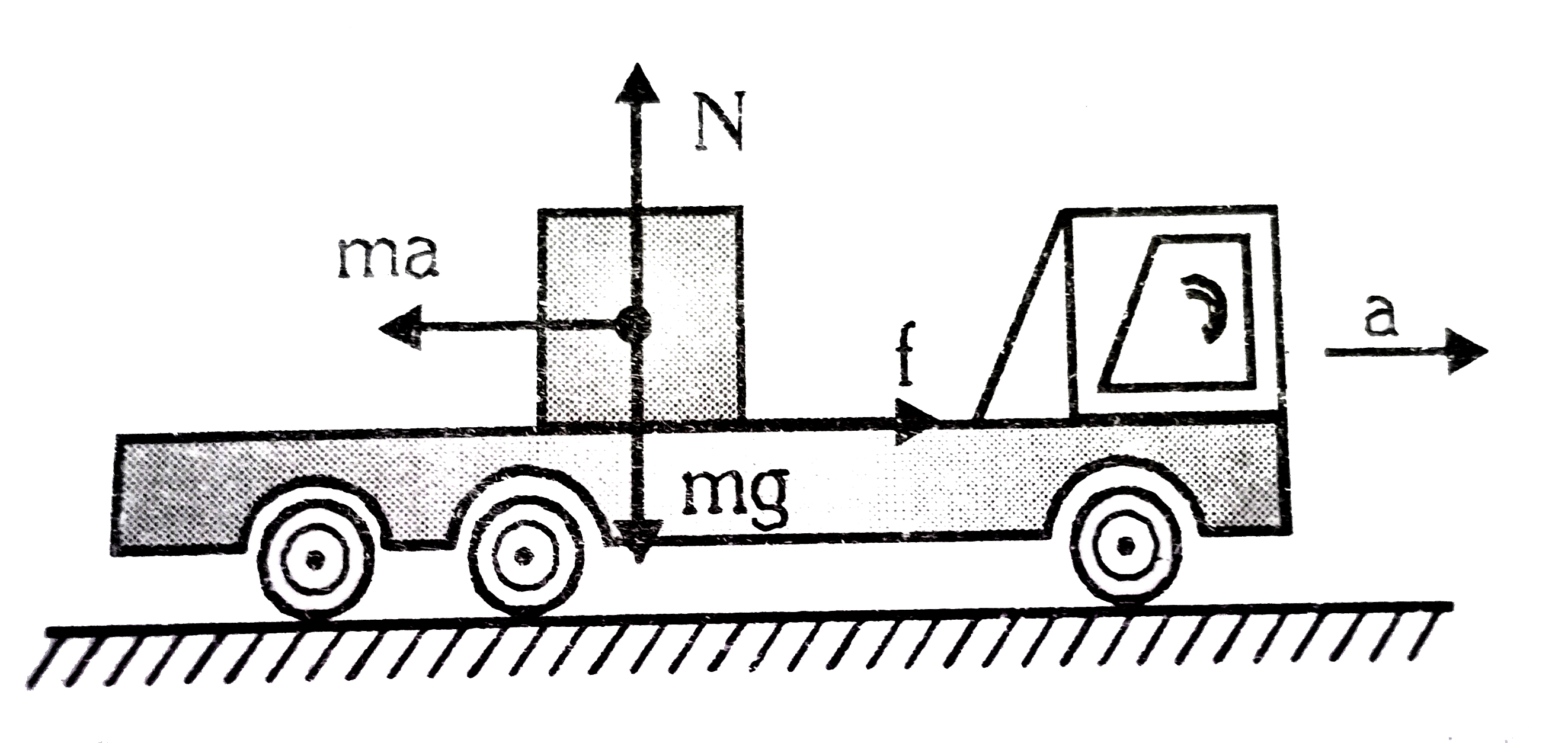
A body of 100 kg is placed on a truck. The coefficient of static friction between the body and the truck is 0.2. The truck suddenly decreases its speed from 90 km/hr to 36 km/hr in 5 second. Then
The block does not move
the block slips forward and hits the driver's cabin
block shifts backward
nothing can be said about the block
The correct Answer is:B
To solve the problem, we need to determine whether the body on the truck will slip or remain stationary as the truck decelerates. We will do this by calculating the deceleration of the truck and comparing it with the maximum static frictional force that can act on the body.
1. Convert velocities from km/hr to m/s:
- Initial velocity (U) = 90 km/hr = 90×10003600=25m/s
- Final velocity (V) = 36 km/hr = 36×10003600=10m/s
2. Calculate the deceleration (a) of the truck:
- We can use the formula for acceleration:
a=V−Ut
- Substituting the values:
a=10m/s−25m/s5s=−15m/s5s=−3m/s2
- The negative sign indicates deceleration.
3. Calculate the maximum static frictional force (F_friction):
- The formula for static friction is:
Ffriction=μs⋅N
- Where μs is the coefficient of static friction and N is the normal force. For a horizontal surface, N=mg:
N=100kg⋅9.8m/s2=980N
- Therefore, the maximum static frictional force is:
Ffriction=0.2⋅980N=196N
4. Calculate the force required to keep the body stationary (F_required):
- The force required to keep the body from slipping due to the truck's deceleration is given by:
Frequired=m⋅a
- Substituting the values:
Frequired=100kg⋅3m/s2=300N
5. Compare the forces:
- We have:
- Maximum static frictional force Ffriction=196N
- Required force to keep the body stationary Frequired=300N
6. Conclusion:
- Since Frequired(300N)>Ffriction(196N), the body will slip forward.
- Initial velocity (U) = 90 km/hr = 90×10003600=25m/s
- Final velocity (V) = 36 km/hr = 36×10003600=10m/s
- We can use the formula for acceleration:
a=V−Ut
- Substituting the values:
a=10m/s−25m/s5s=−15m/s5s=−3m/s2
- The negative sign indicates deceleration.
- The formula for static friction is:
Ffriction=μs⋅N
- Where μs is the coefficient of static friction and N is the normal force. For a horizontal surface, N=mg:
N=100kg⋅9.8m/s2=980N
- Therefore, the maximum static frictional force is:
Ffriction=0.2⋅980N=196N
- The force required to keep the body from slipping due to the truck's deceleration is given by:
Frequired=m⋅a
- Substituting the values:
Frequired=100kg⋅3m/s2=300N
- We have:
- Maximum static frictional force Ffriction=196N
- Required force to keep the body stationary Frequired=300N
- Since Frequired(300N)>Ffriction(196N), the body will slip forward.
Topper's Solved these Questions
- FRICTIONAL IN SOLIDS AND LIQUIDS BOOK - MARVEL PUBLICATIONCHAPTER - FRICTIONAL IN SOLIDS AND LIQUIDS EXERCISE - TEST YOUR GRASP
10 Videos
- FORCE, WORK AND TORQUEBOOK - MARVEL PUBLICATIONCHAPTER - FORCE, WORK AND TORQUEEXERCISE - TEST YOUR GRASP
10 Videos
- MAGNETIC EFFECT OF ELECTRIC CURRENT BOOK - MARVEL PUBLICATIONCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENT EXERCISE - TEST YOUR GRASP
10 Videos
Similar Questions
A block of mass 1 kg lies on a horizontal surface in a truck. The coefficient of static friction between the block and the surface is 0.6. If the acceleration of the truck is 5m/s2, the frictional force acting on the block is…………newtons.
View SolutionA block of mass 2kg is placed on a truck as shown in the figure. The coefficient of friction between the block and surface is 0.5. The truck starts from the rest and moves with acceleration a0. (a) Find the friction force acting on the block if (i) a0=2m/s2 , (ii) 4m/s2 , (iii) 6m/s2 and (b) if acceleration of truck a0=8m/s2. After how much time the blocks falls off the truck and the distance travelled by the truck in this time.
View Solution
Knowledge Check
A body of mass 2 kg is placed on rough horizontal plane. The coefficient of friction between body and plane is 0.2 Then,
 Abody will move in forward direction if F = 5 NBbody will be move in backward direction with acceleration 0.5m/s2 if force F = 3NCif F = 3N, then body will be in rest conditionDBoth a and c are correct
Abody will move in forward direction if F = 5 NBbody will be move in backward direction with acceleration 0.5m/s2 if force F = 3NCif F = 3N, then body will be in rest conditionDBoth a and c are correctA block of mass 1 kg is placed on a truck which accelerates with acceleration 5ms−2. The coefficient of static friction between the block and truck is 0.6. The frictional force acting on the block is
A5 NB6 NC5.82 ND4.6 NA body is struck to the front part of the truck. The coefficient of friction between the body and truck is μ. The minimum acceleration with which the truck should travel so that the body does not fall down is
Aμ/gBμgCg/μDμ2g
A block of mass 1kg lies on a horizontal surface in a turck, the coefficient of static fricition between the block and the surface is 0.6, what is the force of fricition on the block. If the acceleration of the truck is 5m/s2.
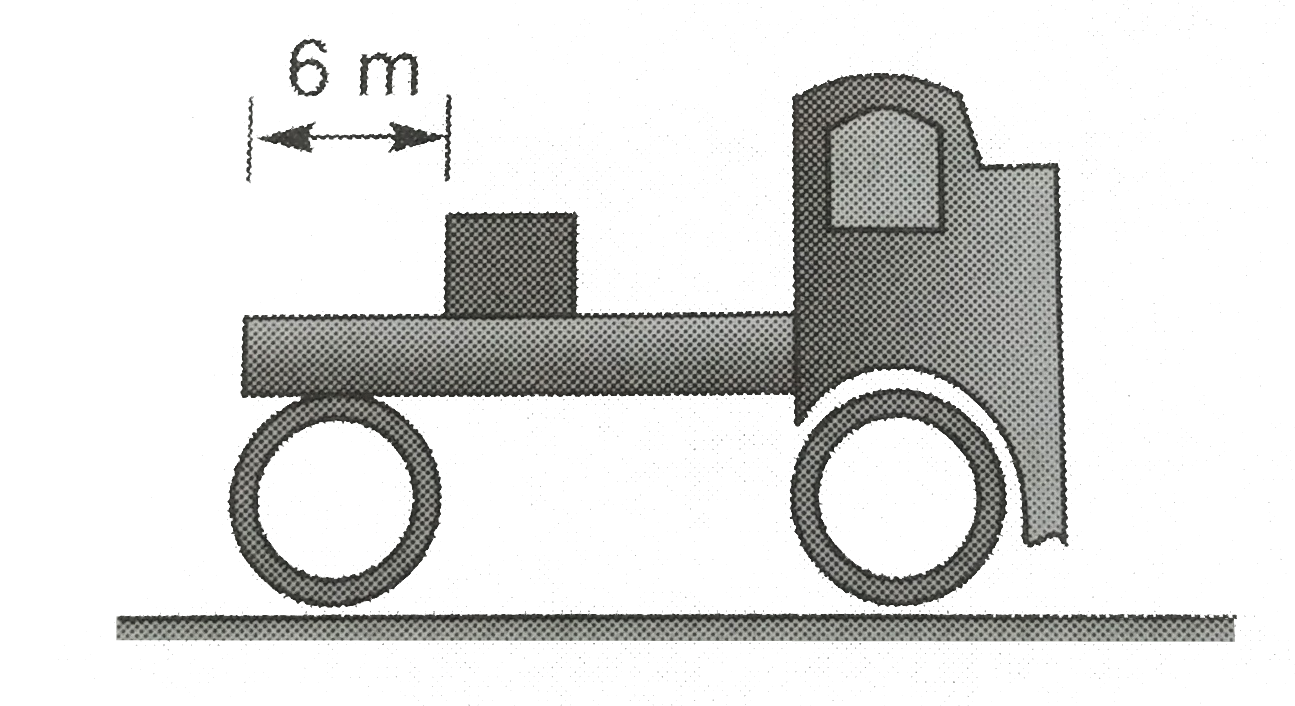
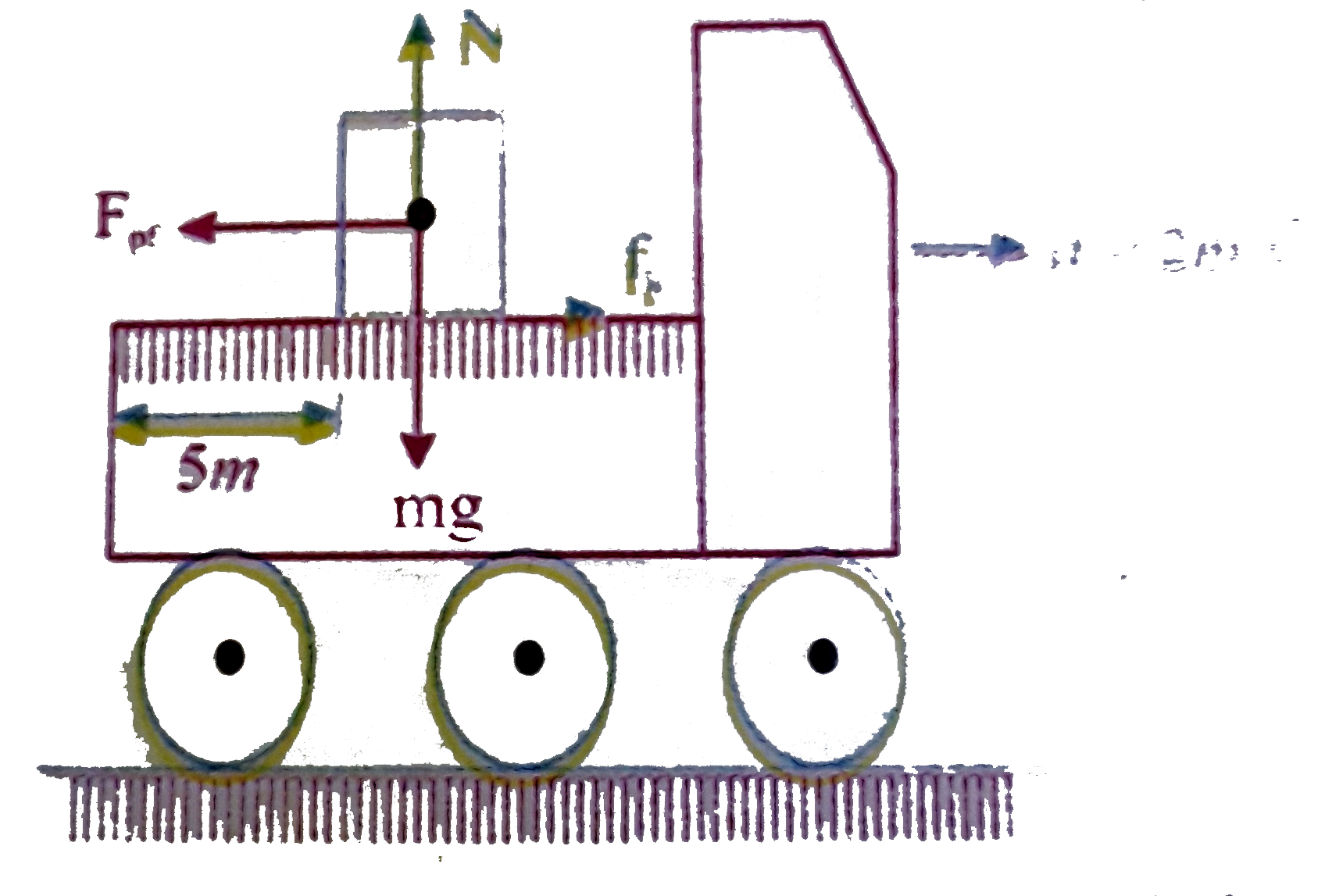
View SolutionThe rear side of a truck is open. A box of 40kg mass is placed 5m away from the open end as shown in figure. The coefficient of friction between the box and the surface is 0.15. On a straight road, the truck starts from rest and accel erating with 2m/s2. At what distance from the starting point does the box fall off the truck? Ignore the size of the box
View SolutionA 30kg mass is intially at rest on floor of a truck . The coefficient of static friction between the mass and the floor of truck in 0.3 and coefficient of kinetic friction is 0.2 . Intially the truck is travelling due east at constant speed.Find the magnitude and direction of the friction force acting on the mass , if : (take g=10m/s2)
(a) the truck acdceleration at 1.8m/s2 eastward
(b) the truck acdceleration at 3.8m/s2 westward
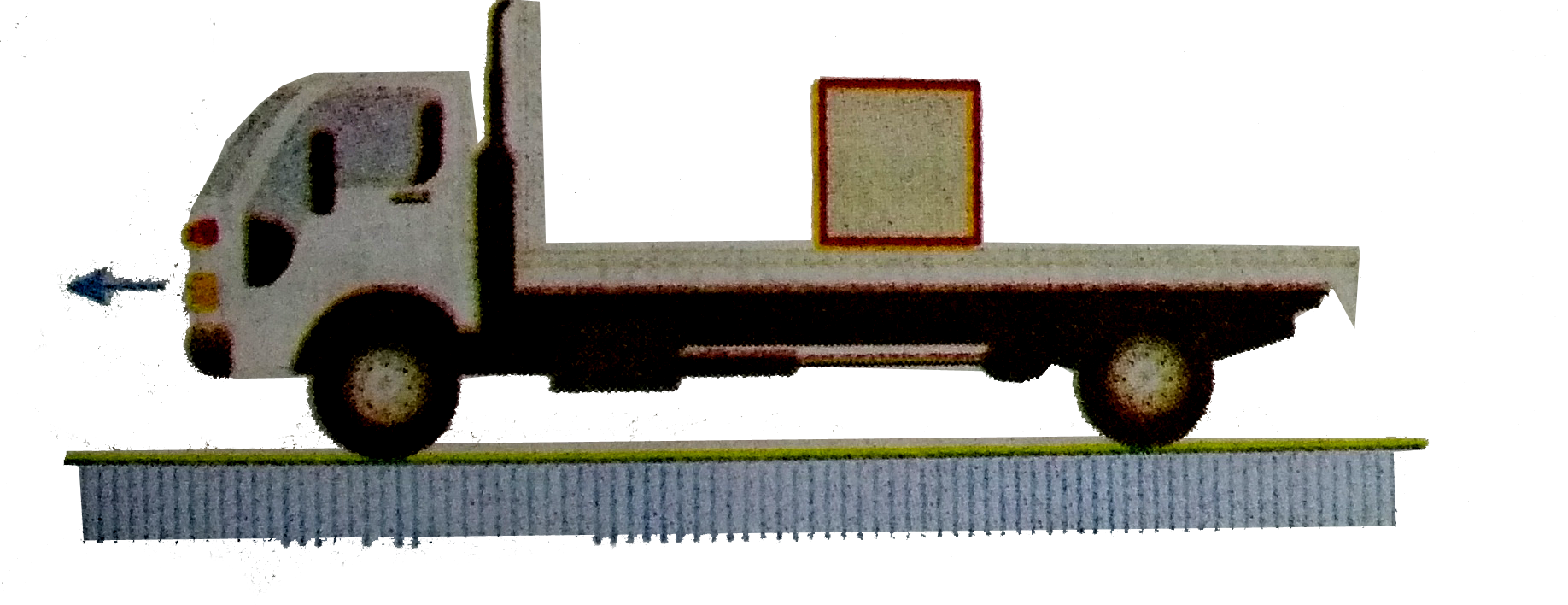
View SolutionThe rear side of a truck is open A box of 40kg mass is placed 5m away from the open end as shown in The coefficient of friction between the box and the surface is 0.15. On a straight road, the truck starts from rest and accel erating with 2m/s2. At what dis tance from the starting point does the box dis-tance from the starting point does the box fall from the truck? (Ignore the size of the box)
 .
.
View SolutionThe rear side of a truck is open and a box of mass 20kg is placed on the truck 4 meters away from the open end coofficent of friction between truck and block is 0.15 the truck starts from rest with an acceleration of 2m/sec2on a straight road The box will fall off the truck when truck is at a distance from the starting point equal to(g=10m/s2)
View Solution
