If the increments in u and v are δuandδv respectively, then the incremnt in their product uv is
δuδv
uδv+vδu
uδv+vδu+δuδv
uδu+vδv
The correct Answer is:C
Step by step video, text & image solution for If the increments in u and v are delta u and delta v respectively, then the incremnt in their product uv is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- APLICATIONS OF DERIVATIVESBOOK - MARVEL PUBLICATIONCHAPTER - APLICATIONS OF DERIVATIVESEXERCISE - MULTIPLE CHOICE QUESTIONS (TEST YOUR GRASP - I : CHAPTER 12)
19 Videos
- APLICATIONS OF DERIVATIVESBOOK - MARVEL PUBLICATIONCHAPTER - APLICATIONS OF DERIVATIVESEXERCISE - MULTIPLE CHOICE QUESTIONS (TEST YOUR GRASP - II : CHAPTER 12)
19 Videos
- APPLICATIONS OF DEFINITE INTEGRALS BOOK - MARVEL PUBLICATIONCHAPTER - APPLICATIONS OF DEFINITE INTEGRALS EXERCISE - TEST YOUR GRASP
11 Videos
Similar Questions
Knowledge Check
If the increments in u and v are δuandδv respectively, then the increment in uv is
Auδv+vδuv2Bvδu−δvv2Cvδu−uδvv(v+δv)Duδu−vδvv2ΔHandΔU are related as
AΔU=ΔH+PΔVBΔH=ΔU−PΔVCΔH=PΔV−ΔUDΔH=ΔU+PΔVFor a diatomic gas change in internal energy for unit change in temperature for constant pressure and constant volume is ΔU1 and ΔU2 respectively. The ratio of ΔU1:ΔU2 is
A5:3B3:5C1:1D5:7
An ideal gas whose adiabatic exponent equals γ is expanded so that ΔQ+ΔU=0. Then the equation of the process in the variables T and V is
View SolutionIf ΔH is the change in enthalpy and ΔU the change in internal energy accompanying a gaseous reaction (A) ΔH is always greater than ΔU (B) ΔH<ΔU only if the number of moles of the products is greater than the number of the reactants (C) ΔH is always less than ΔU (D) ΔH<ΔU only if the number of moles of the products is less than the number of the reactants
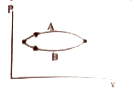
View SolutionLet ΔU1andΔU2 be the changes in energy of the system in the process A and B then.
View SolutionThe difference between ΔHandΔU is equal to
View SolutionThe process in which the value of ΔU=0 is :
View Solution
