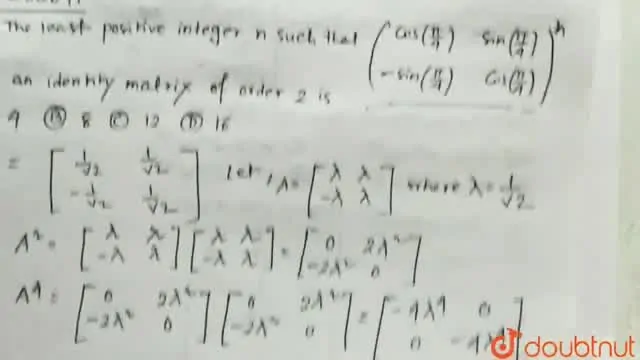The least positive integer n such that ⎛⎜⎝cos(π4)sin(π4)−sin(π4)cos(π4)⎞⎟⎠n is an identity matrix of order 2 is Solution in Bengali
4
8
12
16
The correct Answer is:B
Step by step video, text & image solution for The least positive integer n such that ({:(cos""((pi)/(4)),sin""((pi)/(4))),(-sin""((pi)/(4)),cos""((pi)/(4))):})^n is an identity matrix of order 2 is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTION PAPER -2018EXERCISE - WBJEE45videos
QUESTION PAPER -2018
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUADRATIC EQUATIONSEXERCISE - Sample Question for Competitive Exams (Assertion- Reason Type)2videos
QUADRATIC EQUATIONS
- BOOK - CHHAYA PUBLICATIONCHAPTER - QUESTION PAPER 2017EXERCISE - UNIT-44videos
QUESTION PAPER 2017
Similar Questions
The approximate value of sin31∘ is
02:24View SolutionLet ax2+bx+c=0,a≠0 (a,b,c∈R) has no real roots and a+b+2c=2
Statement-I: ax2+bx+c>0∀x∈R
Statement II: a+b is positive.02:24View SolutionThe equation of the plane through (1,2,-3) and (2,-2,1) and parallel to x axis is
06:42View SolutionEvaluate the following: cos(2π)3cosπ4−sin(2π)3sinπ4
02:24View Solution(cos ^ (2) ((pi) / (4) -A) -sin ^ (2) ((pi) / (4) -A)) / (cos ^ (2) ((pi) / (4) + A) + sin ^ (2) ((pi) / (4) + A) =)
01:46View SolutionProve that
(i) cos (n + 2) x cos (n+1) x +sin (n+2) x sin (n+1) x = cos x)
(ii) cos .(π4−x) cos .(π4−y) - sin (π4−x) sin (π4−y)= sin (x+y)10:23View Solutionसिद्ध कीजिए कि : sin.π4cos.π12+cos.π4sin.π12=√32
02:15View Solutioncos(π4+A)cos(π4−B)+sin(π4+A)⋅sin(π4−B)=
03:16View SolutionI : If sin(π4cotθ)=cos(π4tanθ) then θ=nπ+π/4,n∈Z
II : If tan(π2sinθ)=cot(π2cosθ) then sin(θ+π4)=±1√201:24View SolutionProve that cos(π4−x)cos(π4−y)−sin(π4−x)sin(π4−y)=sin(x+y)
03:11View SolutionThe least positive integer n such that [cosπ4sinπ4−sinπ4cosπ4]n
is an identity matrix of order 2 is01:46View Solutioncos(π4−x)cos(π4−y)−sin(π4−x)sin(π4−y)=sin(x+y)
02:13View Solutioncos(π4−x)cos(π4−y)−sin(π4−x)sin(π4−y)=sin(x+y)
View SolutionProve cos(π4−x)cos(π4−y)−sin(π4−x)sin(π4−y)=sin(x+y)
01:32View SolutionProve that:cos(π4−x)cos(π4−y)−sin(π4−x)sin(π4−y)=sin(x+y)
02:33View Solution