The bromination of acetone that occurs in acid solution is represented by this equation.
CH3COCH3(aq)+Br2(aq)→
CH3COCH2Br(aq)+H+(aq)+Br(aq)
These kinetic data were obtained for given reaction concentrations.
Initial concentration, M
[CH2COCH3][Br2][H+](Initail rate) (disappearance of Br2)0.300.050.051.5×10−50.300.100.055.7×10−50.300.100.101.2×10−40.400.50.203.1×10−4
rate =k[CH3COCH3][Br2]
rate =k[CH3COCH3][Br2][H+]2
rate =k[CH3COCH3][Br2][H+]
rate =k[CH3COCH3][H+]
The correct Answer is:D
To solve the problem of determining the order of the reaction involving the bromination of acetone, we will analyze the given kinetic data step by step.
Step 1: Write the Rate Law Expression
The rate law for the reaction can be expressed as:
Rate=k(CH3COCH3)a(Br2)b(H+)c
where k is the rate constant, and a, b, and c are the orders of the reaction with respect to each reactant.
Step 2: Set Up the Equations from the Given Data
Using the provided initial rates and concentrations, we can set up equations based on the rate law.
1. For the first set of data:
1.5×10−5=k(0.30)a(0.05)b(0.05)c (Equation 1)
2. For the second set of data:
5.7×10−5=k(0.30)a(0.10)b(0.05)c (Equation 2)
3. For the third set of data:
1.2×10−4=k(0.30)a(0.10)b(0.10)c (Equation 3)
4. For the fourth set of data:
3.1×10−4=k(0.40)a(0.05)b(0.20)c (Equation 4)
Step 3: Compare Equations to Determine the Order
To find the values of a, b, and c, we will compare the equations.
Finding b:
- Compare Equation 1 and Equation 2:
5.7×10−51.5×10−5=k(0.30)a(0.10)b(0.05)ck(0.30)a(0.05)b(0.05)c
Simplifying gives:
5.71.5=(0.10)b(0.05)b⟹5.71.5=2b
Solving for b:
3.8≈2b⟹b≈0
Finding c:
- Compare Equation 2 and Equation 3:
1.2×10−45.7×10−5=k(0.30)a(0.10)b(0.10)ck(0.30)a(0.10)b(0.05)c
Simplifying gives:
1.25.7=(0.10)c(0.05)c⟹1.25.7=2c
Solving for c:
0.21≈2c⟹c≈1
Finding a:
- Compare Equation 3 and Equation 4:
3.1×10−41.2×10−4=k(0.40)a(0.05)b(0.20)ck(0.30)a(0.10)b(0.10)c
Simplifying gives:
3.11.2=(0.40)a(0.20)c(0.30)a(0.10)c
This leads to:
3.11.2≈(0.40)a(0.20)1(0.30)a(0.10)1
Solving for a:
2.58≈(0.400.30)a⋅2⟹a≈1
Step 4: Summarize the Orders
From our calculations:
- a=1
- b=0
- c=1
Final Rate Law Expression
The overall rate law is:
Rate=k(CH3COCH3)1(Br2)0(H+)1
This indicates that the reaction is first order with respect to acetone and hydrogen ions, and zero order with respect to bromine.
The rate law for the reaction can be expressed as:
Rate=k(CH3COCH3)a(Br2)b(H+)c
where k is the rate constant, and a, b, and c are the orders of the reaction with respect to each reactant.
Using the provided initial rates and concentrations, we can set up equations based on the rate law.
1.5×10−5=k(0.30)a(0.05)b(0.05)c (Equation 1)
5.7×10−5=k(0.30)a(0.10)b(0.05)c (Equation 2)
1.2×10−4=k(0.30)a(0.10)b(0.10)c (Equation 3)
3.1×10−4=k(0.40)a(0.05)b(0.20)c (Equation 4)
To find the values of a, b, and c, we will compare the equations.
- Compare Equation 1 and Equation 2:
5.7×10−51.5×10−5=k(0.30)a(0.10)b(0.05)ck(0.30)a(0.05)b(0.05)c
Simplifying gives:
5.71.5=(0.10)b(0.05)b⟹5.71.5=2b
Solving for b:
3.8≈2b⟹b≈0
- Compare Equation 2 and Equation 3:
1.2×10−45.7×10−5=k(0.30)a(0.10)b(0.10)ck(0.30)a(0.10)b(0.05)c
Simplifying gives:
1.25.7=(0.10)c(0.05)c⟹1.25.7=2c
Solving for c:
0.21≈2c⟹c≈1
- Compare Equation 3 and Equation 4:
3.1×10−41.2×10−4=k(0.40)a(0.05)b(0.20)ck(0.30)a(0.10)b(0.10)c
Simplifying gives:
3.11.2=(0.40)a(0.20)c(0.30)a(0.10)c
This leads to:
3.11.2≈(0.40)a(0.20)1(0.30)a(0.10)1
Solving for a:
2.58≈(0.400.30)a⋅2⟹a≈1
From our calculations:
- a=1
- b=0
- c=1
The overall rate law is:
Rate=k(CH3COCH3)1(Br2)0(H+)1
This indicates that the reaction is first order with respect to acetone and hydrogen ions, and zero order with respect to bromine.
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - A2ZCHAPTER - CHEMICAL KINETICSEXERCISE - AIIMS Questions
24 Videos
- CHEMICAL KINETICSBOOK - A2ZCHAPTER - CHEMICAL KINETICSEXERCISE - Assertion-Reasoning Questions
6 Videos
- CHEMICAL KINETICSBOOK - A2ZCHAPTER - CHEMICAL KINETICSEXERCISE - Section B - Assertion Reasoning
21 Videos
- BIOMOLECULESBOOK - A2ZCHAPTER - BIOMOLECULESEXERCISE - Section D - Chapter End Test
30 Videos
- COORDINATION COMPOUNDSBOOK - A2ZCHAPTER - COORDINATION COMPOUNDSEXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
The bromination of acetone that occurs in acid solution is represented by
CH3COCH3(aq.)+Br2(aq.)→CH3COCH2Br(aq.)+H+(aq.)+Br−(aq.)
These kinetic data were obtained for given reaction concentration
Initial concentration
Initial concentrationInitial rate ofdisappearance[CH3COCH3][Br2][H+]of Br20.300.050.055.0×10−50.300.100.55.0×10−50.300.100.101.0×10−40.900.050.103.0×10−4
Based on these data, order of reaction will be -
View SolutionThe bromination of acetone that occurs in acid solution is represented by
CH3COCH3(aq.)+Br2(aq.)→CH3COCH2Br(aq.)+H+(aq.)+Br−(aq.)
These kinetic data were obtained for given reaction concentrations :
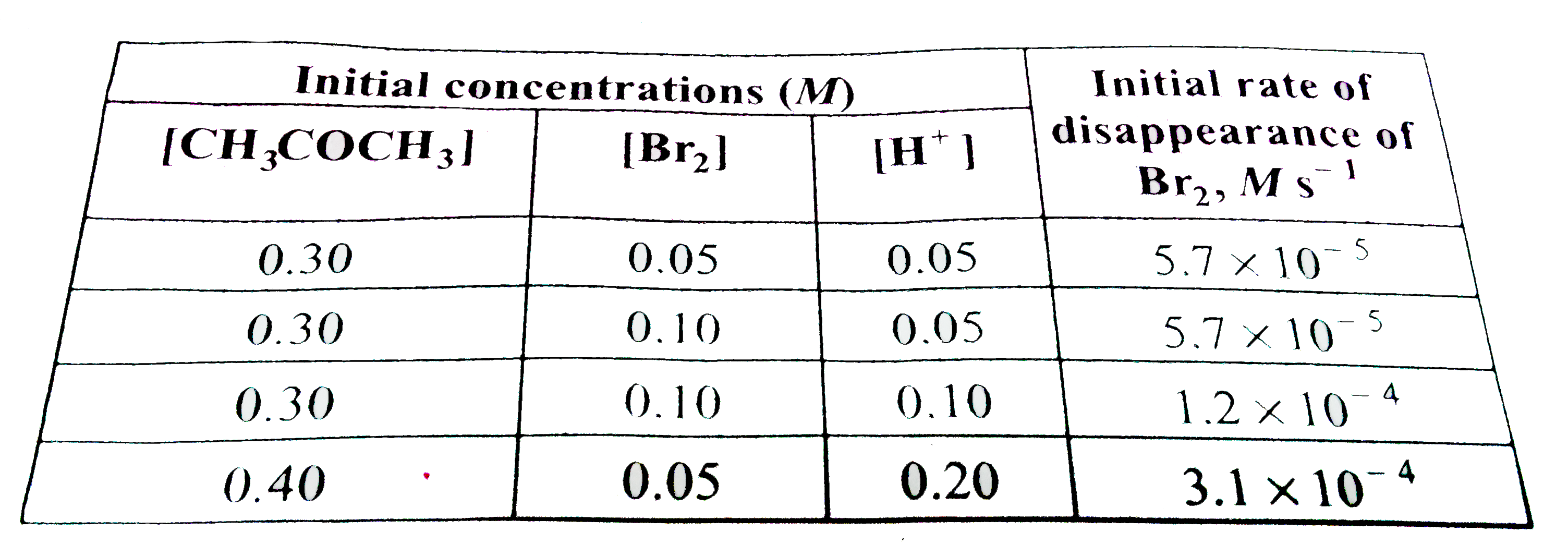
Based on these data, rate equations is :
View Solution
Knowledge Check
The bromination of acetone that occurs in acid solution is represented by
CH3COCH3(aq.)+Br2(aq.)→CH3COCH2Br(aq.)+H+(aq.)+Br−(aq.)
These kinetic data were obtained for given reaction concentrations :

Based on these data, rate equations is :ARate=k[CH3COCH3][Br2][H+]2BRate=k[CH3COCH3][Br2][H+]CRate=k[CH3COCH3[H+]DRate=k[CH3COCH3[Br2]The bromination of acetone that occurs in acid solution is represented by this equation.
CH3COCH3(aq)+Br2(aq)→CH3COCH2Br(aq)+H+(aq)+Br−(aq)
These kinetic data were obtained for given reaction concentrations.
[CH3COCH3][Br2][H+]rate of disappearance of Br2,(Ms−1) 0.300.050.05 5.7×10−5 0.300.100.05 5.7×10−5 0.300.100.10 1.2×10−4 0.400.050.20 3.1×10−4
Based on these data, the rate equation isARate=k[CH3COCH3][Br2][H+]2BRate=k[CH3COCH3][Br2][H+]CRate=k[CH3COCH3][H+]DRate=k[CH3COCH3][Br2]The bromination of acetone that occurs in acid solution is represented by this equation
CH3COCH3(aq)+Br2(aq)→CH3COCH2Br(aq)+H+(aq)+Br−(aq)
These kinetic data were obtained for given reaction concentrations .Initial concentrations , M
[CH3COCH3][Br2][H+]0.300.050.050.300.100.050.300.100.100.400.050.20
Initial rate , disappearance of Br2,Ms−1A5.7×10−5B5.7×10−5C1.2×10−4D3.1×10−4
The bromination of acetone which occurs in acid solution is represented by the equation:
CH3COCH3(aq)+Br2(aq)→CH3COCH2Br(aq)+H+(aq)+Br−1(aq)
The following kinetic data was obtained for the given reaction concentration:

Initial rates of disappearance of of Br2(Ms−1
5.7×10−5,5.7×10−5
1.2×10−4,3.1×10−4
Based on these data, the rate equation is:
View SolutionConisder the reaction represented by the equation:
CH3Cl(g)+H2O(g)→CH3OH(g)+HCl(g)
These kinetics data were obtained for the given reaction concentrations:
Initial conc (M)Initial rate of disappearance[CH3Cl][H2O]of CH3Cl(Ms−1)0.20.210.40.220.40.48
Order with respect to [CH3Cl] will be
View SolutionConisder the reaction represented by the equation:
CH3Cl(g)+H2O(g)→CH3OH(g)+HCl(g)
These kinetics data were obtained for the given reaction concentrations:
Initial conc (M)Initial rate of disappearance[CH3Cl][H2O]of CH3Cl(Ms−1)0.20.210.40.220.40.48
The rate law for the reaction will be
View SolutionConisder the reaction represented by the equation:
CH3Cl(g)+H2O(g)→CH3OH(g)+HCl(g)
These kinetics data were obtained for the given reaction concentrations:
Initial conc (M)Initial rate of disappearance[CH3Cl][H2O]of CH3Cl(Ms−1)0.20.210.40.220.40.48
Unit of rate constant will be
View SolutionConisder the reaction represented by the equation:
CH3Cl(g)+H2O(g)→CH3OH(g)+HCl(g)
These kinetics data were obtained for the given reaction concentrations:
Initial conc (M)Initial rate of disappearance[CH3Cl][H2O]of CH3Cl(Ms−1)0.20.210.40.220.40.48
Overall order of the reaction will be
View Solution
