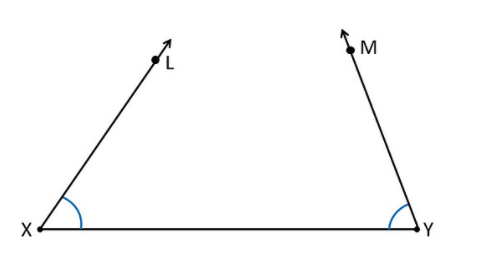
To construct a triangle, given its perimeter and its two base angles.
Draw a line segment XY equal to AB+AC+BC.

Make angle equal to ∠B from point X. Let the angle be ∠LXY.
Make an angle equal to ∠C from point Y. Let the angle be ∠MYX
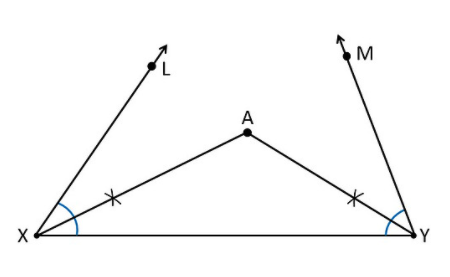
Bisect ∠LMX and ∠MYX. Let the bisector intersect at a point A.
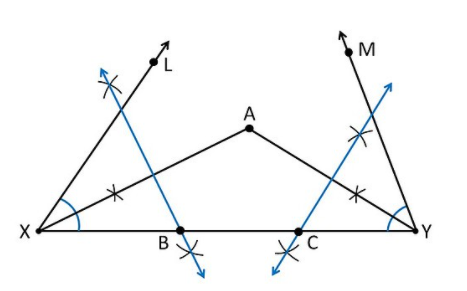
Make a perpendicular bisector of AX. Let it intersect XY at a point B.
Make a perpendicular bisector of AY. Let it intersect XY at point C.
Join AB and AC.
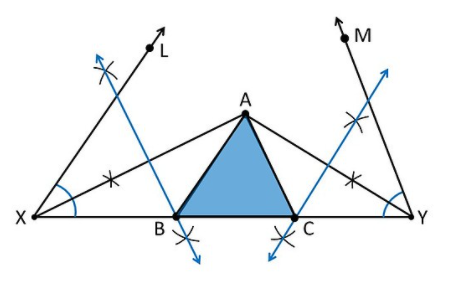
△ABC is the required triangle.
Topper's Solved these Questions
- CONSTRUCTIONSBOOK - NCERTCHAPTER - CONSTRUCTIONSEXERCISE - Example
1 Video
- CONSTRUCTIONSBOOK - NCERTCHAPTER - CONSTRUCTIONSEXERCISE - EXERCISE 11.1
5 Videos
- CIRCLESBOOK - NCERTCHAPTER - CIRCLESEXERCISE - EXERCISE 10.2
2 Videos
- COORDINATE GEOMETRYBOOK - NCERTCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 3.2
2 Videos
Similar Questions
Knowledge Check
It is not possible to construct a triangle when the lengths of its sides are
A3 cm, 4 cm , 5 cmB3 cm , 5 cm , 5 cmC5.3cm,2.2cm,3.1cmD9.3cm,5.2cm,7.4cm
To construct a triangle given its base, a base angle and the difference of the other two sides.
View SolutionExercise-11.1 Discussion|| Construction OF Triangles|| 1 - To construct a triangle|| Given its base|| A Base angle and Sum OF Other Two sides|| 2 - To construct A Triangle||given its base|| A Base angle and The Difference OF other Two sides ABAC
View SolutionConstruct △ABC, whose perimeter is 10cm and base angles are 60∘ and 44∘.
View SolutionConstruct an equilateral triangle, given its side and justify the construction.
View SolutionConstruct a ΔABC whose perimeter is 12cm and the base angles are 60∘ and 70∘. Justify the construction.
View SolutionIf the diameter of an excircle of a triangle is equal to its perimeter then the triangle is
View SolutionThe ratio of the product of the sides of an equilateral triangle to its perimeter is equal to the ratio of the product of the sides of another equilateral triangle to its perimeter. Then the triangles are
View Solution
