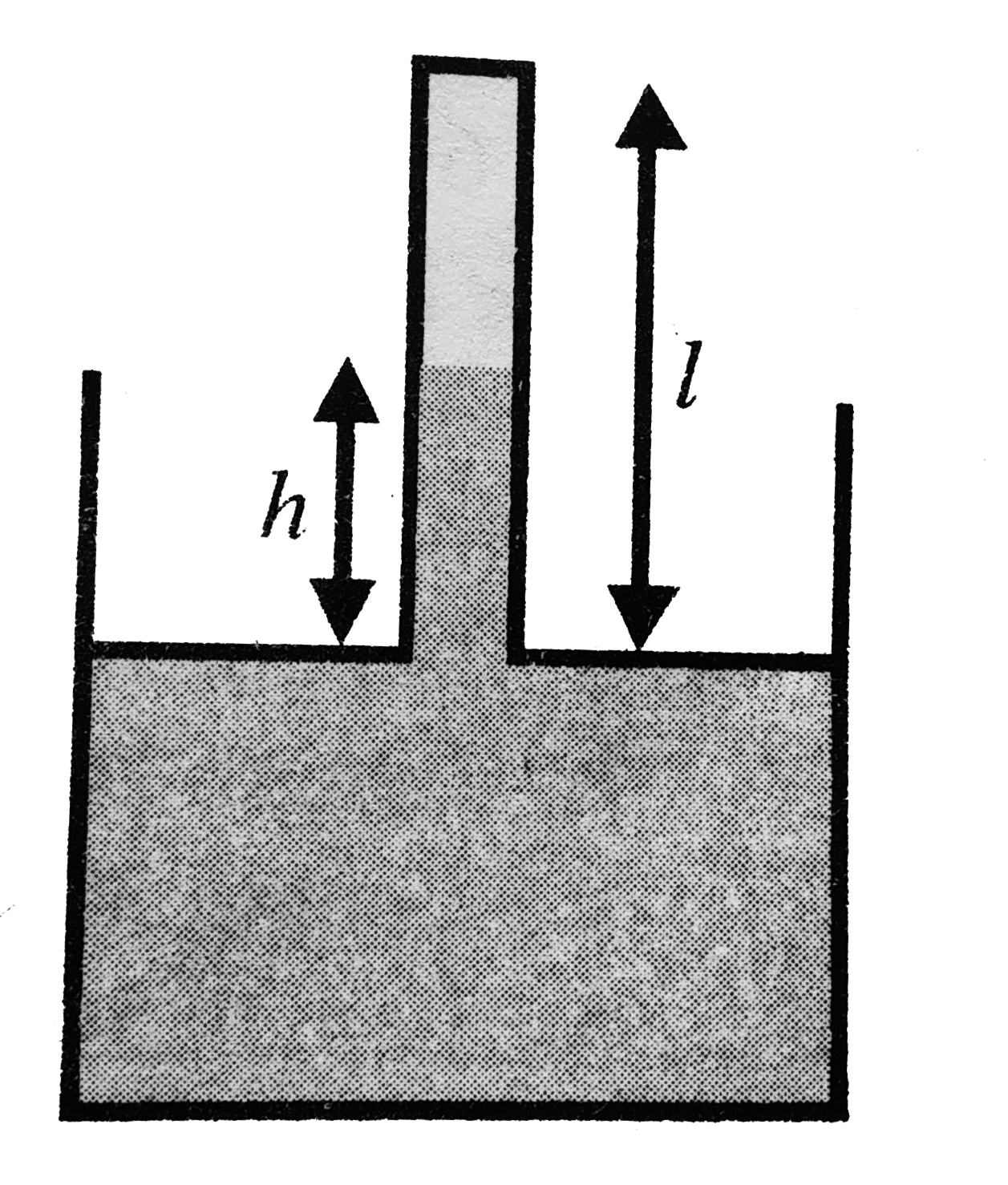
Two vertical plates submerged partially in a wetting liquid form a wedge with a very small angle $δ φ$ . The edge of this wedge is vertical. The density of the liquid is $ρ$ , its surface tension is $α$ , the contact angle is $θ$ . Find the height $h$ , to which the liquid rises, as a function of the distance $x$ from the edge.

Video Solution

Text Solution

Verified by Experts

Let $h$ be the height of the water level at a distance $x$ from the edge. The the total energy of water in the wedge above the level outside is.
$E = \int x δ φ . d x . h . ρ g (\frac{h}{2}) - 2 \int d x . h . α cos 0$
= $\int d x \frac{1}{2} x ρ g δ φ (h^{2} - 2 \frac{2 α cos θ}{x ρ g δ φ} h)$
= $\int d x \frac{1}{2} x ρ g δ φ [(h - \frac{2 α cos θ}{x ρ g δ φ}) - \frac{4 α^{2} {cos}^{2} θ}{x^{2} ρ^{2} g^{2} δ φ^{2}}]$
This is minimum when $h = \frac{2 α cos θ}{x ρ g δ φ}$ .
.

|

Updated on:21/07/2023

Class 12 PHYSICS THERMODYNAMICS AND MOLECULAR PHYSICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two vertical plates submerged partially in a wetting liquid form a wedge with a very small angle $δ φ$ . The edge of this wedge is vertical. The density of the liquid is $ρ$ , its surface tension is $α$ , the contact angle is $θ$ . Find the height $h$ , to which the liquid rises, as a function of the distance $x$ from the edge.
A $\frac{2 T}{ρ g x (δ θ)}$
B $\frac{2 T}{ρ g x}$
C $\frac{2 T (δ θ)}{ρ g x}$
D $\frac{T}{ρ g x (δ θ)}$
Question 2 - Select One or More
A cappillary tube of radiue $r$ is lowered into water whose surface tension is $α$ and density $d$ . The liquid rises to a height. Assume that the contact angle is zero. Choose the correct statement (s):
AMagnitude of work done by force of surface tension is $\frac{4 π α^{2}}{d g}$
BMagnitude of work done by force of surface tension is $\frac{2 π α^{2}}{d g}$
CPotential energy acquired by the water is $\frac{2 π α^{2}}{d g}$
DThe amount of heat developed is $\frac{2 π α^{2}}{d g}$
Question 3 - Select One
Two parallel glass plates separated by a small distance x ar dipped partly in a liquid of density ' d keeping them vertical .The surface tension of the liquid is T and angle of contact is $θ$ . What is the rise of the liquid between the plates due to capillarity ?
A $\frac{2 T cos θ}{x d g}$
B $\frac{2 T}{x d g cos θ}$
C $\frac{T cos θ}{x d g}$
D $\frac{T cos θ}{x d}$

IE IRODOV, LA SENA & SS KROTOV-THERMODYNAMICS AND MOLECULAR PHYSICS-Liquids Capillary Effects

Home
Ask Doubt
Profile