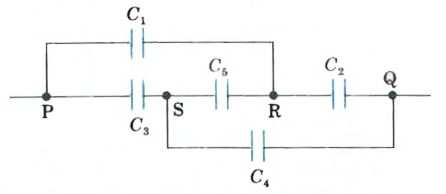
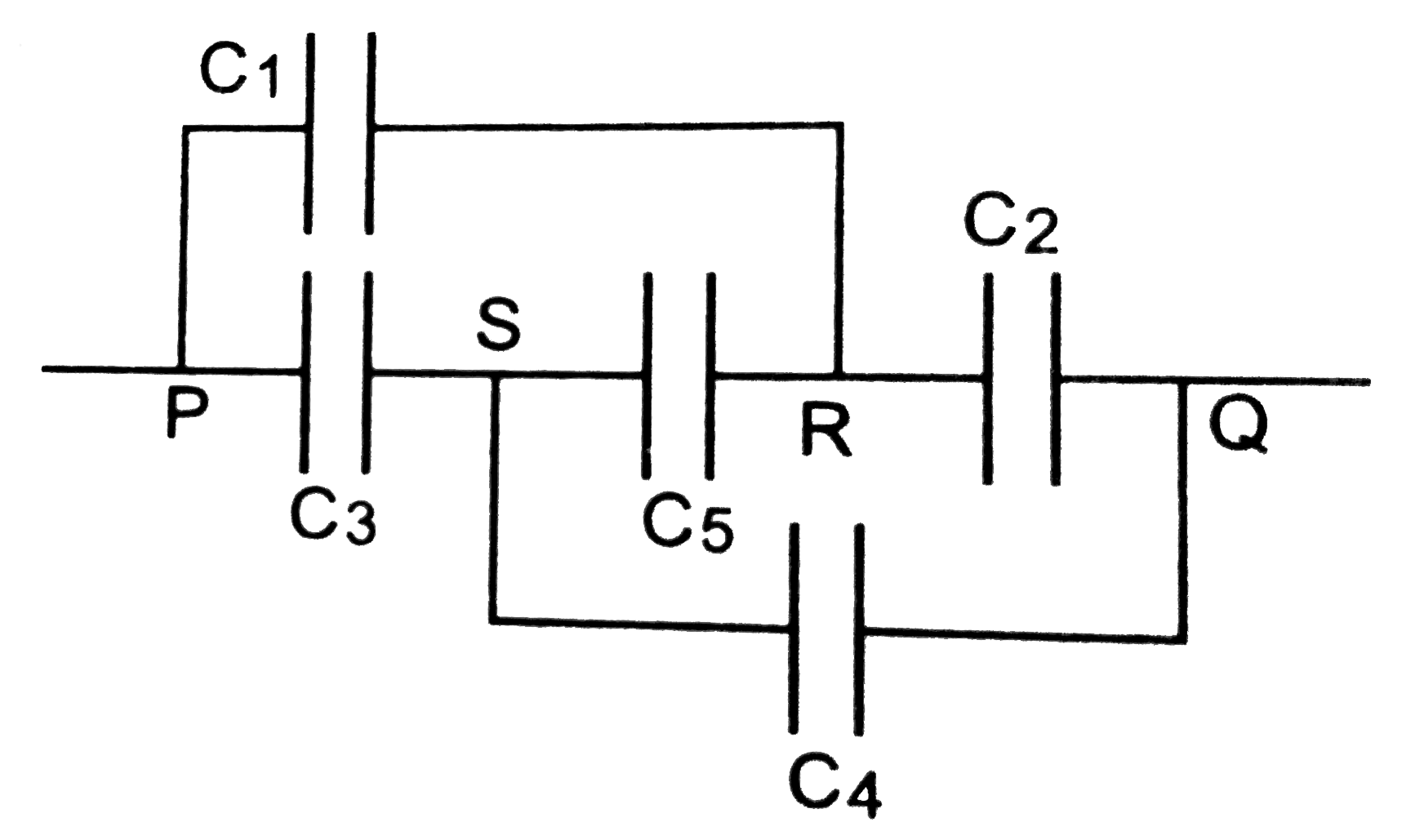
In fig, the values of capacitances are as follow
C1=C2=C3=C4=4μF,C5=5μF Calculate the equivalent capacitance between the points P and Q. If a battery of 10 volt is connected between these points, what will be the charge on each capacitor ?
Video Solution
Text Solution
The given circuit can be redrawn as shown in Fig.
Clearly the capacitors form wheatstone bridge arrangement.
As C1=C2=C3=C4∴C1C2=C3C4
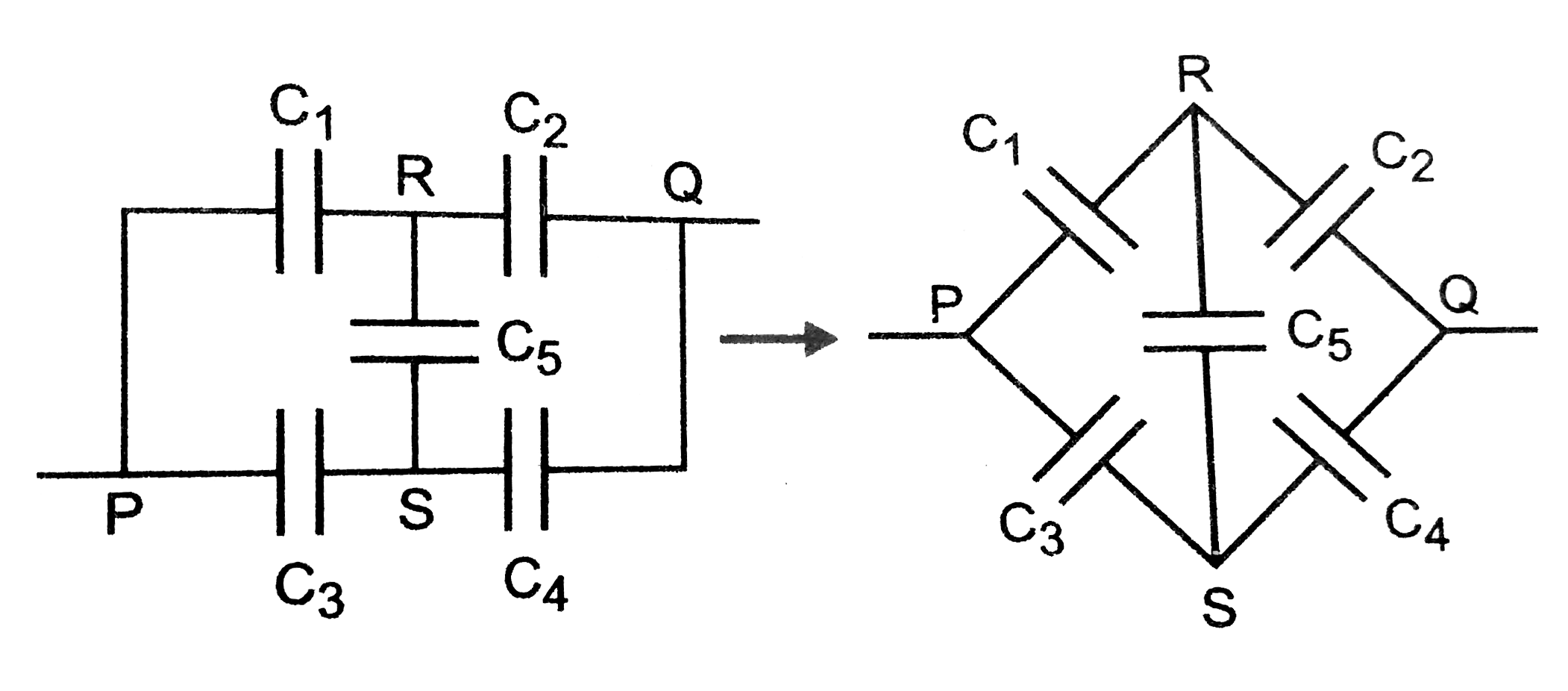
Hence the bridge is balanced. The points R and S are at the same potential. No charge can accumulate on C5, which thus becomes ineffective.
Between P and Q, two series combinations (C1,C2)and(C3,C4) are connected in parallel. If C' and C" are the equivalent capacitances of these combinations, then from
1C'=1C1+1C2=14+14=24,C'=2μF
Similarly,C=2μF
Hence equivalent capacitance between P and Q is C=C'+C=2+2=4μF
As pot diff. between P and Q is V = 0 volt and equivalent capacitance of C1andC2 in series is 2μF.
∴ Charge on each of capacitors C1andC2 is =2×10=20μC.
Similarly, charge on each of capacitors C3andC4 is also 20μC. charge on C5=0
|
Similar Questions
Knowledge Check
In the arrangement C1=10μ,FC2=10μF and C3=20μF . What is the equivalent capacitance ( in μF ) between points A and B ?
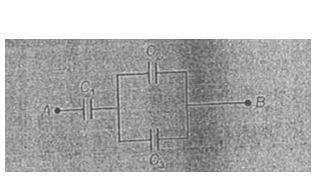 A7.5B10C12D15
A7.5B10C12D15The equivalent capacitance between the points A and B of five identical capacitors each of capacity C1 will be
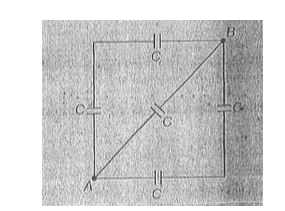 AC2B5 CCC5D2 C
AC2B5 CCC5D2 CThe equivalent capacitance of the network given below is 1μF. The value of 'C' is.
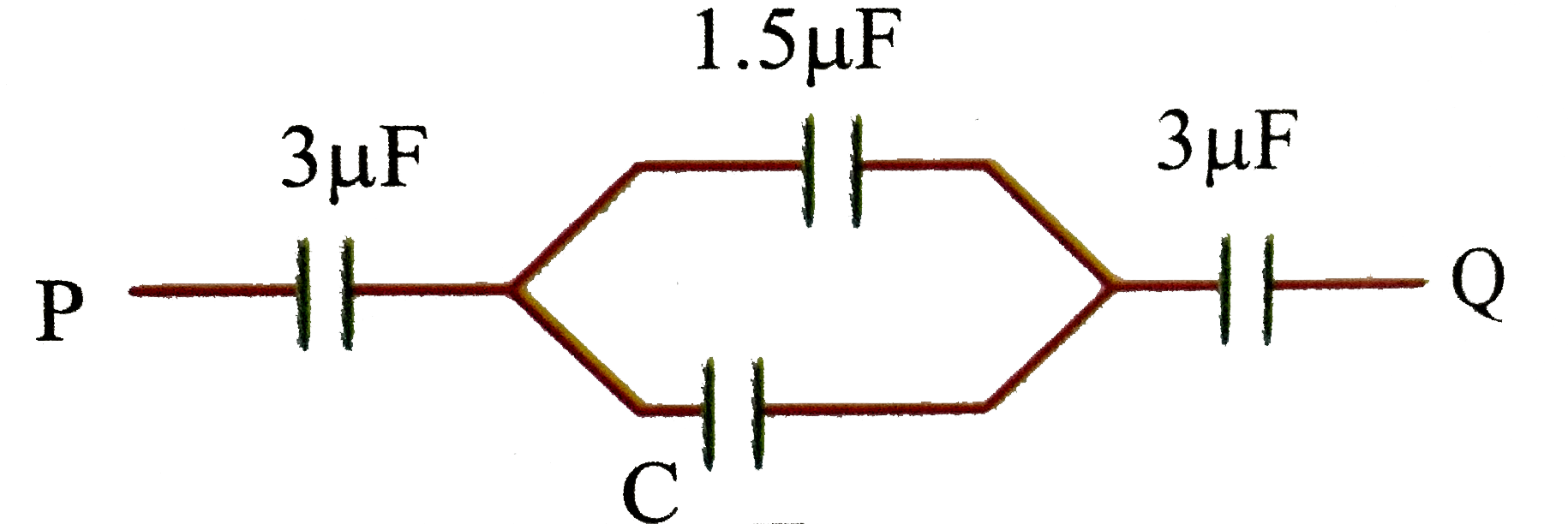 .A3μFB1.5μFC2.5μFD1μF
.A3μFB1.5μFC2.5μFD1μF
If C1=3pF and C2=2pF, calculate the equivalent capacitance of the network shown in Fig between points A and B.
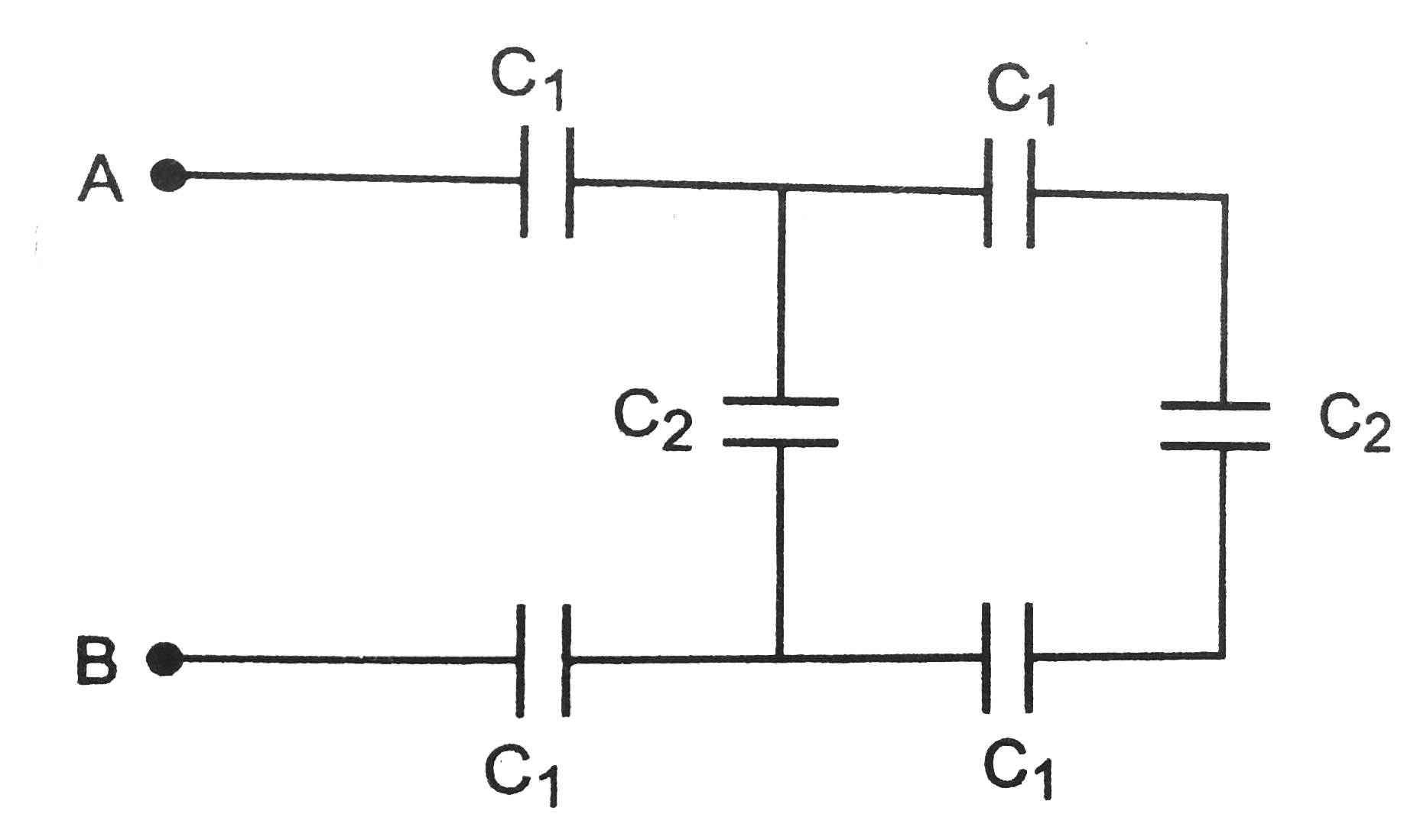
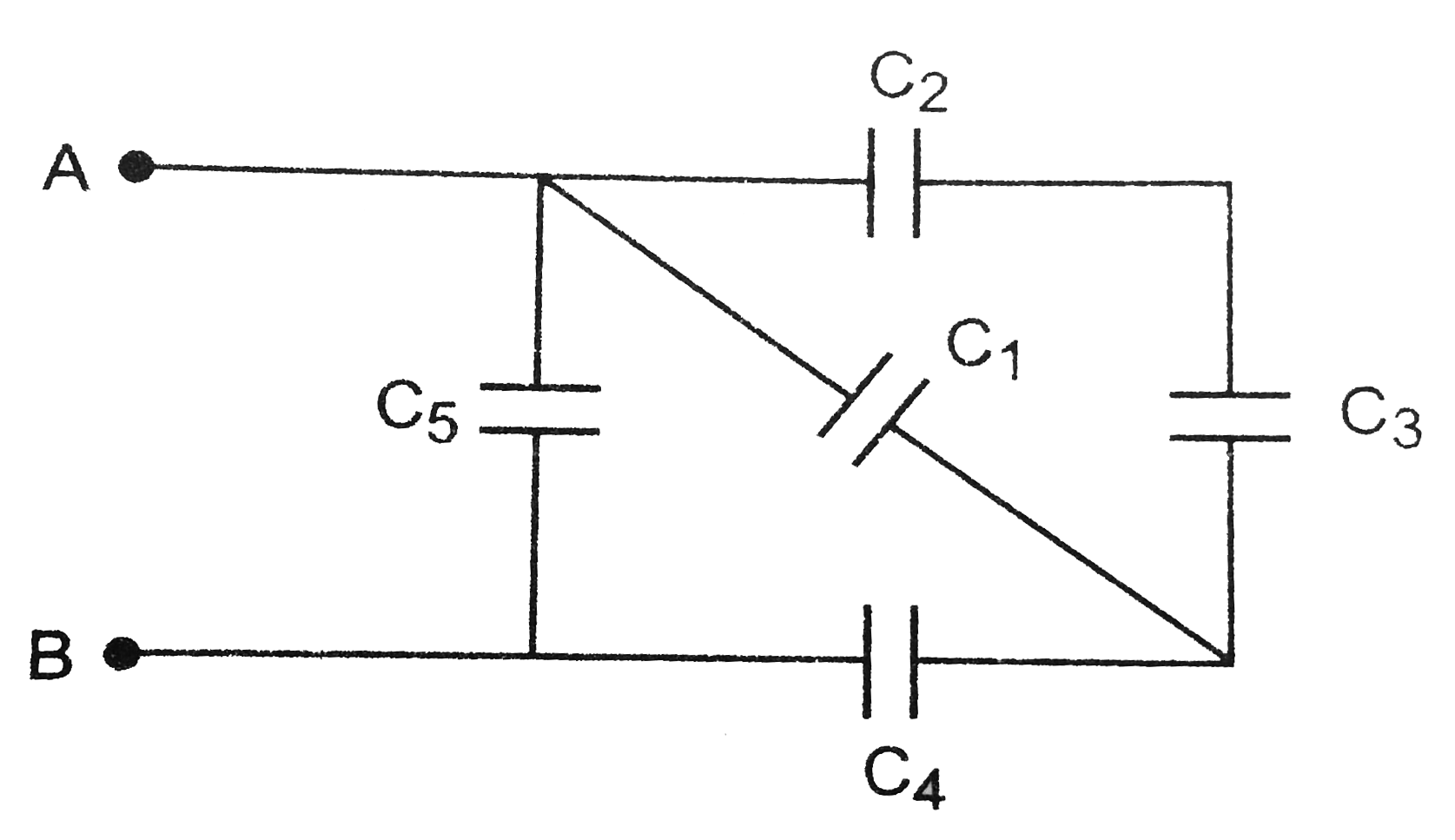
View SolutionFive capacitors of capacitances C1=C5=1μF,C2=C3=C4=2μF are connectes as shown in Fig. Calculate equivalent capacitance of the system between points A and B.
View SolutionTake C1=4.0μFandC2=6.0μF in figure. Calculate the equivalent capacitance of the combination between the points indicated.

View Solution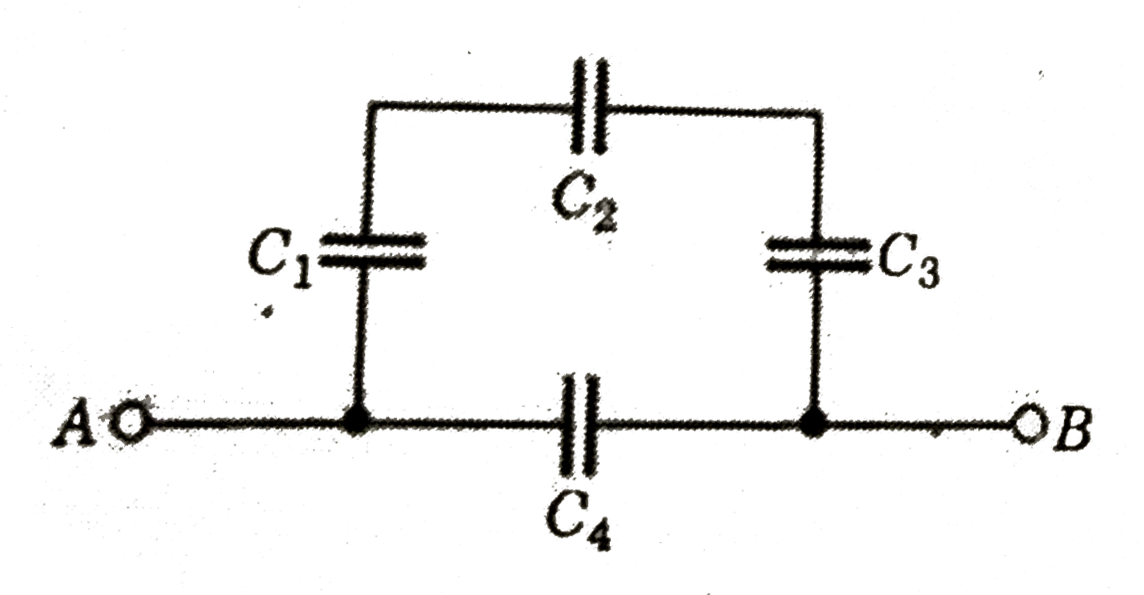
In the network shown in figure. C1=C2=C3=C4=10μF. Find th equivalent capacitance between the points A and B, what will be the charge on each capacitor?
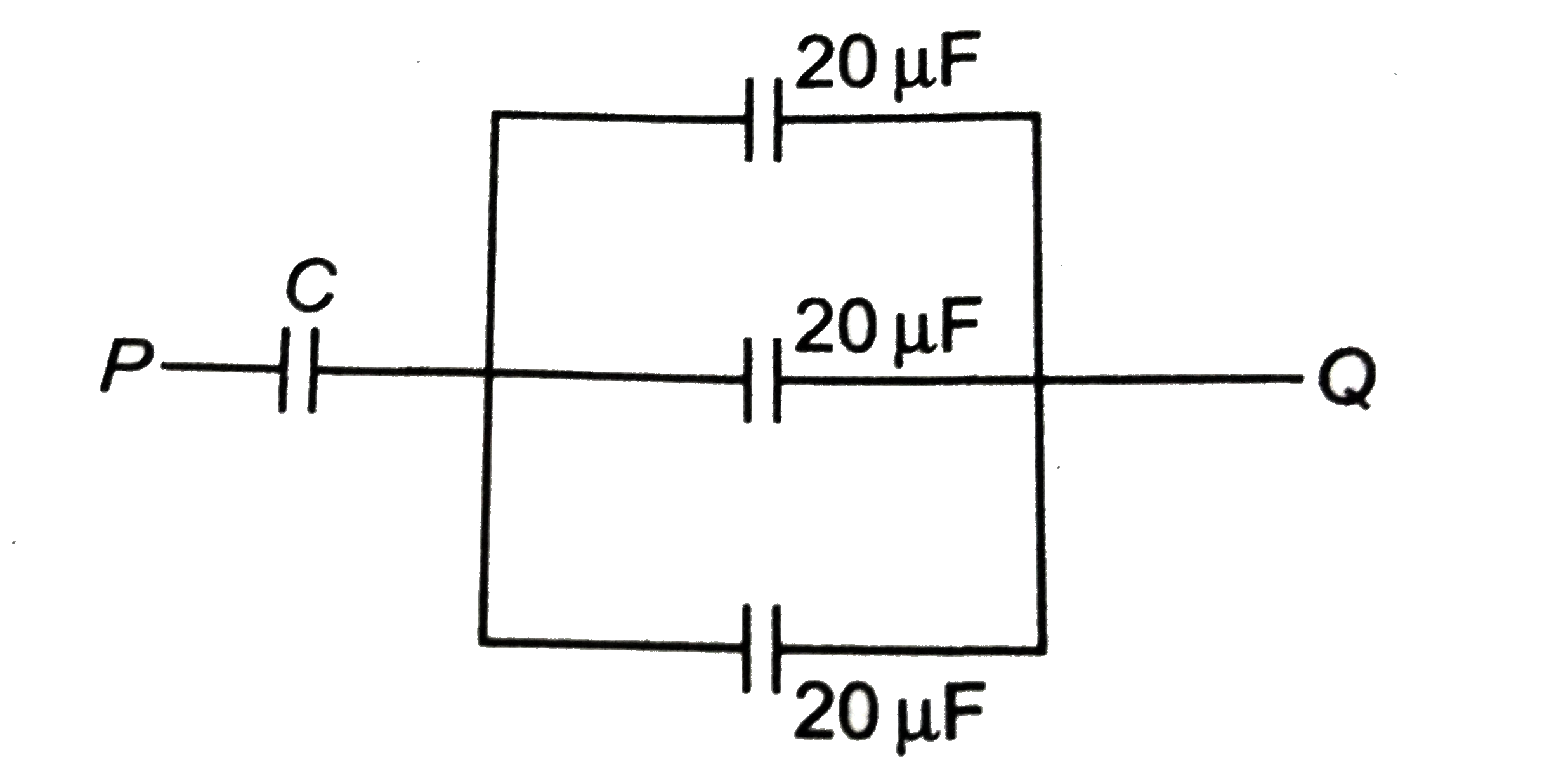
View SolutionIf the equivalent capacitance between points P and Q of the combination of the capacitors hown in figure below is 30μF, the capacitor C is
View Solution