Define surface density of charge and potential of a charged and potential of a charged spherical conductor. Establish a relation between them.
Video Solution
Text Solution
Surface density of charge is defined as the charge per unit area of a charged conductor . If r is the radius of a spherical conductor having charge q, then its surface density of charge,
σ=q4πr2 …(i)
Electric potentail of the spherical charged condutor,
V=q4π∈0rorq=4π∈0r×V
Putting this value in (i), we get
σ=4π∈0rV4πr2=∈0Vr
|
Similar Questions
Knowledge Check
Electric potential at the center ofa charged hollow spherical conductor is
AzeroBtwice as that on the surfaceChalf of that on the surfaceDsame as that on the surfaceInside a hollow charged spherical conductor, the potential
Ais constantBvaries directly as the distance from the centreCvaries inversely as the disatnce from the centreDvaries inversely as the square of the distance from the centresInside a charged hollow spherical conductor. the potential :-
Ais constantBvaries directly as the distance from the centreCvaries inversely as the distance from the centreDvaries inversely as the square of the distance from the centre
The potential energy of a charged conductor of charge (q) and potential (v) is given by
View SolutionThe electric potential at the centre of a charged conductor is
View SolutionThe surface charge density of an irregular shaped conductor is
View Solution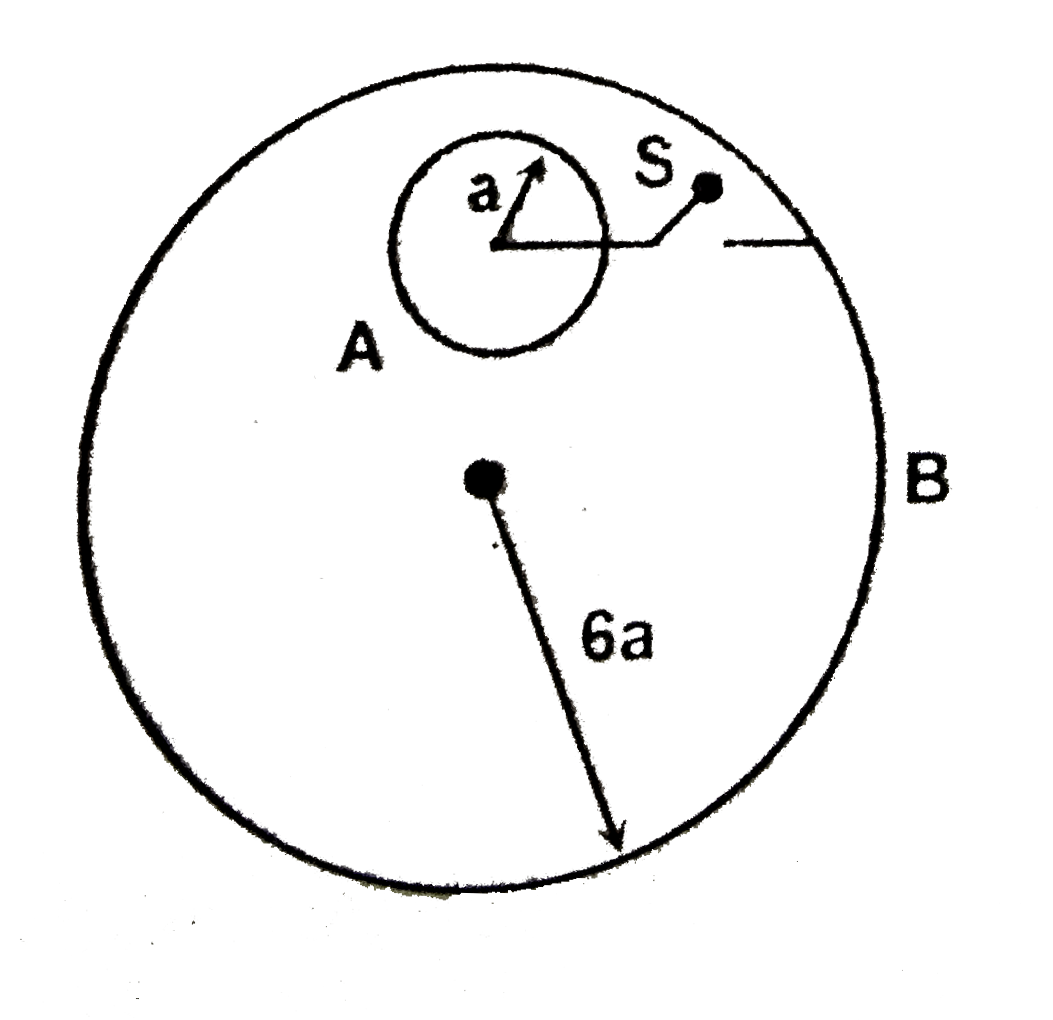
A small spherical conductor 'A' of radias 'a' is initially charged to a potential 'V' and then placed inside an uncharged spherical conducting shell 'B' of radius 6a as shown. The potential of the spherical conductor 'A' after closing the switch S is
View SolutionThe electric intensity at a point near a charged conductor of surface charge density σ is
View Solution