A small source of light is below the surface of a liquid of refractive index .In order to cut off all the light comingout of liquid surface,minimum diameter of the disc placed on the surface of liquid is
The correct Answer is:C
To solve the problem step by step, we need to find the minimum diameter of the disc that can cut off all the light coming out of the liquid surface.
Step 1: Understand the setup
We have a light source located 4 meters below the surface of a liquid with a refractive index of n=53. We need to determine the minimum diameter of a disc that can block all the light rays emerging from the surface of the liquid.
Step 2: Apply Snell's Law
Using Snell's law, we can relate the angles of incidence and refraction at the surface of the liquid. Snell's law states:
n1sinθ1=n2sinθ2
Here, n1=53 (refractive index of the liquid), n2=1 (refractive index of air), θ1 is the angle of incidence (in the liquid), and θ2 is the angle of refraction (in air).
At the critical angle C, the light will just graze the surface:
53sinC=1⋅sin90∘
This simplifies to:
sinC=35
Step 3: Calculate the distance of the light rays
Using the geometry of the situation, we can find the horizontal distance R from the point directly above the light source to the edge of the disc. From the right triangle formed, we have:
sinC=R√R2+H2
where H=4m (the depth of the light source).
Substituting the value of sinC:
35=R√R2+42
Step 4: Solve for R
Squaring both sides gives:
(35)2=R2R2+16
This results in:
925=R2R2+16
Cross-multiplying yields:
9(R2+16)=25R2
Expanding and rearranging gives:
9R2+144=25R2⟹16R2=144⟹R2=9⟹R=3m
Step 5: Calculate the diameter of the disc
The minimum diameter D of the disc that can cut off all the light is twice the radius R:
D=2R=2×3=6m
Final Answer
The minimum diameter of the disc placed on the surface of the liquid is 6m.
---
We have a light source located 4 meters below the surface of a liquid with a refractive index of n=53. We need to determine the minimum diameter of a disc that can block all the light rays emerging from the surface of the liquid.
Using Snell's law, we can relate the angles of incidence and refraction at the surface of the liquid. Snell's law states:
n1sinθ1=n2sinθ2
Here, n1=53 (refractive index of the liquid), n2=1 (refractive index of air), θ1 is the angle of incidence (in the liquid), and θ2 is the angle of refraction (in air).
53sinC=1⋅sin90∘
This simplifies to:
sinC=35
Using the geometry of the situation, we can find the horizontal distance R from the point directly above the light source to the edge of the disc. From the right triangle formed, we have:
sinC=R√R2+H2
where H=4m (the depth of the light source).
35=R√R2+42
Squaring both sides gives:
(35)2=R2R2+16
This results in:
925=R2R2+16
Cross-multiplying yields:
9(R2+16)=25R2
Expanding and rearranging gives:
9R2+144=25R2⟹16R2=144⟹R2=9⟹R=3m
The minimum diameter D of the disc that can cut off all the light is twice the radius R:
D=2R=2×3=6m
The minimum diameter of the disc placed on the surface of the liquid is 6m.
Similar Questions
In a tank filled with a liquid of refractive index 5/3, a point source of light is placed 2m below the surface of water. To cut off all light coming out of water from the source, what should be the minimum diameter of a disc, which should be placed over the source on the surface of water ?
View SolutionA jar of height h is filled wih a transparent liquid of refractive index μ, Fig. At the centre of the jar on the botom surface is a dot. Find the minimum diameter of a disc, such that when placed on the top surface symmetrically about the centre, the dot is invisible.
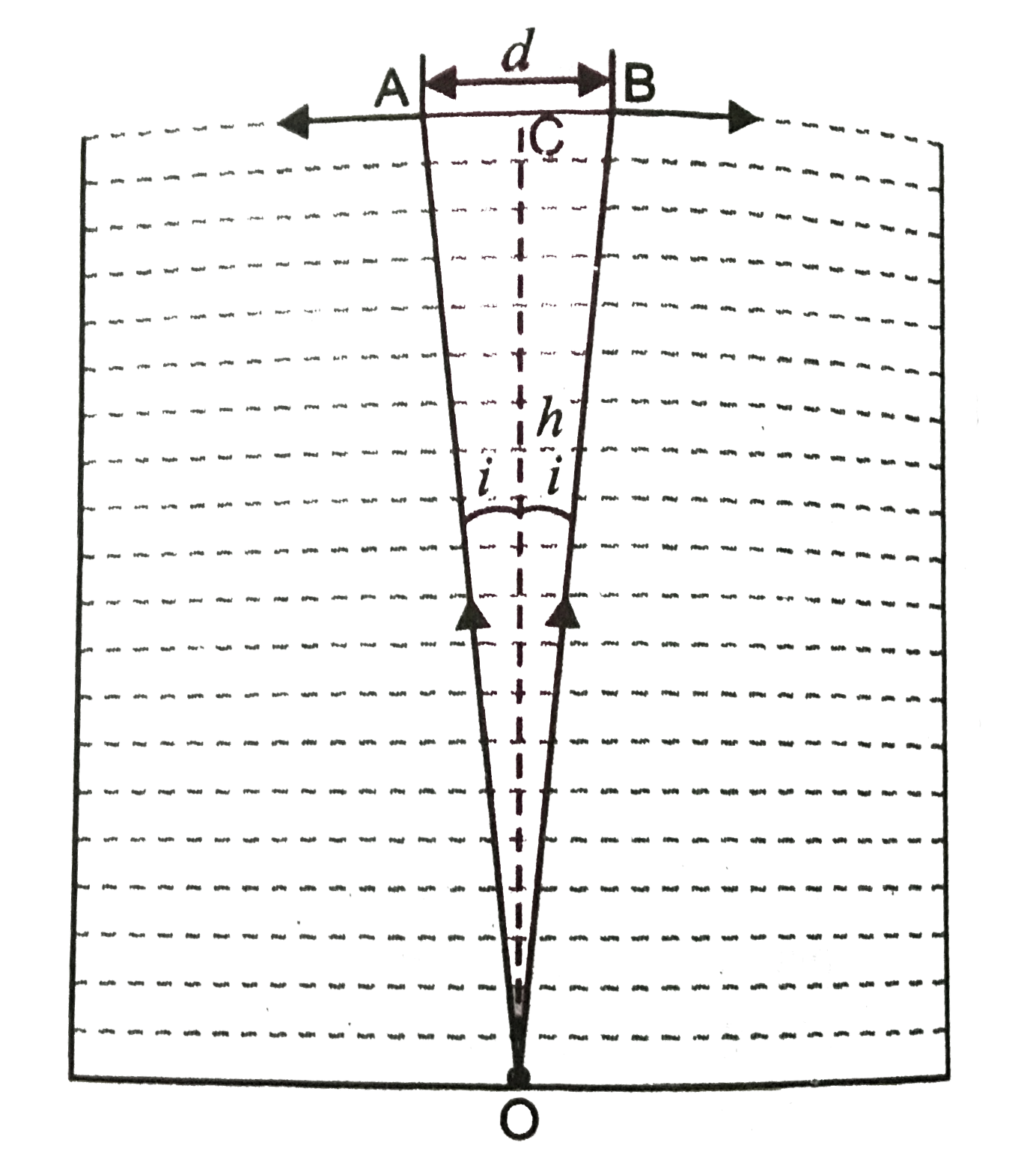 .
.
View Solution
Knowledge Check
A point source of light is placed 4m below the surface of a liquid of refractive index 5/3. The minimum diameter of a disc, which should be placed over the source, on the surface of the liquid to cut off all light out of water, is
A∞B6mC4mD3mA point source of light is placed 4 m below the surface of water of refractive index 5/3. The minimum diameter of a disc, which should be placed over the source, on the surface of water to cut-off all light coming out of water is
AinfiniteB6mC4mD3mA point soujrce of light is placed 4 m below the surface of water of refractive index 53. The minimum diameter of a disc, which should be placed over the source, on the surface of water to cut off alol light coming out of water is
AinfiniteB6mC4mD3m
A disc is placed on the surface of pond filled with liquid of refractive index 53. A source of light is placed 4m below the surface of liquid. Calculate the minimum area of the disc so that light does not come out of liquid.
View SolutionA point source of light S is placed at the bottom of a vessel containg a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of the disc lies vertically above the source S. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot at all be seen from above?
View SolutionA point light source is attached to the base of an empty trough. A transparent liquid of refractive index 1.35 is filled in the trough to a height of 50 cm. Calculate the total area on the surface of the liquid through which light will emerge.
View SolutionA point source of light is placed at a depth of h below the surface of water of refractive index μ.A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface . The minimum diameter of the disc is
View SolutionA point source of light is placed at a depth of h below the surface of water of refractive index μ. A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface. The minimum diameter of the disc is
View Solution