If a tunnel is cut at any orientation through earth, then a ball released from one end will reach the other end in time (neglect earth rotation)
84.6 minutes
42.3 minutes
8 minutes
depends on orientation
The correct Answer is:b
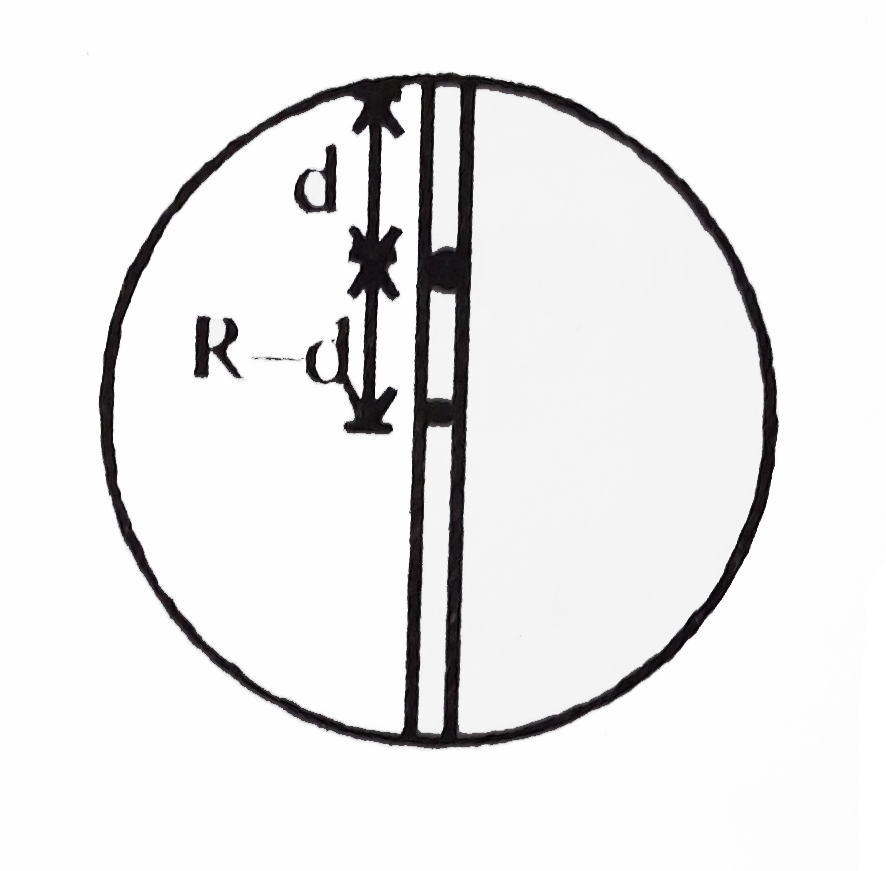
gd=g(1−dR)
gd=gRx
FR=−mgd⇒FR=−mgRx
T=2π√mRmg⇒T=2π√Rg
T=84.6min
t=T2⇒t=42.3 min.
Similar Questions
Imagine a tunnel dug along a diameter of the earth. Show that a particle dropped from one end of the tunnel executes simple harmonic motion. What is the time period of this motion? Assume the earth to be a sphere of uniform mass density (equal to its known average density=5520 kg m−3.)G=6.67×10−11Nm2kg−2.Neglect all damping forces.
View SolutionThe heat energy is carried by molecules from one end to other end of any medium in
View Solution
Knowledge Check
A tunnel has been dug through the centre of the earth and a ball is released in it. It will reach the other end of the tunnel after
A84.6 minB42.3 minC1 dayDWill not reach the other endA tunnel has been dug through the centre of the Earth and a ball is released in it. It will reach the other end of the tunnel after about
Aone dayBone hourC42 minD84 minHeat flows from one end of a steel spoon to the other end by
AConductionBConvectionCRadiationDBoth (a) and (c)
If a smooth tunnel is dug across a diameter of earth and a particle is released from the surface of earth, the particle oscillate simple harmonically along it
Time period of the particle is not equal to
View SolutionIf a smooth tunnel is dug across a diameter of earth and a particle is released from the surface of earth, the particle oscillate simple harmonically along it
Maximum speed of the particle is
View SolutionA smooth tunnel is dug along the radius of the earth that ends at the centre. A ball is released from the surface of earth along the tunnel. If the coefficient of restitution is 0.2 between the surface and ball, then the distance travelled by the ball before second collision at the centre is
View SolutionA tunnel is dug across the diameter of earth. A ball is released from the surface of Earth into the tunnel. The velocity of ball when it is at a distance R2 from centre of earth is (where R = radius of Earth and M = mass of Earth)
View SolutionA tunnel has been dug through the centre of the earth and a ball is released in it. It executes S.H.M. with time period
View Solution