Suppose a sphere of radius $a / 4$ is cut of the cube in previous problem. The center of the excised sphere is at the center of the cube. What is the moment of inertia $I_{e}$ of the resulting object, about the same axis ?
.

Video Solution

Text Solution

Verified by Experts

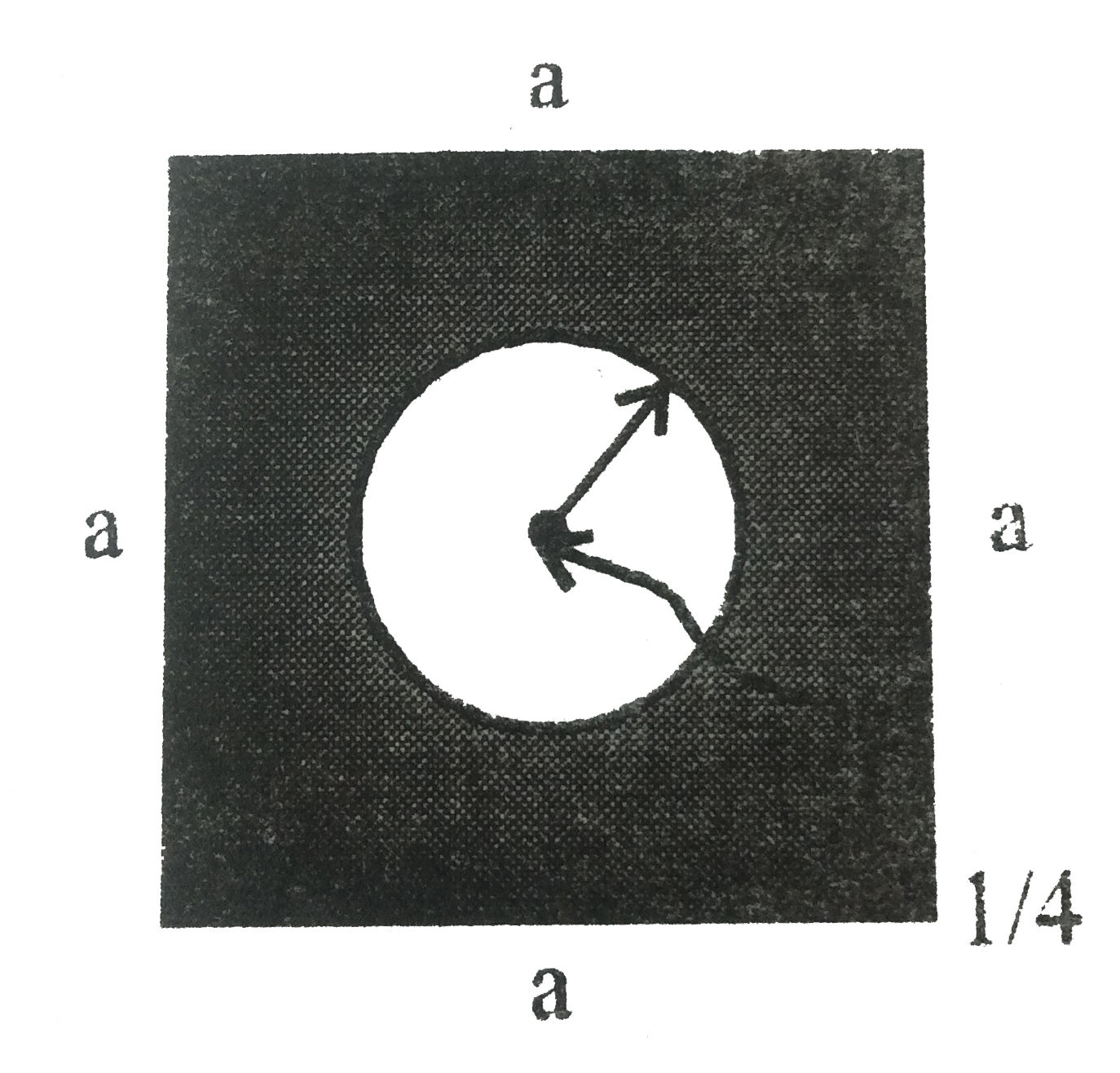The correct Answer is: $M a^{2} [\frac{1}{6} - \frac{π}{1920}]$

Let mass of soild cube $= m$
man of sphere radius $(a / 4), m' = \frac{m}{a^{3}} \times \frac{4}{3} π {(\frac{1}{4})}^{3} = \frac{m π}{48}$
$I_{e} = I_{radius} - I_{sphere} \Rightarrow I_{e} = \frac{m a^{2}}{6} - \frac{2}{5} m' {(\frac{a}{4})}^{2}$
$I_{e} = \frac{m a^{2}}{6} - \frac{2}{5} m \times \frac{m π}{48} \times \frac{a^{2}}{16}$
$I_{e} = m a^{2} [\frac{1}{6} - \frac{π}{1920}]$ .

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

Knowledge Check

Question 1 - Select One
What is the moment of inertia of solid sphere of density $ρ$ and radius $R$ about its diameter ?
A $\frac{105}{176} R^{2} ρ$
B $\frac{105}{176} R^{2} ρ$
C $\frac{176}{105} R^{2} ρ$
D $\frac{186}{105} R^{2} ρ$
Question 2 - Select One
The $M . I .$ of a rod about an axis through its center and perpendicular to it is $I_{0}$ . The rod is bent in the middle so that the two halves make an angle $θ$ . The moment of inertia of the bent rod about the same axis would be
A $I_{0} {sin}^{2} θ$
B $I_{0} {cos}^{2} θ$
C $I_{0}$
D $\frac{I_{0}}{2}$
Question 3 - Select One
A solid sphere and hollow sphere of the same material have mass. Then moment of inertia about the diameter is more for
ASolid sphere
BHollow sphere
Csame for both
Dnone

Recommended Questions

Home
Ask Doubt
Profile