A solid cylinder is relased from rest from the top of an inclined plane of inclination $60^{\circ}$ where friction coefficient varies with distance $x$ as $m i = \frac{2 - 3 x}{\sqrt{3}}$ . Find the distance travelled by the cylinder on incline before it starts slipping.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $1 / 3 m$

$μ = \frac{2 - 3 x}{\sqrt{3}}$
For translation motion
$m g \sin θ - f = m a$ …(1)
For rotational motion
$f \times R = I α \Rightarrow f \times R = (\frac{1}{2} m R^{2}) \frac{a}{R}$
$f = \frac{m a}{2}$
Solving (1) & (2) $a = \frac{2 g \sin θ}{3}$ & $f = \frac{g \sin θ}{3}$
when slipping starts max friction force will act so
$μ m g \cos θ = \frac{m g \sin θ}{3}$

$(\frac{2 - 3 x}{\sqrt{3}}) m g \times \frac{1}{2} = \frac{m g}{3} \times \frac{\sqrt{3}}{2}$
$2 - 3 x = 1$
$x = \frac{1}{3} m$ .

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

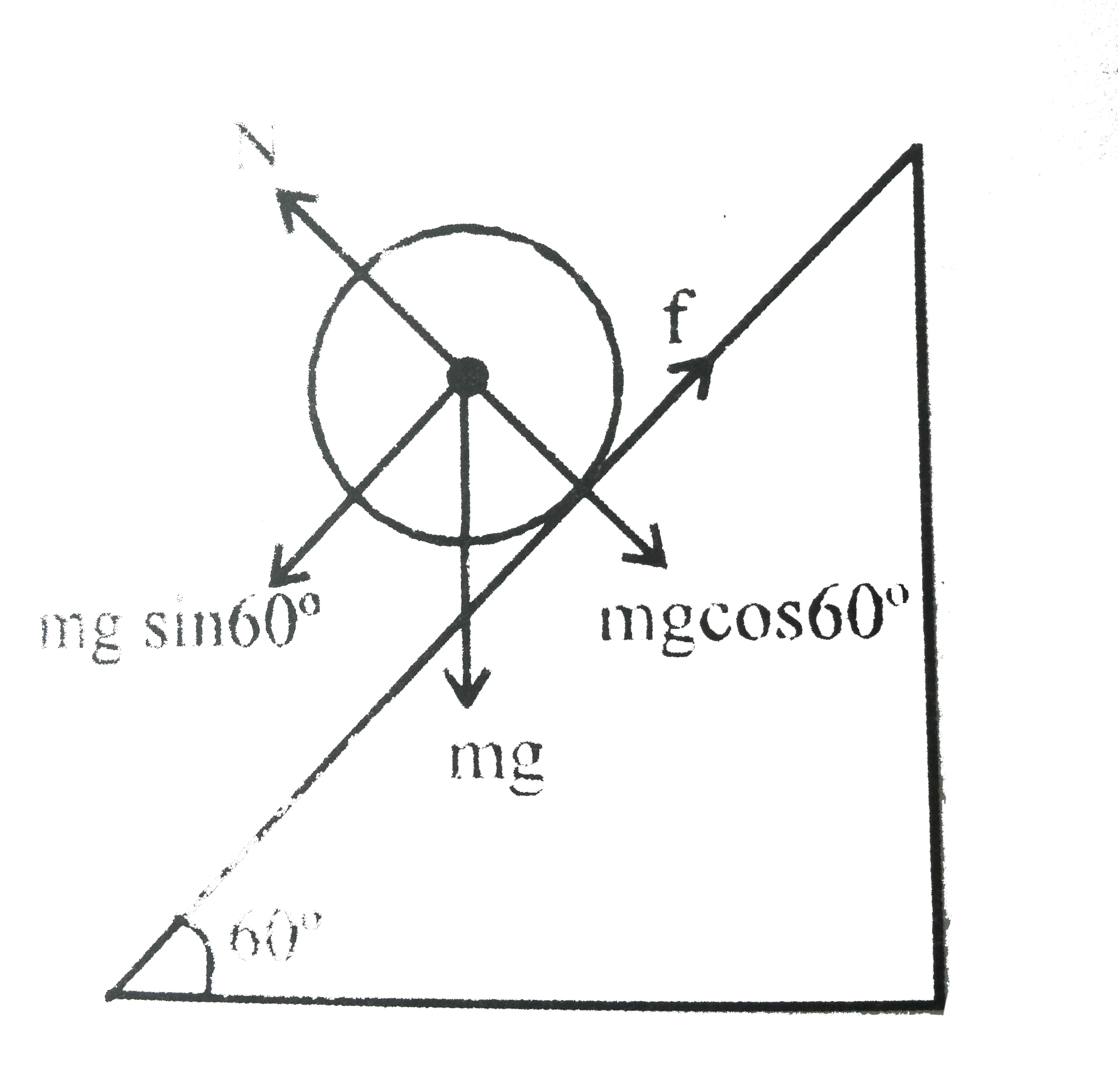
Similar Questions

Knowledge Check

Question 1 - Select One
A cylinder is released from rest from the top of an incline of inclination $θ$ and length $l$ . If the cylinder roles without slipping, its speed at the bottom
A $\sqrt{\frac{4 g l sin θ}{3}}$
B $\sqrt{\frac{3 g l sin θ}{2}}$
C $\sqrt{\frac{4 g l}{3 sin θ}}$
D $\sqrt{\frac{4 g sin θ}{3 l}}$
Question 2 - Select One
Two solid discs of radii $r$ and $2 r$ roll from the top of an inclined plane without slipping. Then
AThe bigger disc will reach the horizontal level first
BThe smaller disc will reach the horizontal level first
CThe time difference or reaching of the discs at the horizontal level will depend on the inclination of the plane
DBoth the discs will reach at the same time
Question 3 - Select One
A solid cylinder of mass 2 kg and radius 50 cm rolls up an inclined plane of angle of inclination $30^{\circ}$ . The centre of mass of the cylinder has speed of 4 m/s. The distance travelled by the cylinder on the inclined surface will be, [ take $g = 10 m / s^{2}]$
A $2.4 m$
B $2.2 m$
C $1.6 m$
D $1.2 m$

Recommended Questions

Home
Ask Doubt
Profile