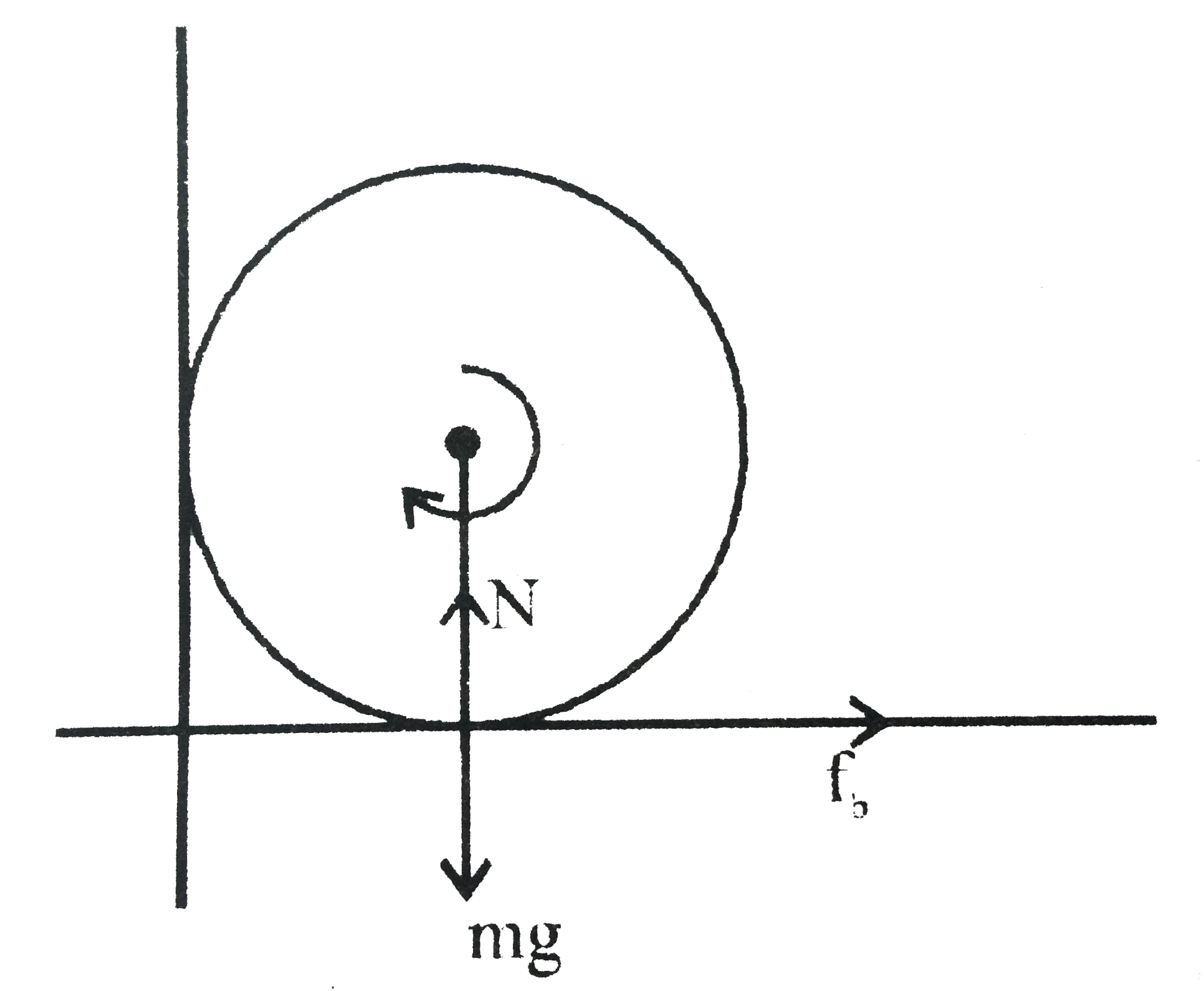
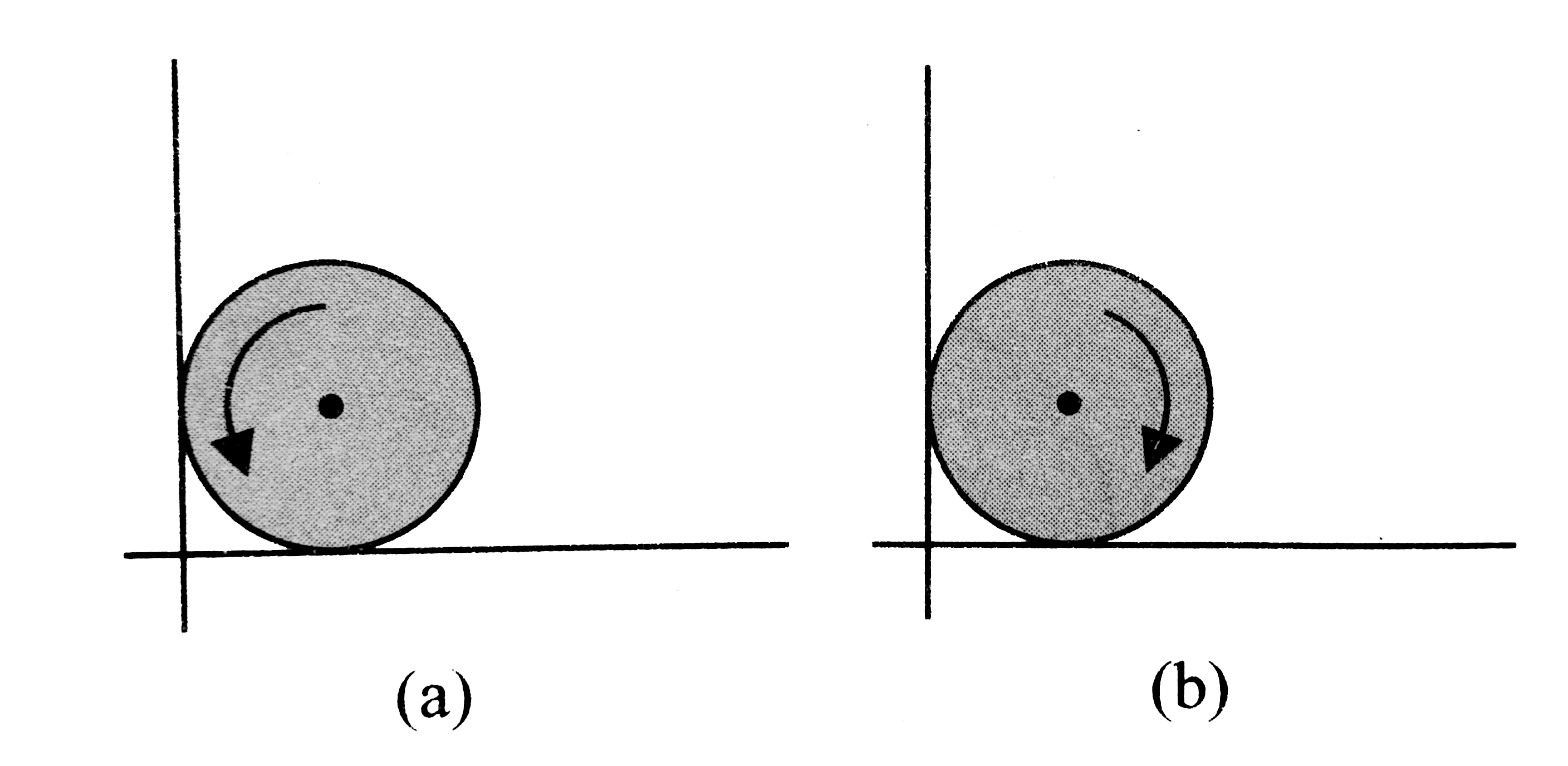
A sphere is placed rotating with its centre initially at rest in a corner as shown in figure (a) and (b). Coefficient of friction between all surfaces and the sphere is 13. Find the ratio of the frictional force fafb by ground in situations (a) and (b).
 .
.
1
9/10
10/9
none
The correct Answer is:B
taking equilibrium in vertical direction
mg=N1+f2 ….(1)
taking equilibrium in horizontal direction
N2=f1
therefore f2=μN2
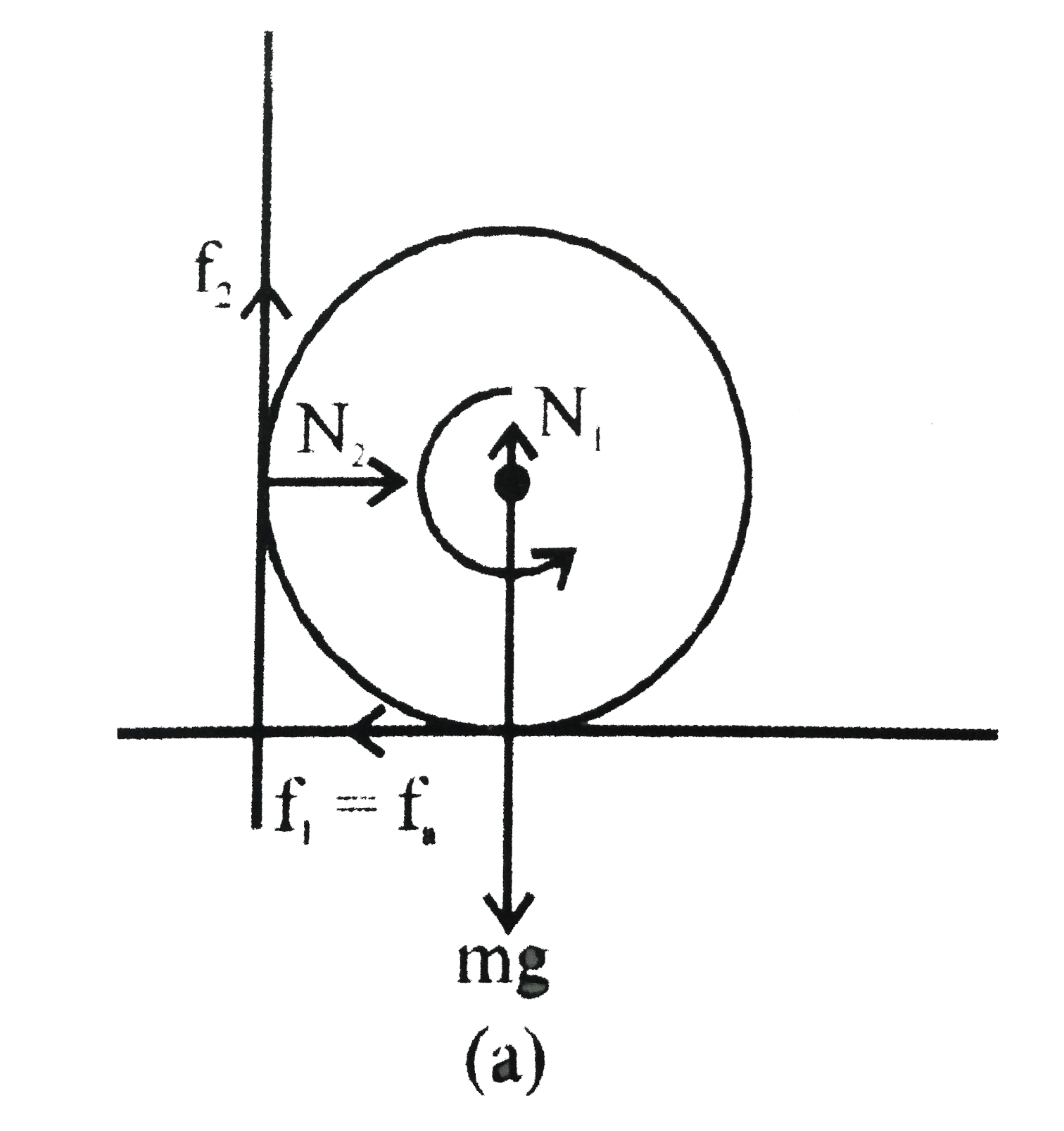
f2=μf1 …(2)
and f1=μN1⇒N1=f1μ ....(3)
From (1), (2) and (3)
mg=f1μ+μf1
f1=mg1μ+μ
fa=mg3+13
fa=310mg
fb−μN⇒fb=μmg⇒fb=mg3,sofafb=910.
 .
.
Similar Questions
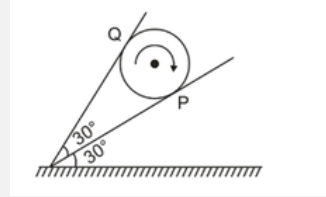
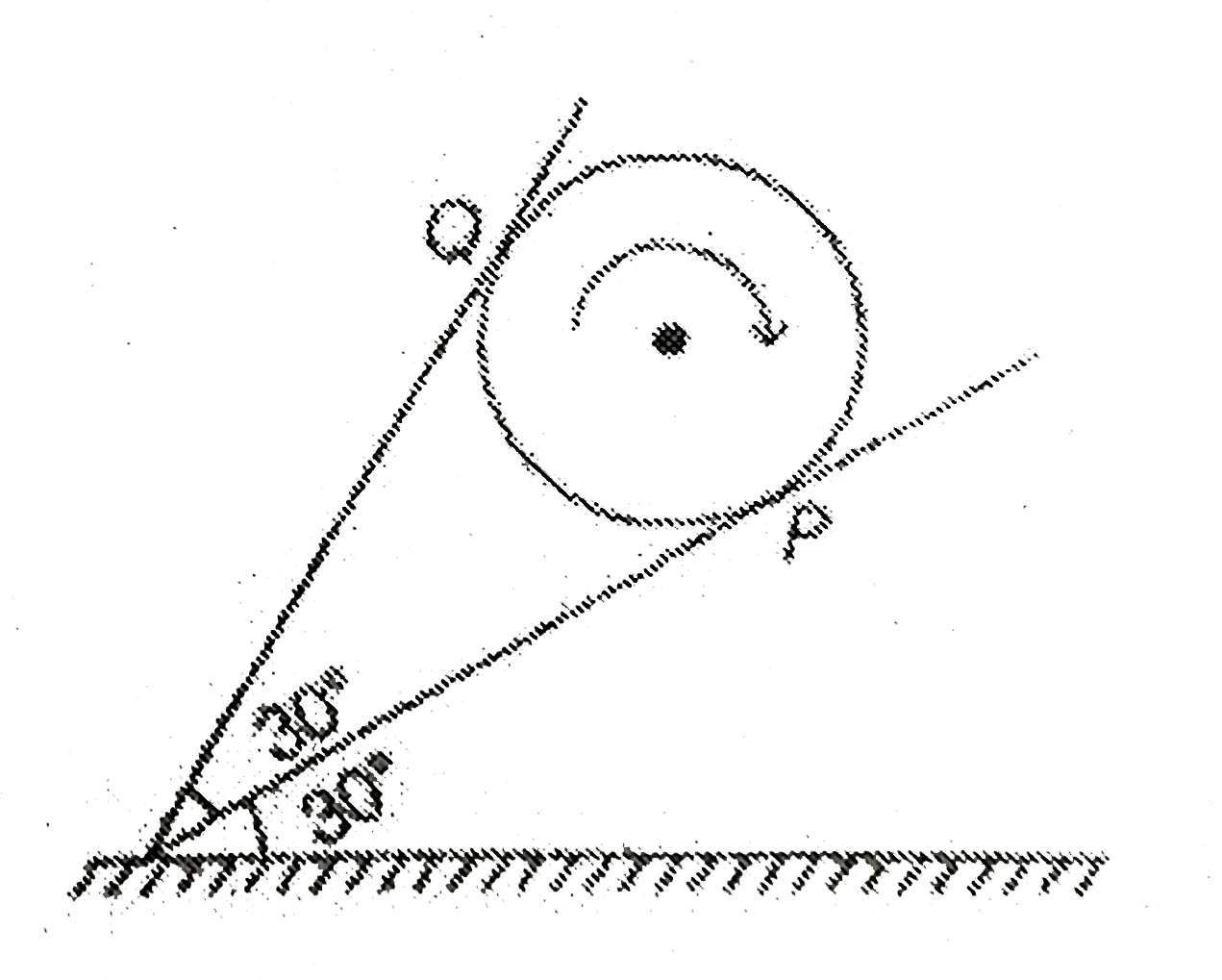
A sphere is rotating between two rough inclined walls as shown in figure. Coefficient of friction between each wall and the sphere is 1/3. If f1andf2 be the frictional forces at P and Q. Then f1f2 is
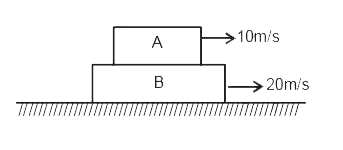
View SolutionAll surfaces as shown in the figure are rough. Draw the friction force on A & B
View Solution
Knowledge Check
A sphere is placed rotating with its centre initially at rest in a corner as shown in Figs.(a) and (b). Coefficient of friction between all surfaces and the sphere is 1/3. Find the ratio of the friction forces fa/fb by ground in situations (a) and (b).
 A1B910C109Dnone of these
A1B910C109Dnone of theseA sphere is rotating between two rough inclined walls as shoen in fiogure. Cofficent of friction between each wall and the sphere is 13. If f1 and f2 be the ffriction forces at PO and Q. Then f1f2 is
 A4√3+1B1√3+2C12+√3D1+2√3
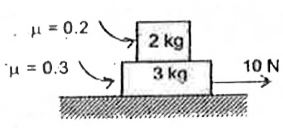
A4√3+1B1√3+2C12+√3D1+2√3Consider the situation as shown in figure, frictional force acting between surface and 3 kg block is
 A15 NB10 NC0 ND4 N
A15 NB10 NC0 ND4 N
Determine the force P required to impend the motion of the block B shown in figure. Take coefficient of friction = 0.3 for all surfaces in contact.
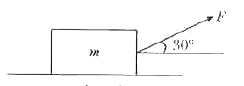
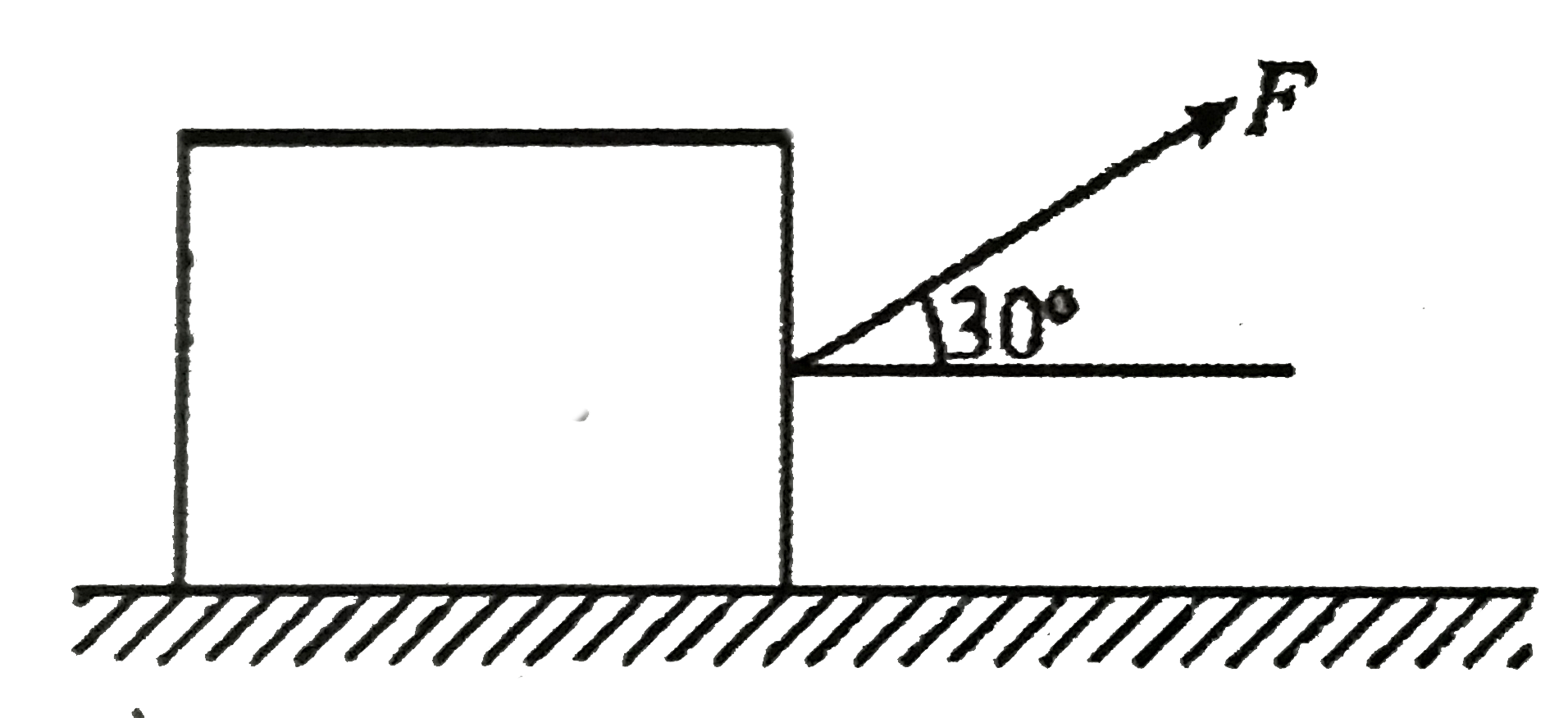
View SolutionA mass m is at rest under the action of a force F. as shown in the figure, on a horizontal surface. The coefficient of friction between mass and surface is u. Then, find the correct choice(s)
View SolutionIf the coefficient of friction between all surface figure is 0.4 then find the minimum force F to have equilibrium of the system.
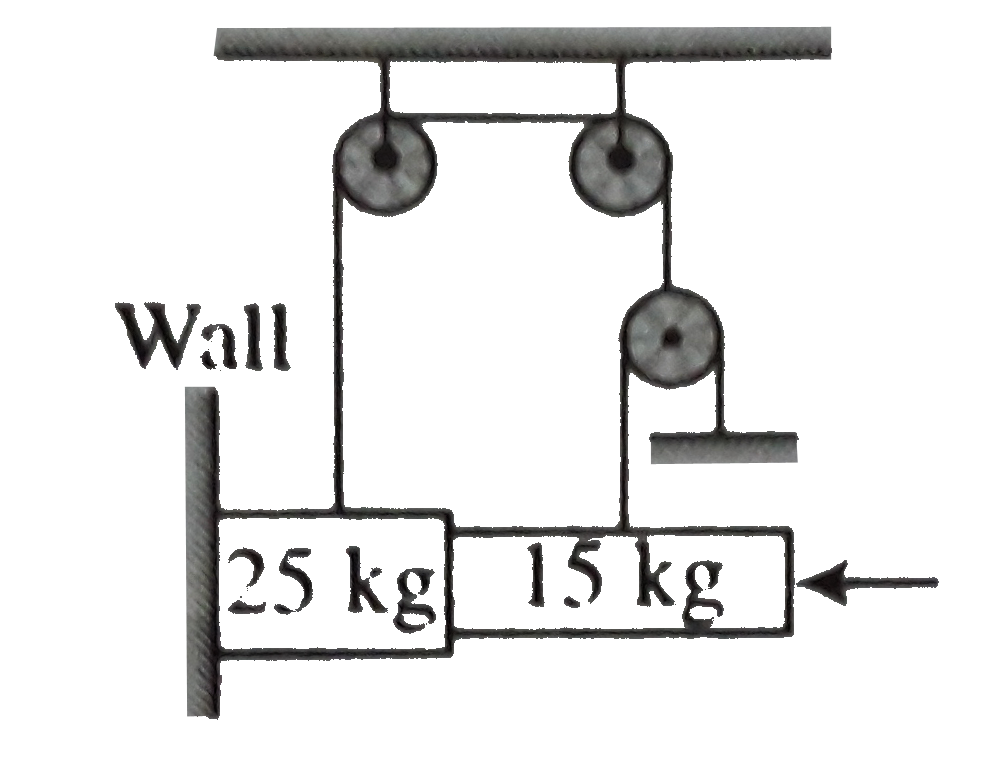
View SolutionA mass m rests under the action of a force F as shown in the figure-2.158 on a horizontal surface. The coefficient of friction between the mass and the surface is μ. The force of friction between the mass and the surface is :
View SolutionIn the figure shown, the force of friction between A and B will be :
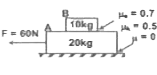
View Solution