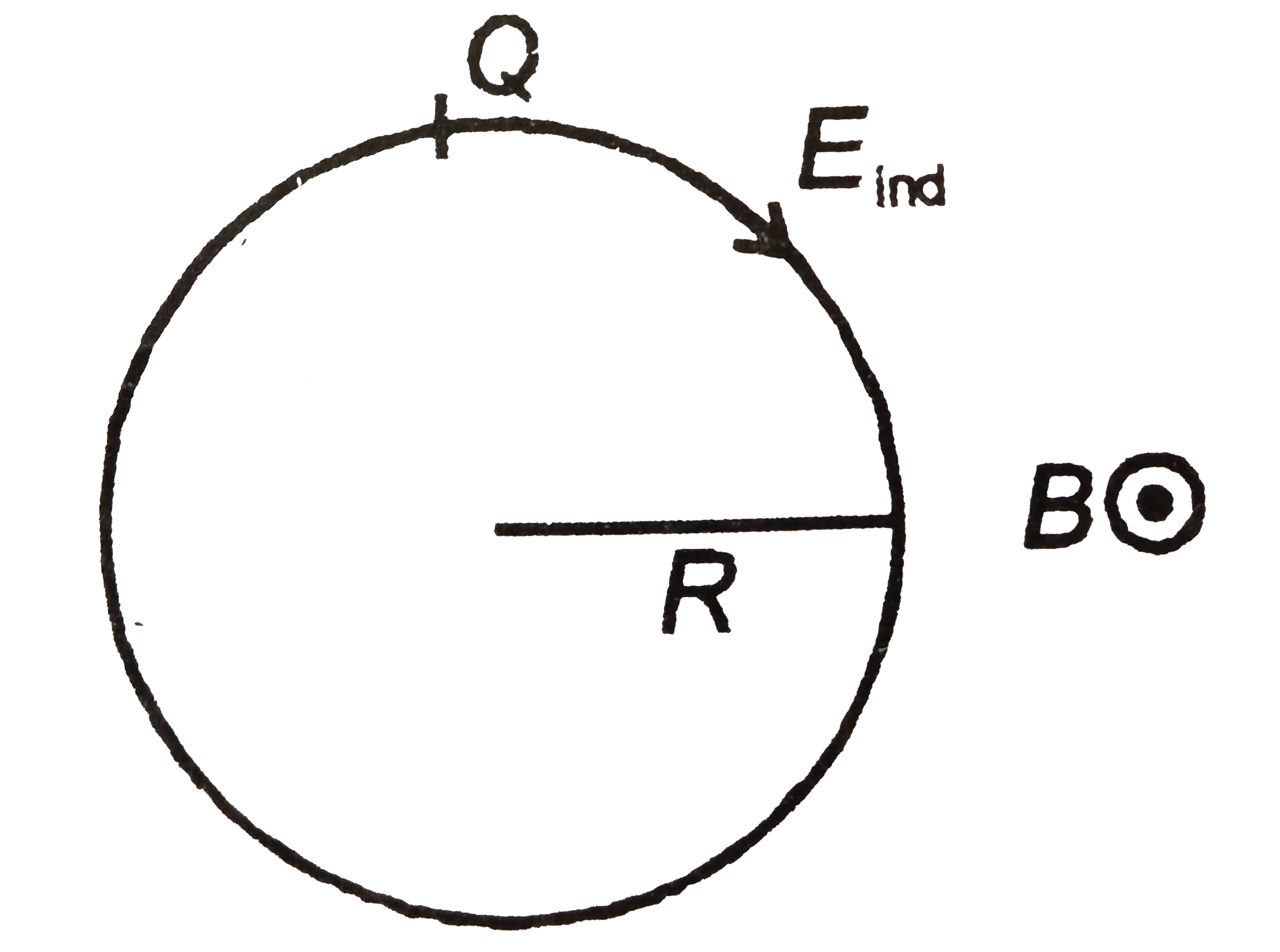
A point charges Q is moving in a circular orbit of radius R in the x-y plane with an angular velocity ω. This can be considered as equivalent to a loop carrying a steady current Qω2π. S uniform magnetic field along the positive z-axis is now switched on, which increases at a constant rate from 0 to B in one second. Assume that the radius of hte orbit remains constant. The application of hte magnetic field induces an emf in the orbit. The induced emf is defined as the work done by an induced electric field in moving a unit positive charge around a closed loop. It si known that, for an orbiting charge, the magnetic dipole moment is proportional to the angular momentum with a porportionality constant λ.
The charge in the magnetic dipole moment associated with the orbit. at the end of the time interval of hte magnetic field charge, is
−gBQR2
gBQR22
gBQR22
gBQR2
The correct Answer is:B
B along (+zaxis)
M=gL=gmwR2
(where m=mass of charged particle)R
M=g.mwR2
M will change due to change in ω Change in ω is
given by|dw|=Bq2m
Change in M=g.mBQ2m.R2=−gBQR22
Negative sign shows change is opposite to direction of B
 .
.
Similar Questions
A particle of charge q and mass m moves in a circular orbit or radius r with angular speed ω. The ratio of the magnitude of its magnetic moment to that of its angular momentum depends on
View SolutionThe magnetic momenrt of a circular orbit of radius 'r' carrying a charge 'q' and rotating with velocity v is given by .
View Solution
Knowledge Check
A point charge Q is moving in a circular orbit of radius R in the x-y plane with an angular velocity ω. This can be considered as equivalent to a loop carrying a steady current Qω/2π. A uniform magnetic field along the positive z-axis is now switched on, which increases at a constant rate from 0 to B in one second. Assume that the radius of the orbit remains constant. The application of the magnetic field induces an emf in the orbit. The induced emf is defined as the work done by an induced electric field in moving a unit positive charge around a closed loop. It is known that , for an orbiting charge, the magnetic dipole moment is proportional to the angular momentum with a proportionally constant γ
The change in the magnetic dipole moment associated with the orbit, at the end of the time interval of the magnetic field change is :A−γBQR2B−γBQR22CγBQR22DγBQR2A point charges Q is moving in a circular orbit of radius R in the x-y plane with an angular velocity ω. This can be considered as equivalent to a loop carrying a steady current Qω2π. S uniform magnetic field along the positive z-axis is now switched on, which increases at a constant rate from 0 to B in one second. Assume that the radius of hte orbit remains constant. The application of hte magnetic field induces an emf in the orbit. The induced emf is defined as the work done by an induced electric field in moving a unit positive charge around a closed loop. It si known that, for an orbiting charge, the magnetic dipole moment is proportional to the angular momentum with a porportionality constant λ.
The charge in the magnetic dipole moment associated with the orbit. at the end of the time interval of hte magnetic field charge, isABR4BBR2CBRD2BRA particle moving along a circule path of radius 'r' with uniform angular velocity ω. Its angular acceleration is
Arω2Br/ωCzeroDω/r
An electron revolves in a circular orbit of radius r with angular speed ω . The magnetic field at the centre of electron orbit is
View SolutionA particle of charge q and mass m moves in a circular orbit of radius r with angular speed ω. The ratio of the magnitude of its magnetic moment to that of its angular momentum depends on
View SolutionA particle of charge q and mass m moves in a circular orbit of radius r with angular speed ω. The ratio of the magnitude of its magnetic moment to that of its angular momentum depends on
View SolutionAn electron of chargee, revolves round in an orbit of radius r with a uniform angular velocity ω. The magnetic dipole moment of the electron in the orbit is
View SolutionA particle of the charged q and massm moves in a circular orbit of radius r with angular speed ω . The ratio of the magnitude of its magnetic moment to that of its angular momentum depends on
View Solution
