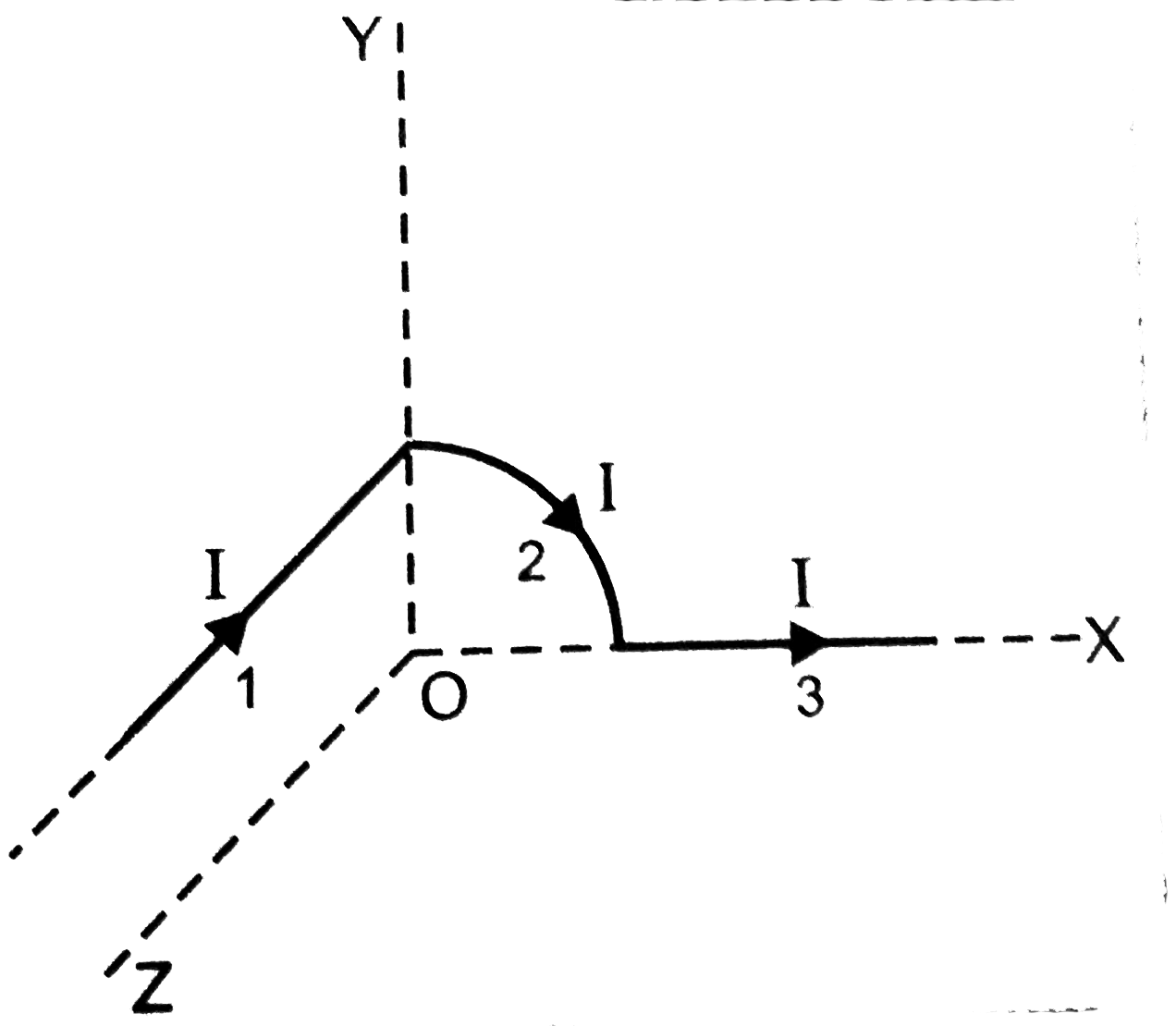
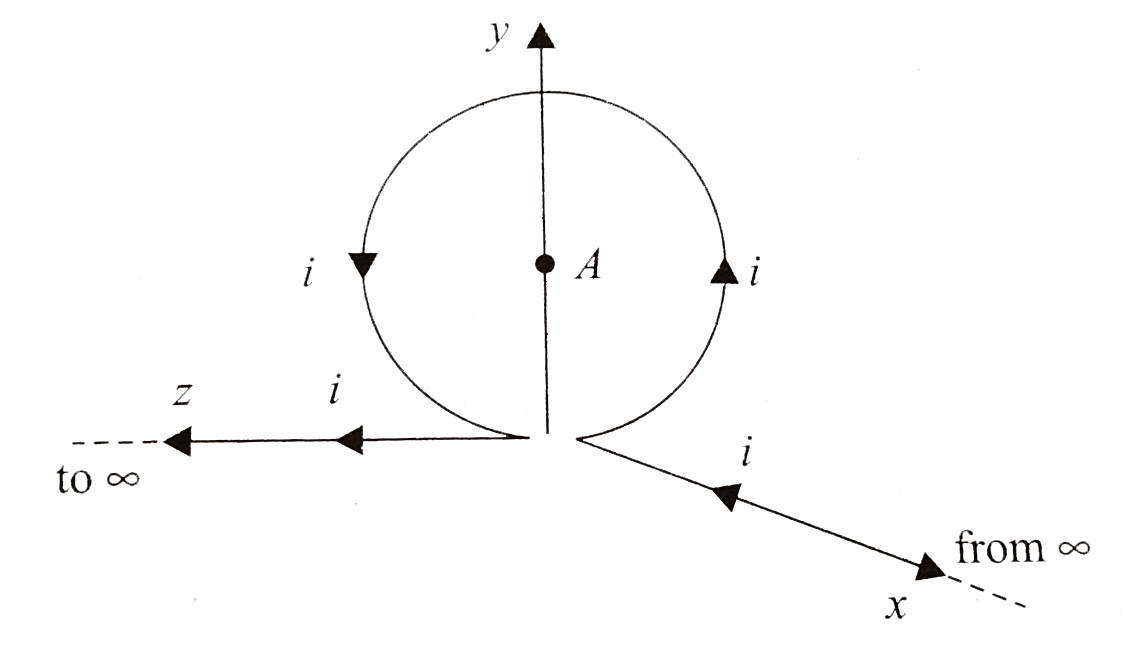
Find the magnitude of the magnetic induction B of a magnetic field generated by a system of thin conductors along which a current I is flowing at a point A (O, R, O), that is the centre of a circular conductor of radius R. The ring is in yz plane.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: $B = \frac{μ_{0} i}{4 π R} \sqrt{2 (2 π^{2} - 2 π + 1)}$

${\to B}_{1} = \frac{μ_{0}}{4 π} \frac{I}{R} (- ˆ k) {\to B}_{2} = \frac{μ_{0}}{4 π} \frac{2 π I}{R} (ˆ i)$
$- \to B_{3} = \frac{μ_{0}}{4 π} \frac{I}{R} (- ˆ i)$
$\to B = {\to B}_{1} = {\to B}_{2} {\to B}_{3}$
$= \frac{μ_{0}}{4 π} \frac{I}{R} (2 π - 1) ˆ i + \frac{μ_{0}}{4 π} \frac{I}{R} (ˆ k)$
$B = \frac{μ_{0}}{4 π} \frac{I}{R} \sqrt{{(2 π - 1)}^{2} + 1}$
$= \frac{μ_{0}}{4 π} \frac{I}{R} \sqrt{2 (2 π^{2} - 2 π + 1)}$
.

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Similar Questions

Knowledge Check

Question 1 - Select One

A conducting wire carrying a current I is bent into the shape as shown. The net magnetic field at the centre O of the circular arc of radius R
A $\frac{μ_{0} l}{2 R} (\frac{1 + 3 π}{π})$
B $\frac{μ_{0} l}{4 R} (\frac{1 + 3 π}{π})$
C $\frac{μ_{0} l}{8 R} (\frac{1 + 3 π}{π})$
D $\frac{μ_{0} l}{8 R} (\frac{2 + 3 π}{π})$
Question 2 - Select One
Magnetic field at some point, generated by a current bearing conductor, is proportional to
Aresistance of the conductor
Bthickness of the conductor
Ccurrent passing through conductor
Ddistance from the conductor
Question 3 - Select One
The magnetic induction at $O$ due to a current in conductor shaped as shown in figure

A $\frac{μ_{0} i}{4 π} [\frac{3 π}{2 a} + \frac{\sqrt{2}}{b}]$
B $\frac{μ_{0} i}{4 π} [\frac{3 π}{4 a} - \frac{\sqrt{2}}{b}]$
C $\frac{μ_{0} i}{4 π} [\frac{3 π}{4 a} + \frac{1}{\sqrt{2} b}]$
D $\frac{μ_{0} i}{4 π} [\frac{1}{a} + \frac{1}{b}]$

Recommended Questions

Home
Ask Doubt
Profile