Three concentric conducting spherical shells of radii R,2R and 3R carry charges Q,−2Q and 3Q, respectively.
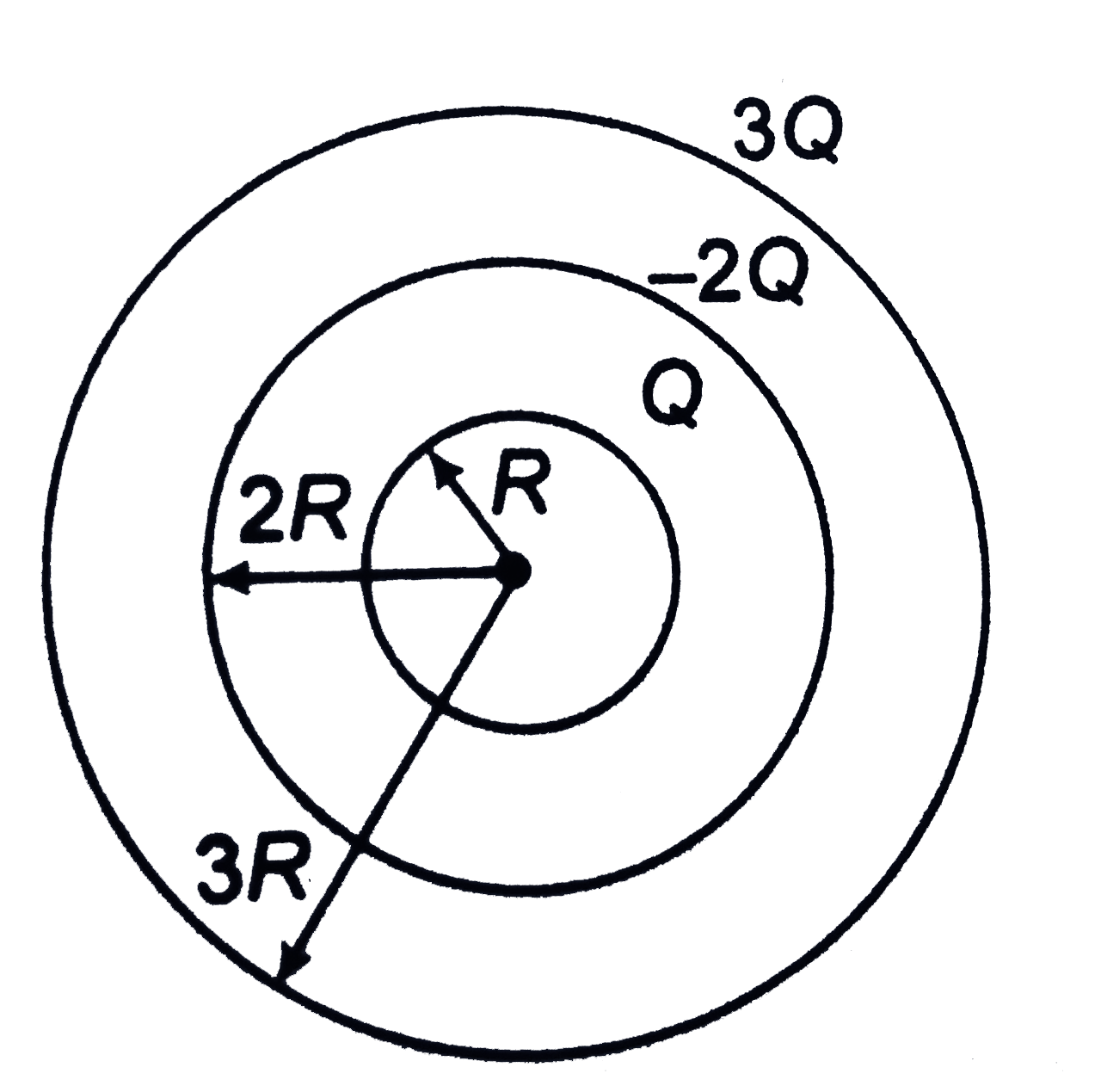
a. Find the electric potential at r=R and r=3R where r is the radial distance from the centre. ltbr> b. Compute the electric field at r=52R
c.computethe→tale≤ctrostatice≠rgys→red∈thesystem.<br>The∈≠rshellisnowco∩ected→theexternalo≠byaconduct∈gwire,passingthroughaverysmallho≤∈them≤shell.<br>Computethechar≥sonthespheresofradiiRand3R.<br>e.Computethee≤ctricfieldatr=5/2R.
The correct Answer is:(a) Q4πɛ0R,Q6πɛ0R ; (b) −Q25πɛ0R2ˆr,
(c ) Q24πɛ0Rˆr,
(d) Q+2Q112πɛ0R,Q1=Q2.Q2=7Q2
(e ) −3Q50ɛ0R2ˆr.
(a) Potential of inner shpere
VR= Potential due to won charge + ptential due to middle sphere + potential due to oute shere .
VR=KQR+K(−2Q)2R+K(3Q)3R

VR=KQR
Vr=14π∈0QR
V3R= Potential due to ininer shpere + potential due to middle sheere + pontential due to own charge .
V3R=KQ2R+K(−2Q)3R+K(3Q)3R=2KQ3R=Q6π∈0R
(b) From gauss theorem
∮→Es→S=∑q∈0⇒e.4π(52R)2=(Q−2Q)∈0
→E=−Q25π∈0r2→r .
(c) Chagrge distaribution will be as shown in so combination will acts as two sphereical capacitor of capacity →C and cexC2
C1=4π∈0R×2R(2R−R)

C1=8π∈0R ....(1)
C2=4π∈0(2R×3R)(3R−2R)=24π∈0R ...(2)
U=U1+U2
U=−Q22C1+((3Q))22C2 ....(3) Fpr, (1) , (2) and (3)
U=Q24π∈2R
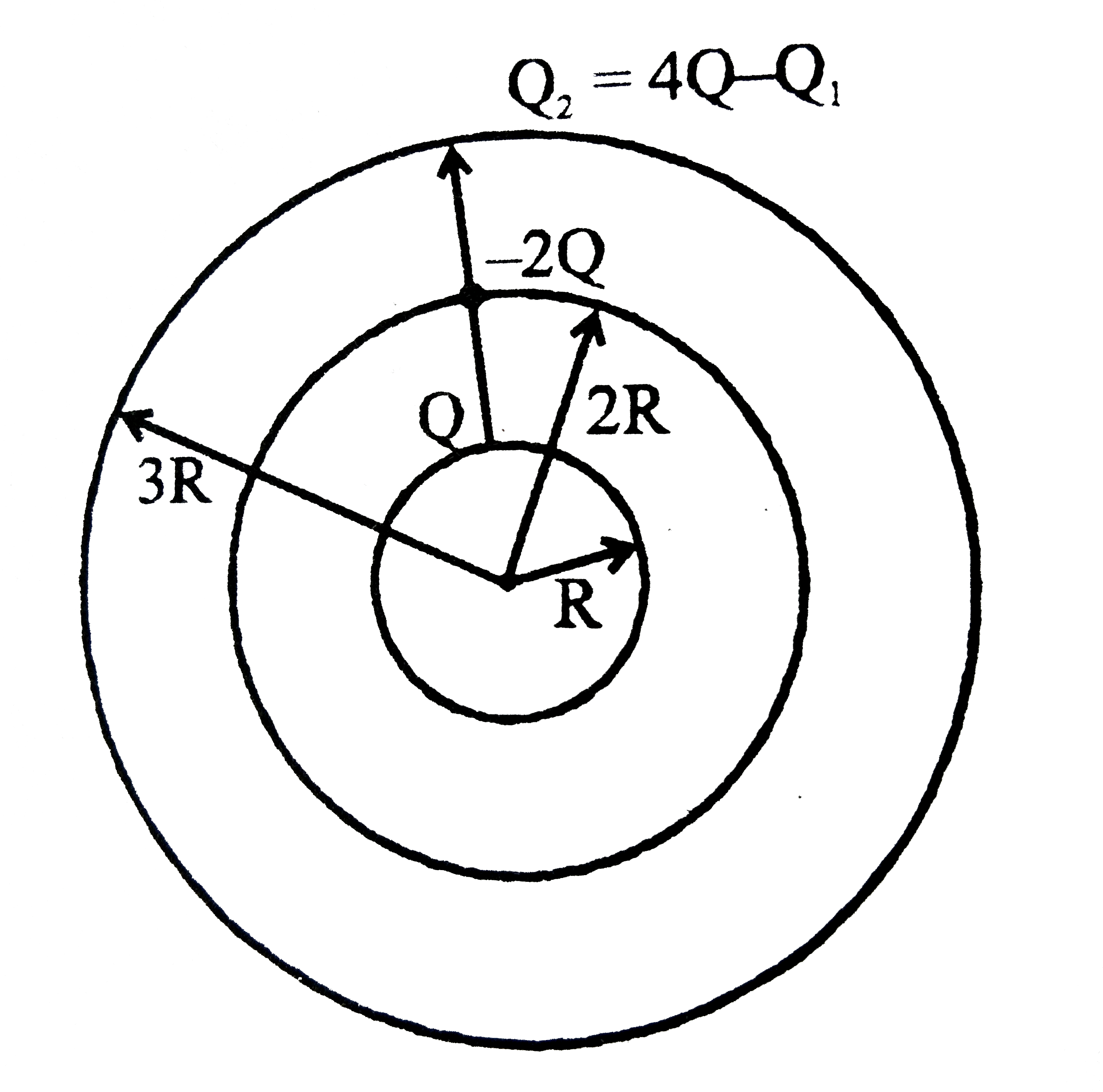
(d) Since inner sphere and out shere are connected by a sonducing wire so they have equal potential
VR=V3R
KQ1R+K(−∘Q)2R+K(4Q−Q1)3R=kQ13R+K(−02Q)2R
+K(4Q−Q)3R
Q1−Q=Q13−2Q3
2Q13=Q3⇒Q1=Q2
and Q2=4Q−Q1=4Q−Q2
Q2=7Q2
E≤ctricfieldatr = 5/2 R.<br>int _s vec Ed vec A = (sum q)/(in_0) rArr E. 4 pi (5/2 R)^2 = (Q_1-2Q)/(in_0)<br>E = q/(25 pi R^2 in_0) xx (Q/2- 2 Q) rArr E = (3 Q)/(50 pi in_0 R^2).
Similar Questions
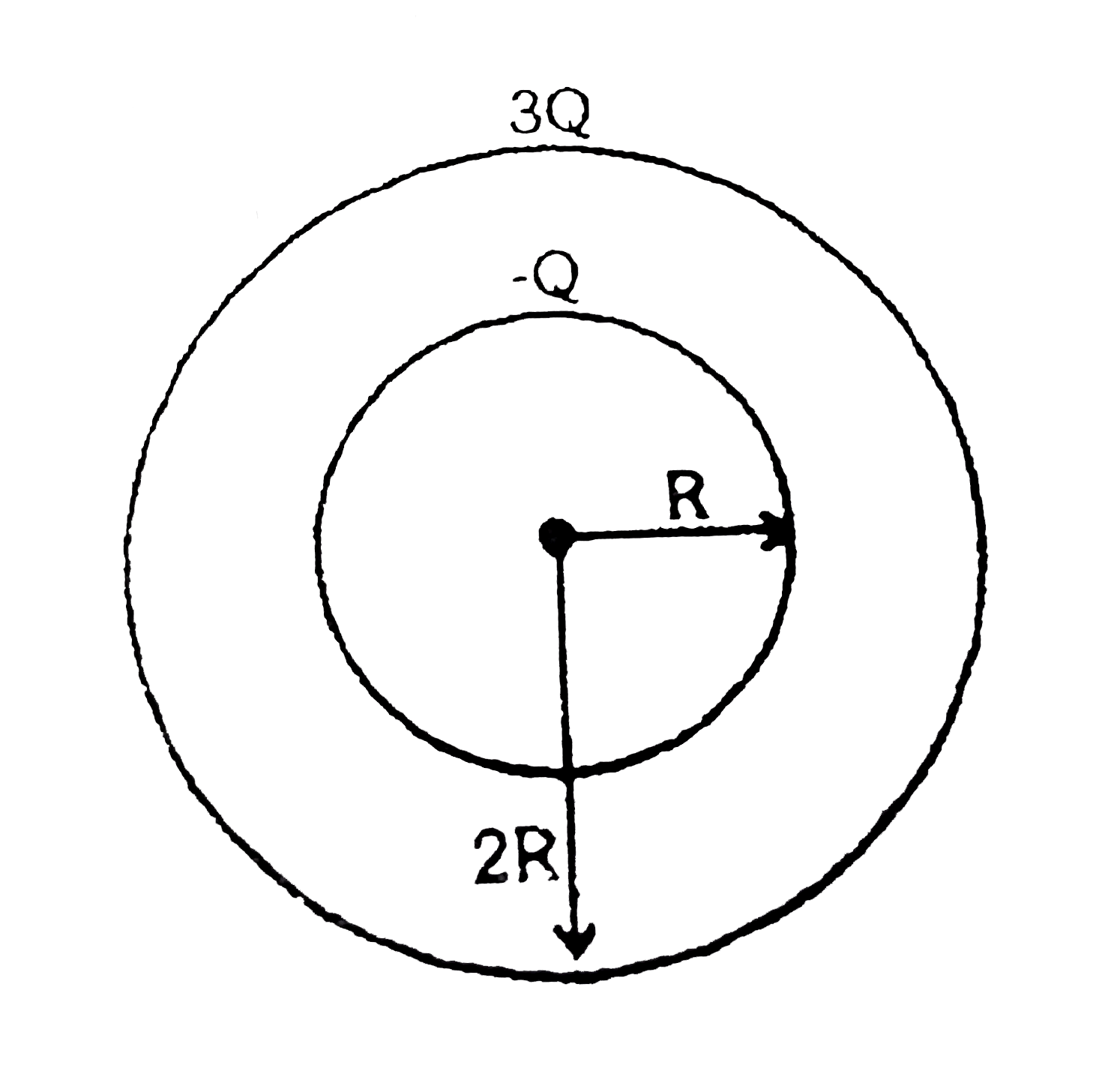
Two conducting hollow spherical shells of radius R and 2R carry charges -Q and 3Q respectively. How much charge will flow into the earth if inner shell is grounded?
View SolutionTwo concentric conducting spheres of radii R and 3R carrying charges Q and 2Q respectively.If the charge on inner sphere is doubled.The potential difference between inner and outer spheres will..?
View Solution
Knowledge Check
Three concentric conducting spherical shells have radii r, 2r, and 3r and charges q1,q2andq3, respectively. Innermost and outermost shells are earthed as shown in figure. The charges shown are after earthing. Select the correct alternative.
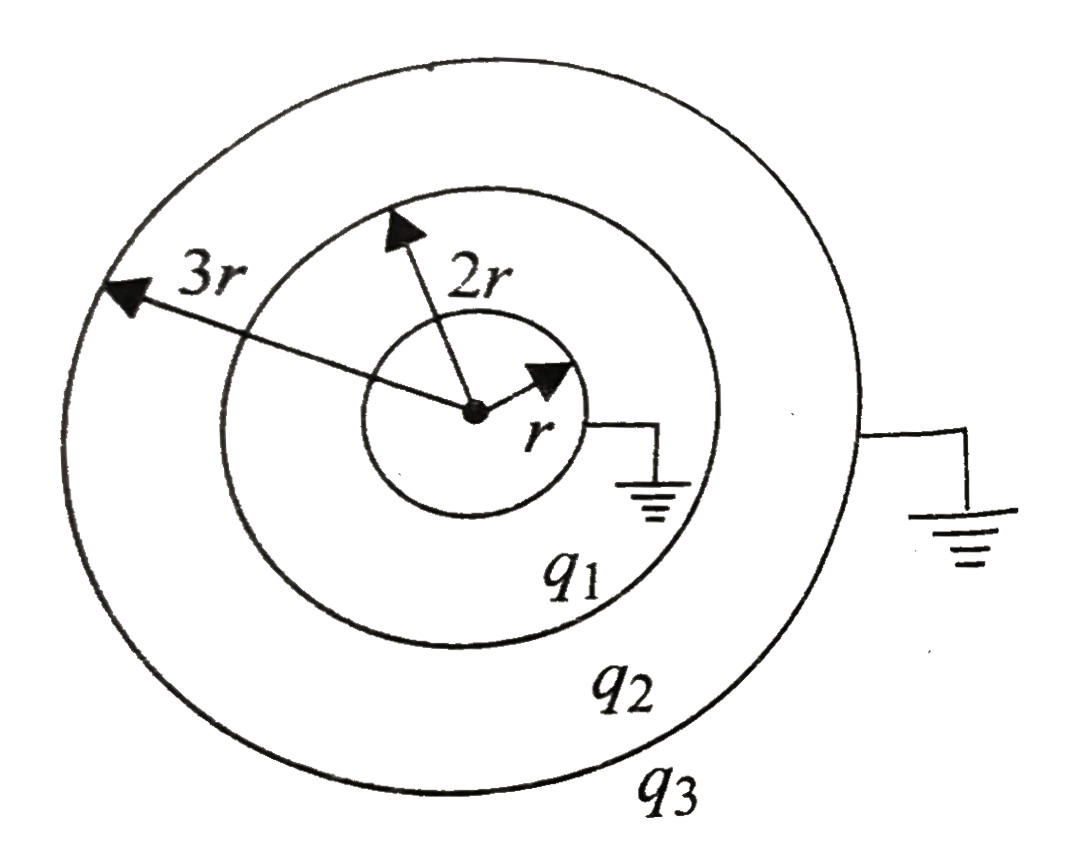 Aq1+q3=−q2Bq1=−q2Cq3q2=−13Dnone of these
Aq1+q3=−q2Bq1=−q2Cq3q2=−13Dnone of theseTwo concentric spherical conducting shells of radii r and R (r<R) carry charges q and Q respectively . The two shells are now connected by a conducting wire. The final charge on the inner shell is
AzeroB(Q+q)rRC(Q−q)rRDqrRA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
A(a) 2Q4πε0RB(b) 2Q4πε0R−2q4πε0RC(c) 2Q4πε0R+q4πε0RD(d) (q+Q)24πε0R
Two concentric spherical conducting shells of radii R and 2R carry charges Q and 2Qrespectively.Change in electric potential on the outer shell when both are connected by a conducting wire is (k=14πɛ0)
View SolutionTwo concentric spherical conducting shells of radii R and 2R carry charges Q and 2Qrespectively.Change in electric potential on the outer shell when both are connected by a conducting wire is (k=14πɛ0)
View SolutionTwo isolated concentric conducting spherical shells have radii R and 2R and uniform charges q and 2q respectively.If V1 and V2 are potentials at points located at distances 3R and R2 respectively from the centre of shells.Then the ratio of (V2V1) will be
View SolutionTwo concentric spherical shells of radii R and 2R have charges Q and 2Q as shown in figure

If we draw a graph between potential V and distance r from the centre, the graph will be like
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
View Solution