A non conduction ring of raducs R Has uniformly distributed positive charge Q.A small part of the ring of length d, is removed (d lt lt R) . The electric field at the centre of the ring now be .
directed towards the gop,inversl propprtional to R3
directed towards the gap, inversly proportional to R∘
directed away from the gap, inversly
directed away from the gap, inversly proportional to R∘
The correct Answer is:A
Field due to charged arc at the centre of arc
E=2KλR.Sin(θ)2
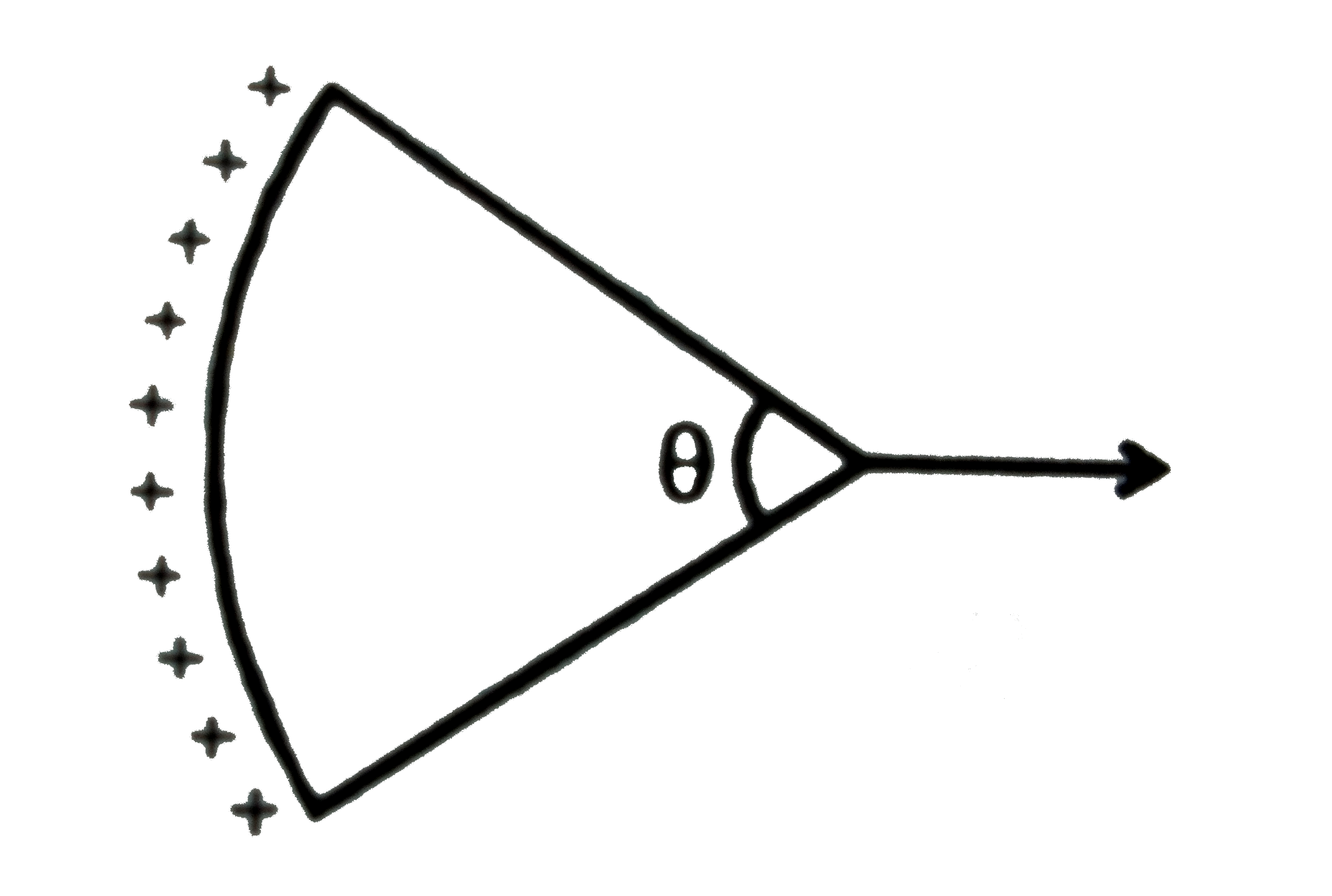
Field due to remaining part at the centre will be equal and opposite to the field due to removed part .
(##NGPHYC01E01008_S02.png width=80%><br>E = (2 k lambda)/R . sin (theta)/2Forsmall theta<br> E = (K lambda) /R theta = K/R . Q/(2 pi R) . d/R<br> E = (KQd)/(2piR^3).
Similar Questions
A non conducting ring of radius R is charged as shown in figure : Figure

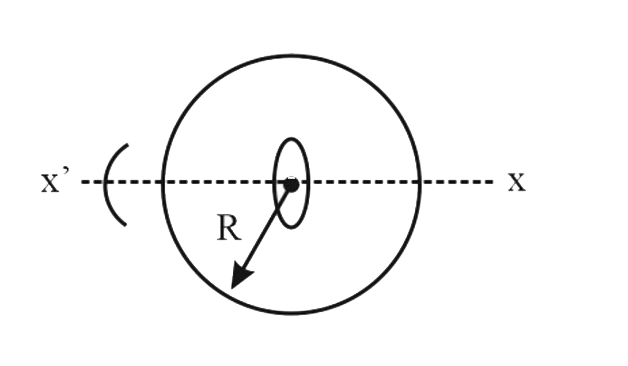
View SolutionA non-conducting ring of radius R having uniformly distributed charge Q starts rotating about x-x' axis passing through diameter with an angular acceleration α, as shown in the figure. Another small conducting ring having radius a(a<<R) is kept fixed at the centre of bigger ring is such a way that axis xx' is passing through its centre and perpendicular to its plane. If the resistance of small ring is r=1Ω , find the induced current in it in ampere.
(Given q=16×102μ0C,R=1m,a=0.1m,α=8rad s−2
View Solution
Knowledge Check
Charges q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
Aq2π2ε0R2Bq4π2ε0R2Cq4πε0R2Dq2πε0R2A circular ring of radius r carries a charge Q uniformly spread on it. A small length dl is cut off. Find the electric field at the centre due to the remaining wire.
AQ4πε0r2BQdl4π2ε0r3CQdl8π2ε0r2DQdl8π2ε0r3A thin conducting ring of radius R is given a charge +Q, Fig. The electric field at the center O of the ring due to the charge on the part AKB of the ring is E. The electric field at the center due to the charge on part ACDB of the ring is
 A3E along KOBE along OKCE along KOD3E along OK
A3E along KOBE along OKCE along KOD3E along OK
A ring of radius R carries a charge +Q, which is uniformally distributed on the surface of the ring. The electrostatic force acting on a charge +q situated at the centre of ring is zero.
View SolutionA non-conducting ring of radius R having uniformly distributed charge Q starts rotating about x-x' axis passing through diameter with an angular acceleration α, as shown in the figure. Another small conducting ring having radius a(a<<R) is kept fixed at the centre of bigger ring is such a way that axis xx' is passing through its centre and perpendicular to its plane. If the resistance of small ring is r=1Ω , find the induced current in it in ampere.
(Given q=16×102μ0C,R=1m,a=0.1m,α=8rad s−2

View SolutionA ring of radius R with a uniformly distributed charge q as shown in figure. A charge q0, is now placed at the centre of the ring. Find the increment in-the tension in ring.
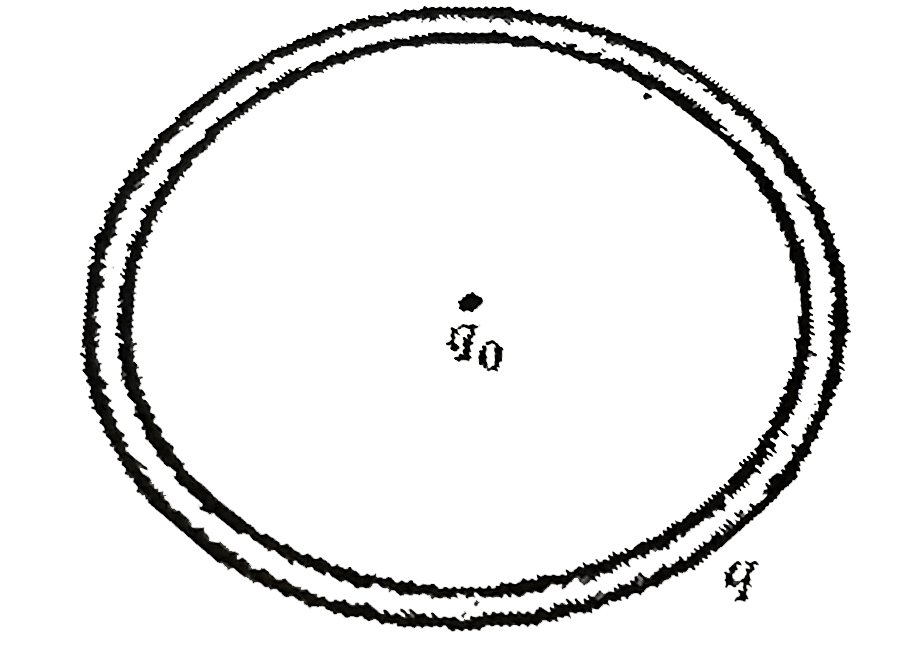
View SolutionA ring of radius R is with a uniformly distributed charge Q on it .A charge q is now placed in the centre of the ring .Find the increment in tension in the ring.
View Solution
A small part of dl length is removed from a ring having charge per unit length λ. Find electric field at centre due to remaining ring.
View Solution