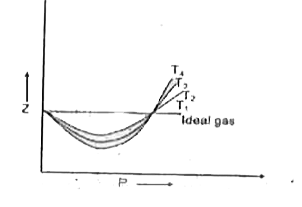
Assertion: Compressibility factor for hydrogen varies with pressure with positive slope at all pressures.
Reason: Event at low pressures, repulsive forces dominate hydrogen gas.
A
If both assertion and reason are true and the reason is the correct explanation of the assertion.
B
If both assertion and reason are true but reason is not the correct explanation of the assertion.
C
If assertion is true but reason is false.
D
If assertion is false but reason is true.
Video Solution
Text Solution
The correct Answer is:A
In case of H2, compressibility factor increases with the pressure. At 273K,Z>1 which shows that it is difficult to compress the gas as compared to ideal gas. In this case repulsive forces dominate.
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Assertion: Compressibility factor for hydrogen varies with pressure with positive slope at all pressures.
Reason: Even at low pressures, repulsive forces dominate hydrogen gas.AIf both assertion and reason are true and the reason is the correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of the assertion.CIf assertion is true but reason is false.DIf assertion is false but reason is true. - Question 2 - Select One
Assertion: Compressibility factor for hydrogen varies with pressure with positive slope at all pressures.
Reason: Even at low pressures, repulsive forces dominate hydrogen gas.AIf both assertion and reason are true and the reason is correct explanation of the assertion.BIf both assertion and reason are true but reason is not the correct explanation of the assertion.CIf assertion is true but reason is false.DIf the assertion and reason both are false - Question 3 - Select One
STATEMENT-1 : Compressibility factor for hydrogen varies with pressure with positive slope at all pressures at 0∘C.
STATEMENT-2 : Even at low pressures, repulsive forces dominate in hydrogen gas at 0∘C.AIf both the statement are TRUE and STATEMENT-2 is the correct explanation of STATEMENT-1BIf both the statement are TRUE but STATEMENT-2 is NOT the correct explanation of STATEMENT-1CIf STATEMENT-1 is TRUE and STATEMENT-2 is FALSEDIf STATEMENT-1 is FALSE and STATEMENT-2 is TRUE
At moderate pressure, compressibility factor of a gas
View SolutionThe compressibility factor (Z) of real gas is usually less than 1 at low temperature and low pressure because
View SolutionCompressibility factor (Z) is plotted against pressure at different temperature for same gas
View SolutionThe rise is compressibility factor (Z) with increasing pressure of a gas is due to
View SolutionFor which of the following gas compressibility factor value increases with increase of pressure even at low temperatures
View Solution